Discrete and continuous random variables | Probability and Statistics | Khan Academy
Summary
TLDRThis video explains the difference between discrete and continuous random variables. Discrete random variables take on distinct, countable values, such as the outcome of a coin flip or the number of ants born. Continuous random variables, on the other hand, can take any value within a range, such as the mass of an animal or the exact time in a race. Through relatable examples and clear distinctions, the video highlights the importance of understanding whether a random variable is discrete or continuous for statistical analysis.
Takeaways
- 😀 Discrete random variables can take on distinct or separate values, which are countable.
- 😀 Continuous random variables can take on any value within a specified range or interval, and these values are uncountable.
- 😀 A discrete random variable's possible values can be listed or counted, even if infinite.
- 😀 The coin toss example (1 for heads, 0 for tails) illustrates a discrete random variable.
- 😀 A continuous random variable, like the mass of an animal, can take on infinite values in a range, making it uncountable.
- 😀 Discrete random variables are often finite but can also be countably infinite, such as the number of ants born in the universe.
- 😀 Continuous random variables, like the exact time of a race, can have infinite precision, with no way to list or count all possible values.
- 😀 A discrete random variable's values are countable, while continuous random variables' values cannot be listed exhaustively.
- 😀 The year a student was born is a discrete random variable because you can count specific years (e.g., 1992, 1993, etc.).
- 😀 The exact mass of an animal in a zoo is a continuous random variable because it can take on any value within a range, including infinite decimals.
Q & A
What is the main distinction between discrete and continuous random variables?
-The main distinction is that discrete random variables take on distinct, separate values that can be counted, while continuous random variables can take on any value within a given range or interval, and the possible values are uncountably infinite.
What is an example of a discrete random variable from the script?
-An example of a discrete random variable from the script is the result of a fair coin flip. It can only take on the values 0 (tails) or 1 (heads), making it a discrete variable.
Why is the mass of a random animal at a zoo considered a continuous random variable?
-The mass of a random animal is considered a continuous random variable because it can take on any value within a range (e.g., between 0 kg and 5,000 kg), and there are an infinite number of possible mass values in between any two given values.
Can a discrete random variable have an infinite number of possible values? Give an example.
-Yes, a discrete random variable can have an infinite number of possible values, as long as those values can be counted. An example is the year of birth of a random student, where the possible years (1990, 1991, 1992, ...) can go on infinitely but are still countable.
What is the role of rounding in distinguishing between discrete and continuous random variables?
-Rounding can make a continuous random variable appear discrete. For example, the exact winning time in the 100-meter dash is a continuous variable, but if it is rounded to the nearest hundredth of a second, it becomes a discrete variable because the rounded times can be listed (e.g., 9.56, 9.57, 9.58).
Why is the number of ants born tomorrow in the universe a discrete random variable?
-The number of ants born tomorrow is a discrete random variable because you can count the number of ants. Whether it is 1, 2, or a quadrillion, these values are distinct and countable.
What makes continuous random variables different from discrete ones in terms of the values they can take?
-Continuous random variables can take on any value within a given range and are not limited to distinct or countable values. For example, the mass of an animal at the zoo can be any real number within a range, unlike discrete variables, which can only take on specific, countable values.
In the example of the coin flip, why is the random variable considered discrete?
-The random variable in the coin flip example is considered discrete because it can only take two distinct values: 0 (tails) or 1 (heads), and these values are countable and distinct.
Can continuous random variables be approximated by discrete ones in some cases?
-Yes, continuous random variables can be approximated by discrete ones when the values are rounded or truncated. For instance, the exact time for the 100-meter dash can be continuous, but when rounded to the nearest hundredth, it behaves as a discrete random variable.
What is the significance of being able to 'count' the values of a random variable?
-Being able to count the values of a random variable indicates that the variable is discrete. If the values can be listed or counted, it is a discrete random variable; if they cannot be listed because there are infinitely many possibilities in between any two values, the variable is continuous.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

Distribusi Binomial • Part 1: Variabel Acak
Random Variables - Grade 11 (Statistics and Probability) @MathTeacherGon

Distribusi Normal • Part 1: Distribusi Peluang Variabel Acak Kontinu

VARIABEL ACAK DISKRIT: Pengertian dan Distribusi Peluang-nya!

Konsep Variabel Acak Diskrit dan Kontinu
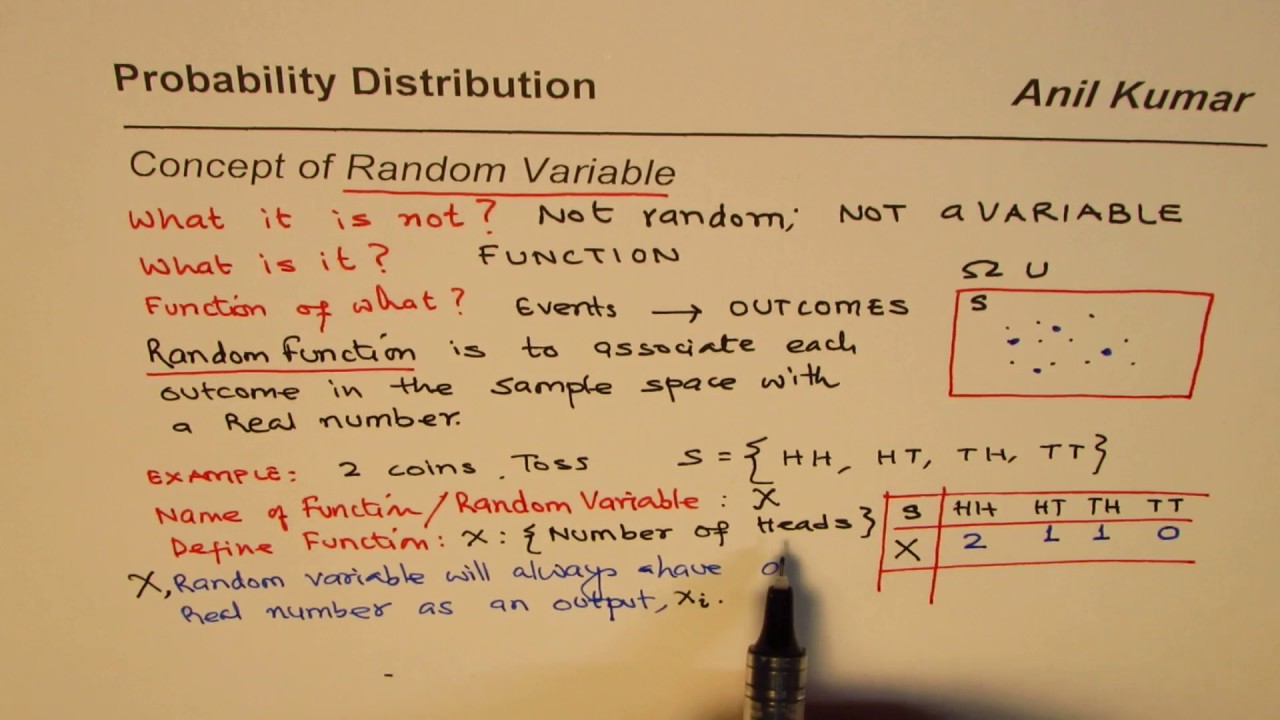
Introduction to Random Variables Probability Distribution
5.0 / 5 (0 votes)
