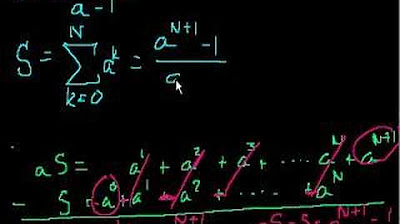Geometry of sums of powers of 1/5
Summary
TLDRThe video explains the geometric representation of the infinite sum of powers of one-fifth using a square. By dividing the square into five equal parts—four triangles and a smaller inner square—each piece represents one-fifth of the area. This process is repeated indefinitely within the inner square, illustrating the series 1/5 + 1/5² + 1/5³, and so on. When the shape is rotated four times, it fills the entire square, leading to the conclusion that the sum of the infinite series equals one-fourth.
Takeaways
- 🟢 The video begins by exploring the infinite sum of the powers of one-fifth.
- 🟩 A 1x1 square is used as the base to visualize the process.
- 🔺 Connecting the vertices to the midpoints divides the square into five equal parts.
- 📐 Four triangles and one inner square each represent one-fifth of the area.
- ♻️ The process can be repeated infinitely with the inner square, generating smaller and smaller areas.
- 🔄 Each iteration takes one-fifth of the previous inner square, spiraling down indefinitely.
- ➕ The shaded area represents a series: 1/5 + (1/5)^2 + (1/5)^3 and so on.
- 🔄 Rotating the shape three more times fills in the entire square with these areas.
- ✅ The sum of this infinite series of powers of 1/5 equals one-fourth.
- 📊 The geometric visualization demonstrates how the infinite sum converges to a finite value of 1/4.
Q & A
What does the initial one-by-one square represent in the context of the video?
-The one-by-one square represents the area that is progressively divided into smaller pieces to illustrate the sum of the powers of one-fifth.
How is the square divided initially?
-The square is divided into five equal pieces by connecting its vertices to the midpoints, resulting in four triangles around the outside and one inner square, each consisting of one-fifth of the area.
What is the area of the triangle formed by connecting the vertices to the midpoints?
-Each of the four triangles formed around the inner square has an area of one-fifth of the total square.
What happens to the inner square in the process described?
-The process is repeated on the inner square, dividing it into smaller sections, each time reducing its area by a factor of one-fifth.
What mathematical concept is illustrated by this division process?
-The division process illustrates an infinite geometric series, where each new section of the square represents a successive power of one-fifth.
What is the sum of the areas of the shaded regions?
-The sum of the areas of the shaded regions is represented by the infinite series 1/5 + (1/5)^2 + (1/5)^3, and so on.
Why is the infinite sum of powers of one-fifth equal to one-fourth?
-The sum is equal to one-fourth because when the shape is rotated three more times to fill the entire square, the total shaded area corresponds to the sum of the geometric series, which converges to 1/4.
How does rotating the shape help explain the sum of the series?
-By rotating the shape three more times, it demonstrates that the infinite sum of the areas covers the entire square, proving that the total sum is one-fourth of the square’s area.
What is the significance of the infinite geometric series in this context?
-The infinite geometric series represents the progressively smaller areas of the inner square, illustrating how the sum of these infinite fractions converges to a finite value (one-fourth).
How does the process demonstrate the concept of infinity in geometry?
-The process shows that even though the division of the square into smaller and smaller sections can continue indefinitely, the sum of these sections converges to a finite value, illustrating the idea of infinite sums in geometry.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführen5.0 / 5 (0 votes)