Fourier Transform Explained
Summary
TLDRIn this video, we dive into the Fourier Transform, a key tool for analyzing signals in the frequency domain. The video explains how Fourier Series apply to periodic signals, and how the Fourier Transform is used for aperiodic signals, such as speech. It covers how these transforms enable us to move between the time and frequency domains, ultimately leading to a continuous frequency spectrum. The video also introduces the inverse Fourier transform and prepares viewers for upcoming lessons on its properties, especially in communications.
Takeaways
- 😀 Fourier Transform is a crucial tool for frequency domain analysis of signals and communication.
- 😀 Fourier Transform helps in finding the frequency spectra of aperiodic signals, unlike Fourier Series, which is used for periodic signals.
- 😀 Practical signals, like speech signals, are typically aperiodic and exist for a finite duration.
- 😀 The Fourier Transform allows us to analyze the frequency spectrum of a signal that exists only from a finite time t1 to t2.
- 😀 By applying the Fourier Transform to a periodic version of an aperiodic signal, we can generate the continuous frequency spectrum.
- 😀 The Fourier Transform of a periodic signal can be found by taking the limit as the time period (T0) tends to infinity.
- 😀 As T0 approaches infinity, the frequency spectrum becomes continuous rather than discrete.
- 😀 The inverse Fourier Transform allows us to reconstruct the time-domain signal from its frequency spectrum.
- 😀 When the time period (T0) of a periodic signal increases, the frequency components get denser, and the spectrum becomes more continuous.
- 😀 As the time period T0 tends to infinity, the summation over frequency components becomes an integral, representing the continuous frequency spectrum.
- 😀 The inverse Fourier Transform is used to reconstruct signals in the time domain, with the relationship defined by an integral that spans from minus infinity to plus infinity.
Q & A
What is the Fourier Transform, and why is it important in signal analysis?
-The Fourier Transform is a mathematical tool used to convert a signal from the time domain into the frequency domain. It is important in signal analysis because it helps to understand the frequency components of a signal, which is crucial in fields like communication and signal processing.
How does the Fourier Series differ from the Fourier Transform?
-The Fourier Series is used to analyze periodic signals by breaking them down into sums of sine and cosine functions. In contrast, the Fourier Transform is used for aperiodic signals, converting them into a continuous frequency spectrum.
What is the main distinction between periodic and aperiodic signals?
-Periodic signals repeat themselves after a fixed time period, while aperiodic signals exist for a finite time and do not repeat. Most practical signals, such as speech, are aperiodic.
Why do we need the Fourier Transform for aperiodic signals?
-Aperiodic signals do not have a simple frequency spectrum like periodic signals. The Fourier Transform helps find the continuous frequency spectrum for aperiodic signals, providing insight into their frequency components.
What happens to the frequency spectrum when the time period of a periodic signal is doubled?
-When the time period of a periodic signal is doubled, the frequency gets halved, and the amplitude or weightage of each frequency component decreases. As the time period increases, the spectral components become closer, leading to a denser frequency spectrum.
How is the Fourier Transform related to the concept of frequency spectra?
-The Fourier Transform helps convert a time-domain signal into its frequency spectrum, showing how much of each frequency component is present in the signal. This is especially useful for analyzing aperiodic signals, which don’t have a straightforward periodic frequency spectrum.
What does the inverse Fourier Transform do?
-The inverse Fourier Transform is used to reconstruct a signal in the time domain from its frequency spectrum. It essentially converts the frequency-domain representation back to the time-domain signal.
What role does the sampling of the frequency spectrum play in the Fourier Transform?
-Sampling the frequency spectrum involves taking specific samples of the continuous frequency function at intervals. As the time period (T0) tends to infinity, the sampling interval (f0) tends to zero, and the discrete frequency spectrum becomes a continuous spectrum.
What happens when the time period T0 tends to infinity in the Fourier Transform?
-As the time period T0 tends to infinity, the periodic signal becomes equivalent to the aperiodic signal, and the frequency spectrum transitions from discrete to continuous. This results in a continuous Fourier Transform instead of discrete coefficients.
What is the significance of the Δf in the context of the Fourier Transform?
-Δf represents the frequency interval between samples of the frequency spectrum. As the interval Δf tends to zero (when T0 tends to infinity), the frequency spectrum becomes continuous, and the summation over discrete frequencies is replaced by an integration over continuous frequencies.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة

The Fourier Transform in 15 Minutes
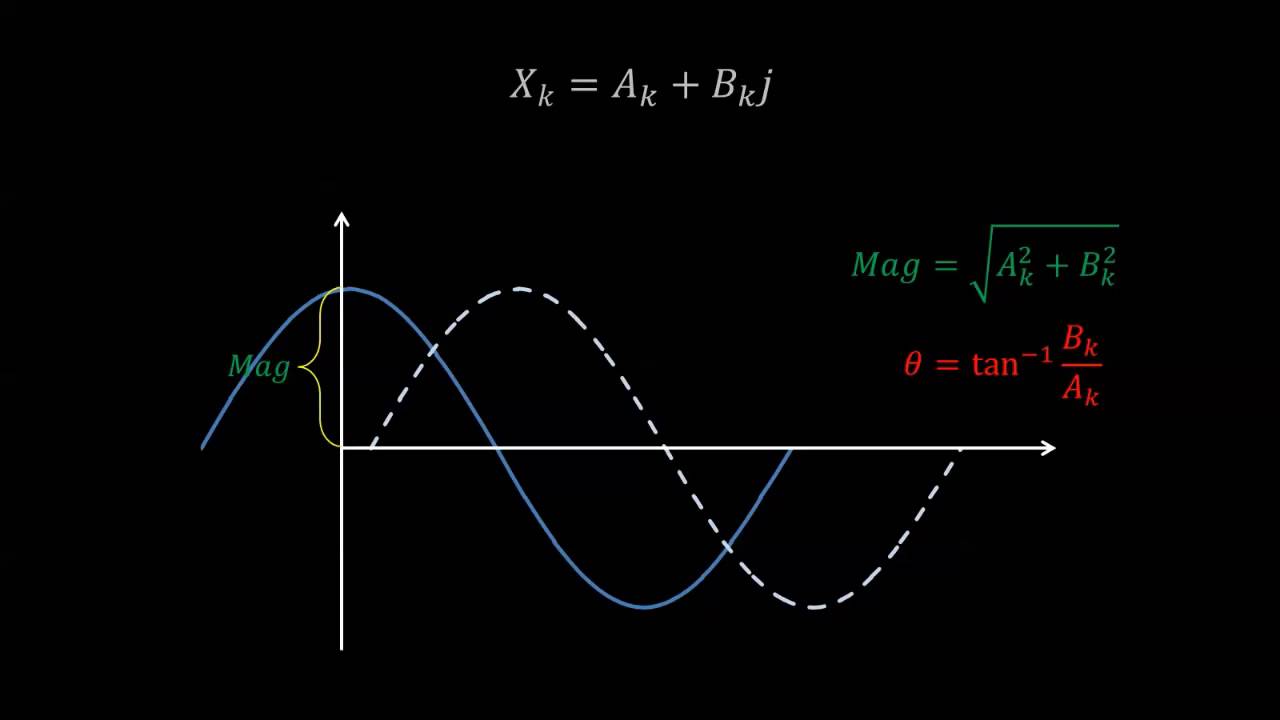
Discrete Fourier Transform - Simple Step by Step

Control Systems Lectures - Time and Frequency Domain

Understanding the Discrete Fourier Transform and the FFT

Introduction to Fourier Transform CTFT/FT (Continuous Time Fourier Transform)

What is the Fourier Transform used for?
5.0 / 5 (0 votes)
