Derivatives of Trigonometric Functions
Summary
TLDRIn this engaging lecture, Professor Dave explores the derivatives of trigonometric functions, including sine, cosine, tangent, cotangent, secant, and cosecant. He illustrates how to derive these functions through graphical interpretations and established rules like the product and quotient rules. The discussion emphasizes key relationships, such as the derivative of sine being cosine, and uses the Pythagorean identity to simplify expressions. Additionally, the lecture introduces the derivatives of inverse trigonometric functions, stressing the importance of memorization and comprehension for future calculus problems. This foundational knowledge is crucial for mastering differentiation involving trigonometric terms.
Takeaways
- 😀 The derivatives of trigonometric functions are essential for understanding calculus.
- 😀 The six primary trigonometric functions are sine, cosine, tangent, cosecant, secant, and cotangent.
- 😀 The derivative of sin(x) is cos(x), and the derivative of cos(x) is -sin(x).
- 😀 At the maxima and minima of sine and cosine functions, the slope (derivative) is zero.
- 😀 The derivative of tangent(x) is sec²(x), derived using the quotient rule.
- 😀 The derivative of cotangent(x) is -csc²(x), while the derivative of cosecant(x) is -csc(x)cot(x).
- 😀 The derivative of secant(x) is sec(x)tan(x).
- 😀 The product rule and quotient rule are crucial for finding the derivatives of products and quotients of functions.
- 😀 Examples in the script illustrate how to apply the product and quotient rules effectively.
- 😀 Familiarity with the derivatives of inverse trigonometric functions is important for solving more complex calculus problems.
Q & A
What is the main topic of Professor Dave's lesson?
-The main topic is how to take the derivatives of trigonometric functions, building on previously learned polynomial derivatives.
What are the six primary trigonometric functions discussed?
-The six primary trigonometric functions are sine (sin x), cosine (cos x), tangent (tan x), cosecant (csc x), secant (sec x), and cotangent (cot x).
How is the derivative of sine (sin x) determined?
-The derivative of sin x is determined by analyzing the slope of the tangent line, which equals zero at the maxima and minima. The derivative is found to be cosine (cos x).
What is the relationship between the graphs of sine and cosine functions?
-The derivative of sin x is cos x, which means the graph of the derivative corresponds to the graph of the cosine function, reflecting its behavior.
What is the derivative of cosine (cos x)?
-The derivative of cos x is negative sine (-sin x), which reflects its downward slope behavior.
What rule is applied to find the derivative of tangent (tan x)?
-The quotient rule is applied, leading to the derivative of tangent being secant squared (sec^2 x).
How do you find the derivative of cotangent (cot x)?
-The derivative of cotangent is derived to be negative cosecant squared (-csc^2 x) using similar rules.
What are the derivatives of the inverse trigonometric functions?
-The derivatives of the six inverse trigonometric functions are typically expressed as 1 over some expression involving x squared, often under a radical.
What example does Professor Dave provide for using the product rule?
-One example is finding the derivative of (x^2) * (sin x), which results in (x^2)(cos x) + (2x)(sin x).
What is the importance of memorizing trigonometric derivatives?
-Memorizing these derivatives is essential for solving problems involving trigonometric functions, as well as for applying product and quotient rules effectively.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة
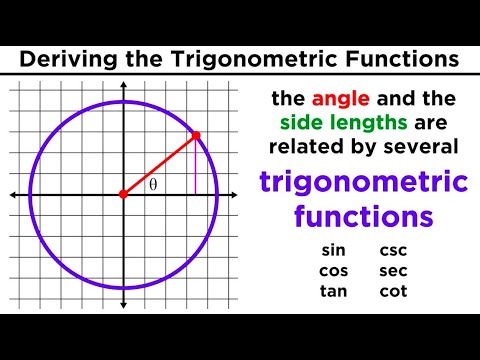
Trigonometric Functions: Sine, Cosine, Tangent, Cosecant, Secant, and Cotangent

Identitas Trigonometri - Part 1
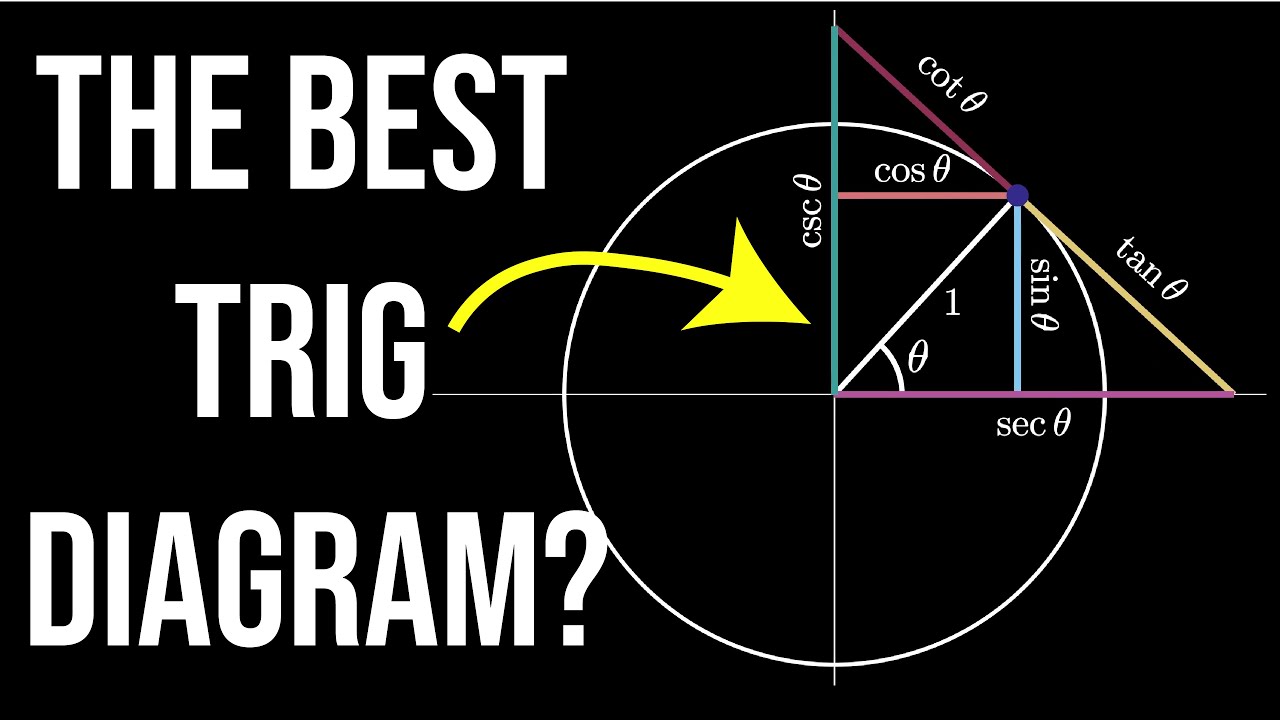
Trig Visualized: One Diagram to Rule them All (six trig functions in one diagram)

Identitas Trigonometri: Identitas Kebalikan, Perbandingan dan Pythagoras - SMA Kelas 10

Identitas Trigonometri | Matematika Wajib Kelas X

ILLUSTRATING CIRCULAR FUNCTIONS || PRE-CALCULUS
5.0 / 5 (0 votes)
