Trigonometric Functions: Sine, Cosine, Tangent, Cosecant, Secant, and Cotangent
Summary
TLDRIn this video, Professor Dave introduces trigonometric functions through a clear and engaging explanation. The video starts by connecting trigonometry to right triangles, explaining sine, cosine, and tangent, and how they relate to the sides of the triangle. The mnemonic SOHCAHTOA is introduced for easy recall. The video also explores reciprocal functions—cosecant, secant, and cotangent—and demonstrates how to calculate these for various triangles. Special triangles like 30-60-90 and 45-45-90 are covered in depth, helping viewers grasp the common values for sine, cosine, and tangent. The video concludes with a review to check comprehension.
Takeaways
- 😀 Trigonometry is based on triangles, and the relationship between the sides of a right triangle and an angle is key to understanding the trigonometric functions.
- 😀 The sine, cosine, and tangent functions relate the lengths of a right triangle's sides to the angle of interest.
- 😀 The sine of an angle is the ratio of the opposite side to the hypotenuse, the cosine is the ratio of the adjacent side to the hypotenuse, and the tangent is the ratio of the opposite side to the adjacent side.
- 😀 The mnemonic 'SOHCAHTOA' helps remember the definitions of sine, cosine, and tangent: Sine = Opposite/Hypotenuse, Cosine = Adjacent/Hypotenuse, Tangent = Opposite/Adjacent.
- 😀 Trigonometric functions can be evaluated using a triangle with known side lengths, such as the 3-4-5 triangle.
- 😀 Reciprocal trigonometric functions include cosecant (reciprocal of sine), secant (reciprocal of cosine), and cotangent (reciprocal of tangent).
- 😀 The special triangles—30-60-90 and 45-45-90 triangles—have specific side ratios that make evaluating trigonometric functions easier.
- 😀 For a 30-60-90 triangle, the side lengths follow a specific pattern: the shorter leg is 1, the longer leg is √3, and the hypotenuse is 2.
- 😀 The trigonometric values for 30°, 60°, and 45° angles can be derived from the side ratios of the special triangles.
- 😀 The values of sine, cosine, and tangent for special angles like 30°, 60°, and 45° are important to memorize as they appear frequently in trigonometric problems.
- 😀 To simplify trigonometric expressions, such as the tangent of 30° (1/√3), we multiply by √3/√3 to rationalize the denominator.
Q & A
What is the significance of radians in trigonometry?
-Radians provide a way to measure angles based on the radius of a circle. In trigonometry, they help to describe the relationship between the angle of rotation and the sides of a right triangle inscribed in a circle.
How is the trigonometric triangle constructed on the coordinate plane?
-A right triangle is formed by drawing a radius of a circle on the coordinate plane, then dropping a perpendicular from the endpoint of the radius to the x-axis. This creates a triangle with the radius as the hypotenuse and the x and y legs corresponding to the sides of the triangle.
What are the names of the sides of a right triangle in trigonometry?
-In a right triangle, the side adjacent to the angle of interest is called the 'adjacent leg,' the side opposite the angle is the 'opposite leg,' and the side opposite the right angle is the 'hypotenuse.'
What are the basic trigonometric functions and how are they defined?
-The basic trigonometric functions are sine (sin), cosine (cos), and tangent (tan). They are defined as: sin(θ) = opposite/hypotenuse, cos(θ) = adjacent/hypotenuse, and tan(θ) = opposite/adjacent.
What is the mnemonic to remember the definitions of the basic trigonometric functions?
-The mnemonic is SOHCAHTOA. It stands for Sine = Opposite/Hypotenuse, Cosine = Adjacent/Hypotenuse, and Tangent = Opposite/Adjacent.
How do you calculate the reciprocal functions in trigonometry?
-The reciprocal functions are calculated by inverting the basic functions: Cosecant (csc) is the reciprocal of sine (hypotenuse/opposite), secant (sec) is the reciprocal of cosine (hypotenuse/adjacent), and cotangent (cot) is the reciprocal of tangent (adjacent/opposite).
How do you calculate trigonometric functions for a given triangle?
-To calculate the trigonometric functions for a triangle, first identify the lengths of the sides, then apply the appropriate ratios. For example, if the sides are 3, 4, and 5, then sin(θ) = 3/5, cos(θ) = 4/5, and tan(θ) = 3/4.
What are the key properties of the 30-60-90 triangle in trigonometry?
-In a 30-60-90 triangle, if the shorter leg has a length of 1, the other sides will be root 3 and 2. The sine, cosine, and tangent values for 30° and 60° can be derived from the side lengths, and they are commonly used in trigonometric calculations.
What are the sine, cosine, and tangent values for 30°, 60°, and 45°?
-For a 30° angle, sin(30) = 1/2, cos(30) = √3/2, and tan(30) = √3/3. For a 60° angle, sin(60) = √3/2, cos(60) = 1/2, and tan(60) = √3. For a 45° angle, sin(45) = √2/2, cos(45) = √2/2, and tan(45) = 1.
Why is it important to memorize the sine, cosine, and tangent values for special triangles?
-Memorizing the sine, cosine, and tangent values for special triangles like the 30-60-90 and 45-45-90 triangles is essential because they frequently appear in trigonometric problems and simplify calculations.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
Derivatives of Trigonometric Functions

(TRIK) Perbandingan Trigonometri Sudut Berelasi #fazanugas

Anatomia da Pelve Feminina e Assoalho Pélvico ft. Lívia Rossi

Limit Fungsi Trigonometri Matematika Kelas 12 • Part 1: Konsep dan Penurunan / Pembuktian Rumus

Inverse Trigonometric Functions
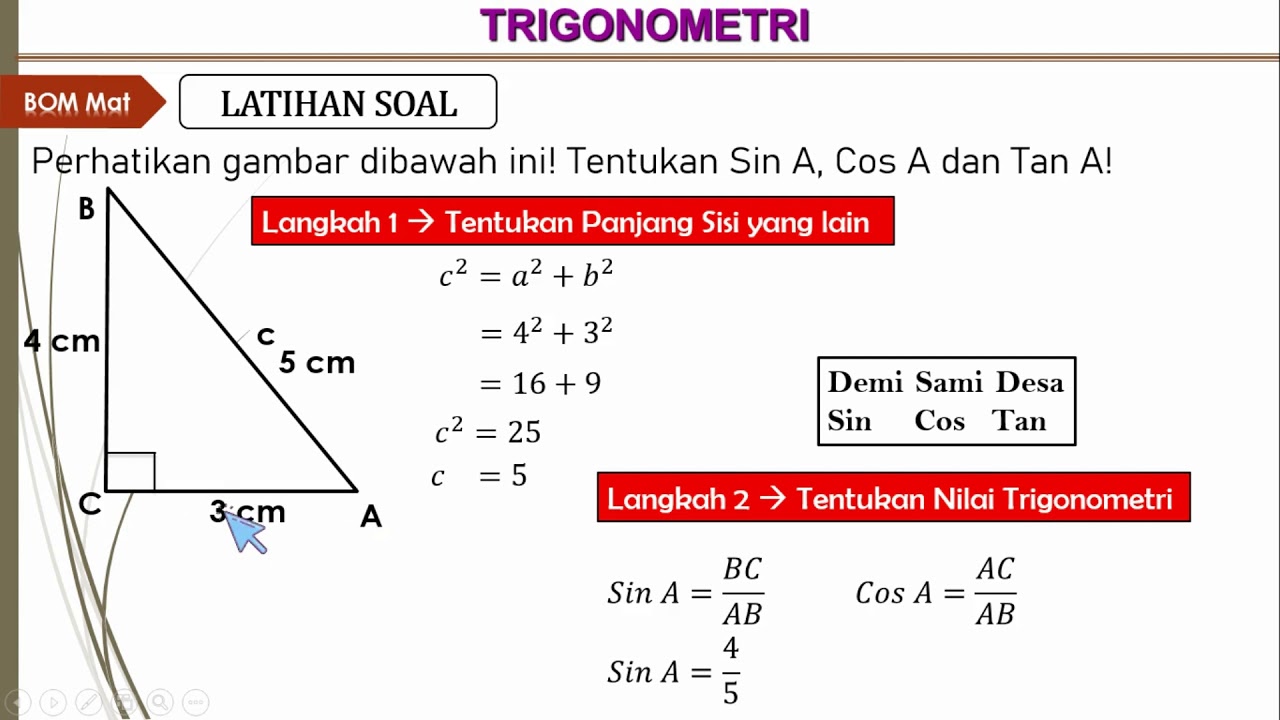
Perbandingan trigonometri pada segitiga siku siku, Menjelaskan rasio trigonometri
5.0 / 5 (0 votes)