Garis (2) Perbandingan Segmen Garis
Summary
TLDRThis educational video focuses on teaching the concept of line segment ratios in triangles, particularly the comparison of segments when a line is drawn parallel to one side. The instructor explains how to calculate the ratios of corresponding segments, such as comparing the lengths of segments AD to DB and AE to AC. Examples are provided to demonstrate how to solve problems using these ratios, making use of proportionality principles. The video encourages students to practice solving problems through exercises and apply the methods taught for better understanding.
Takeaways
- 😀 The lesson is focused on comparing line segments in triangles using proportional relationships.
- 😀 In triangle ABC, drawing a parallel line DE creates proportional relationships between various segments.
- 😀 The key proportional relationships to understand are: AD/DB = AE/AC and AD/AB = DE/BC.
- 😀 To😀 To solve problems, identify the corresponding line segments and set up the correct proportion.
- 😀 In Example 1, the length of AE is calculated using the ratio AD/DB = AE/AC, yielding AE = 2 cm.
- 😀 In Example 2, the length of DE is determined using the proportion AD/AB = DE/BC, resulting in DE = 8 cm.
- 😀 Proportional relationships are a helpful tool in solving problems involving parallel lines in triangles.
- 😀 The principle of parallel lines creating equal ratios is critical in understanding and solving the problems.
- 😀 To solve for unknown lengths, use cross-multiplication when solving proportions.
- 😀 Consistently practice using proportional relationships to build a solid understanding of segment comparisons in geometry.
Q & A
What is the main topic of the lesson in the transcript?
-The main topic is about 'Line Segment Ratios' or 'Ratios of Line Segments' in a triangle, specifically the relationship between various parts of a triangle when a line is drawn parallel to one of its sides.
What is the significance of the line drawn from point D to point E in the triangle?
-The line drawn from point D to point E is parallel to the base of the triangle, BC. This parallelism forms proportional relationships between the segments of the triangle.
What does the symbol '||' signify in this context?
-The symbol '||' indicates that two lines are parallel. In the script, 'DE || BC' means that line DE is parallel to line BC.
What is the first ratio or proportion introduced in the lesson?
-The first ratio introduced is the comparison between the lengths of the segments AD and DB, which is proportional to the comparison between AE and AC.
What is the second ratio discussed in the lesson?
-The second ratio discussed is the comparison between the lengths of AD and AB, which is proportional to the comparison between AE and AC.
How do we calculate the unknown segment AE in the first example?
-In the first example, the ratio AD/DB = AE/AC is used. Given that AD is 3 cm, DB is 6 cm, and AC is 4 cm, we solve for AE. The calculation is AE = (3 * 4) / 6, which gives AE = 2 cm.
What method is used to solve the example where AE is unknown?
-The method used is cross-multiplication, where the known values are plugged into the proportion, and then the unknown is solved by isolating AE.
What changes in the second example when solving for AE?
-In the second example, the known values are different. AD is 4 cm, DB is 2 cm, and BC is 12 cm. The ratio used is AD/AB = AE/BC. We calculate AE by first finding the length of AB, which is AD + DB = 4 + 2 = 6 cm, and then solving for AE using the proportion.
How do we calculate the length of line segment AB in the second example?
-In the second example, the length of AB is found by adding the lengths of AD (4 cm) and DB (2 cm), so AB = 4 cm + 2 cm = 6 cm.
What is the final result for AE in the second example?
-The final result for AE in the second example is 8 cm, which is found by solving the proportion using the formula AE = (4 * 12) / 6.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
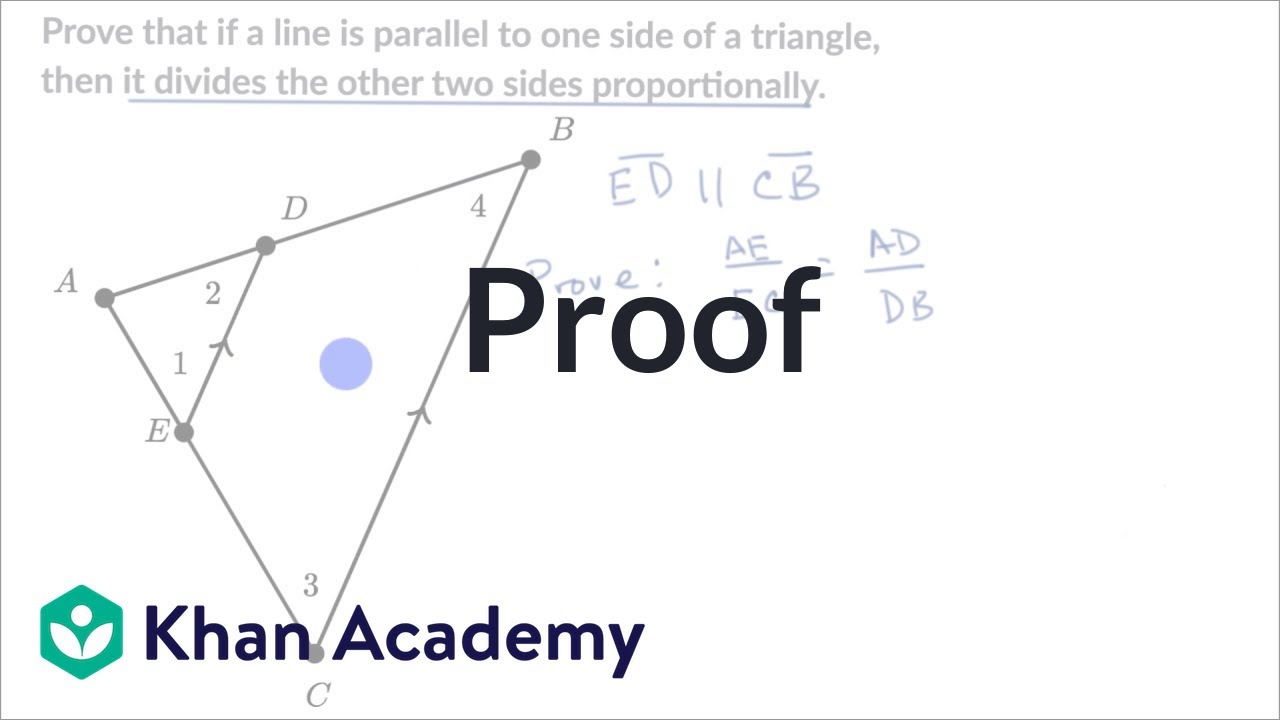
Proof: Parallel lines divide triangle sides proportionally | Similarity | Geometry | Khan Academy

02 Proportionality Theorems

MEMBAGI RUAS GARIS (Menentukan bagian ruas garis dengan perbandingan)

GEO.1.2

Pengertian Kemiringan Hal 239-243 Bab 5 Persamaan Garis Lurus Kelas 8 Kurikulum Merdeka Belajar
Naming a Polygon and Its Parts | Matatag Curriculum | Grade 7 | Explain in Detailed |
5.0 / 5 (0 votes)
