Trigonometri #14 - Sudut Elevasi dan Sudut Depresi
Summary
TLDRIn this video, the presenter explains the concepts of elevation and depression angles in trigonometry. Using visual examples such as a person observing a bird flying or looking at a chicken on the ground, the presenter defines elevation as the angle formed when looking upward and depression as the angle formed when looking downward. Practical applications are discussed, such as determining the height of a tree or a building using trigonometric principles. The use of a clinometer to measure these angles and solve real-life problems is also explored, along with examples involving bridges and water currents.
Takeaways
- 😀 The angle of elevation is the angle formed when an observer looks upward from a horizontal line to an object.
- 😀 The angle of depression is the angle formed when an observer looks downward from a horizontal line to an object.
- 😀 Both angles are measured between the line of sight and the horizontal plane (eye level).
- 😀 In practical applications, these angles help in measuring the height of objects or distances that are not directly measurable.
- 😀 A clinometer can be used to measure the angle of elevation or depression to calculate unknown heights or distances.
- 😀 Trigonometry, particularly the tangent and sine functions, are key to solving problems involving the angles of elevation and depression.
- 😀 For example, to find the height of a tree, you can use the tangent function if the angle of elevation and the distance to the tree are known.
- 😀 In a practical problem, if the observer is 6 meters away from a tree and the angle of elevation is 30°, the height of the tree can be calculated using trigonometric ratios.
- 😀 For the angle of depression, if an observer is above an object, trigonometric functions like sine can help calculate the height between the observer and the object.
- 😀 In some cases, special angles like 30° or 45° are used because they simplify calculations due to their known trigonometric values.
- 😀 The angle of depression is also used in situations like measuring the height of a bridge from its top to the water below.
Q & A
What is the concept of 'angle of elevation' as described in the video?
-The angle of elevation is the angle formed between the line of sight looking upwards and the horizontal line from the observer's eye level. It occurs when an observer looks at an object above them, such as a bird flying in the sky.
How does the video explain the 'angle of depression'?
-The angle of depression is the angle formed between the line of sight looking downwards and the horizontal line from the observer's eye level. This happens when an observer looks at something below them, such as a chicken on the ground.
Can you explain the practical application of the angle of elevation and depression in measuring the height of objects?
-The video shows that angles of elevation and depression can be used to determine the height of objects like trees, poles, or buildings. By using a clinometer to measure the angle, one can apply trigonometric principles to calculate the height based on the known distance and angle.
How does the video use a real-life example to demonstrate the concept of elevation?
-In the video, the example of observing the top of a tree is used. The observer, standing a certain distance away, looks upwards at the top of the tree, forming an angle of elevation. This angle, along with the distance from the tree, helps in calculating the height of the tree using trigonometric formulas.
What mathematical method is used to calculate the height of the tree in the example?
-The video uses trigonometry, specifically the tangent function, to calculate the height of the tree. With a known angle of elevation and the horizontal distance to the tree, the height can be calculated using the formula: tan(angle) = opposite/adjacent.
What is the significance of the angle 30 degrees in the example?
-In the video, the 30-degree angle is used as an example of a special angle where trigonometric values are easy to work with. The ratio for tan 30 degrees is √3/3, making calculations more straightforward. It also helps demonstrate the application of trigonometry with known angles.
How does the clinometer help in measuring angles for such calculations?
-A clinometer is used to measure the angle of elevation or depression. By aligning it with the object being observed, the angle can be read off the device. This angle is then used in trigonometric calculations to determine the height of objects.
What is the purpose of using a 45-degree angle in the video example?
-The 45-degree angle is chosen because, in a right-angled triangle, when the angle is 45 degrees, the lengths of the adjacent and opposite sides are equal. This makes the calculation simpler, as the height of the object will be equal to the distance from the observer to the object.
How is the angle of depression used to measure the height of a bridge in the video?
-The video describes using the angle of depression to measure the height of a bridge above a river. A rope is tied with a float, and the angle of depression to the water's surface is measured. Using this angle, trigonometric principles, and the length of the rope, the height of the bridge can be calculated.
Why is it important to understand the concept of elevation and depression in real-world applications?
-Understanding angles of elevation and depression is crucial for various real-world applications, such as determining the height of buildings, trees, or bridges, surveying land, and even in navigation. These concepts allow precise measurements without the need for direct measurement, especially in cases where access to the object is difficult.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

Perbandingan Trigonometri: Pembahasan Soal Cerita
TRIGONOMETRI (5): PEMBAHASAN SOAL KONTEKSTUAL PERBANDINGAN TRIGONOMETRI ATAU APLIKASI SEHARI-HARI
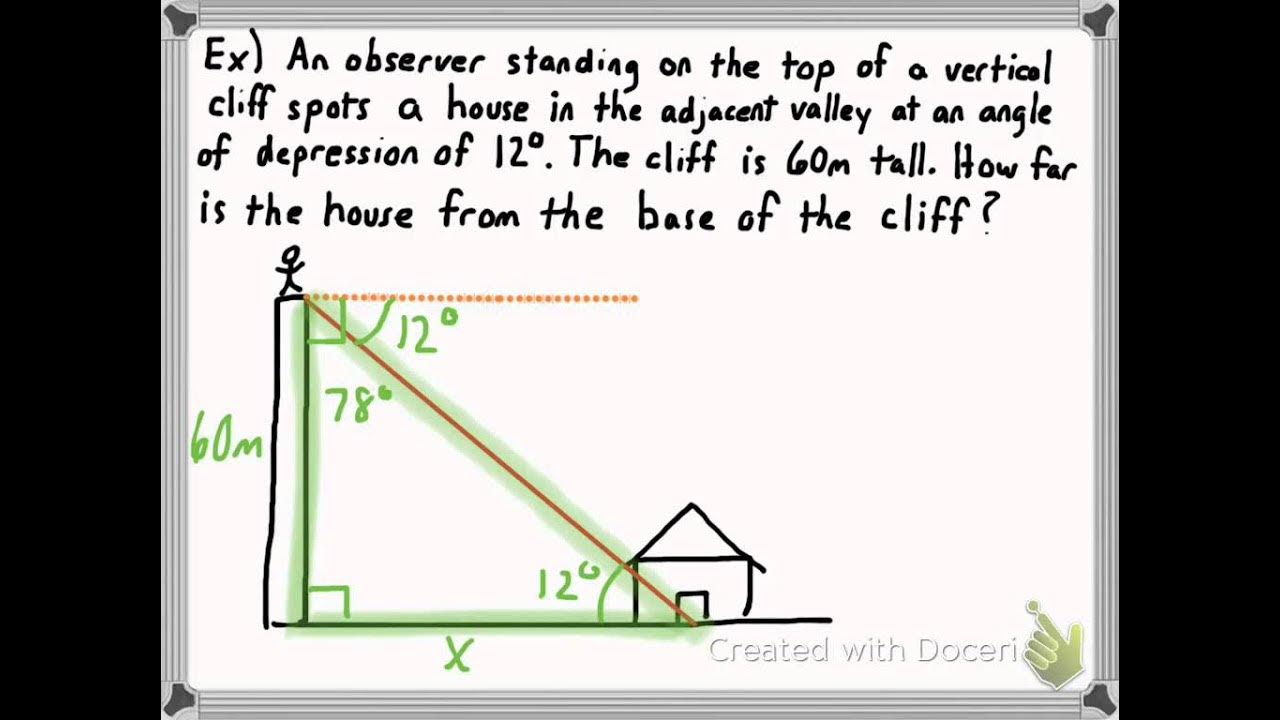
Angle of Elevation/Angle of Depression Problems
Matematika SMA - Trigonometri (2) - Aplikasi Perbandingan Trigonometri, Sudut Depresi & Elevasi (A)

Perbandingan Trigonometri Part 2- Mengukur Tinggi Benda dengan Clinometer

Membuat Alat Klinometer dan Penggunaan Alat Peraga Klinometer
5.0 / 5 (0 votes)
