Progression and Series Part 11 IIT JEE Maths
Summary
TLDRThis video script explains the mathematical principles behind arithmetic progressions (AP), specifically focusing on the sum of the first 'n' terms and the relationship between the common difference and the quadratic expressions of sums. It discusses key formulas and properties, such as the effect of a negative common difference and how to determine the common difference from the quadratic form of a series. The explanation also includes detailed steps for calculating terms and the significance of quadratic sums in determining the nature of the sequence. Overall, it highlights the mathematical intricacies of APs and their algebraic structures.
Takeaways
- 😀 The common difference (d) in an arithmetic progression (AP) determines the behavior of the terms, and if it's negative, the nth term (Tn) will also be affected.
- 😀 The sum of the first n terms of an AP can be represented by the formula: S_n = n * (2a - d), where 'a' is the first term and 'd' is the common difference.
- 😀 The nth term in an AP series follows a linear pattern, while the sum of terms results in a quadratic expression as 'n' increases.
- 😀 A quadratic expression for the sum of terms in an AP indicates that the series is indeed an arithmetic progression, and the constant term will be zero.
- 😀 The difference between consecutive terms (Tn - Tn-1) in an AP is constant, which is a key property of an arithmetic series.
- 😀 If the sum of the first n terms yields a quadratic expression with no constant term, this confirms the series is an AP.
- 😀 The sum of terms in an AP results in a quadratic function, and the coefficient of the n² term directly relates to the common difference.
- 😀 The quadratic form of the sum formula confirms that the common difference is consistent, and the series follows an arithmetic progression.
- 😀 When the coefficient of n² is doubled, it matches the common difference, further proving the arithmetic nature of the series.
- 😀 In the context of this AP, proving the constancy of the common difference (by showing Tn - Tn-1 is constant) validates that the series behaves as an arithmetic progression.
Q & A
What happens when the common difference (d) in an arithmetic progression (AP) is negative?
-When the common difference is negative, the terms of the arithmetic progression decrease rather than increase. This affects the general behavior of the terms, as each subsequent term is smaller than the previous one.
How do you find the sum of the first n terms (S_n) in an arithmetic progression?
-The sum of the first n terms in an AP is given by the formula: S_n = n * (2a + (n - 1) * d), where a is the first term, d is the common difference, and n is the number of terms.
What does it mean when the sum of the first n terms forms a quadratic expression?
-When the sum of the first n terms forms a quadratic expression, it indicates that the series is an arithmetic progression (AP). The quadratic nature arises because the sum is dependent on the square of n, with a constant term of zero.
How is the common difference (d) related to the quadratic expression of an arithmetic progression?
-In a quadratic expression for the sum of an AP, the coefficient of the n^2 term represents half of the common difference. This is because the second difference of the terms in the AP is constant, which leads to the quadratic form.
What is the significance of the difference between consecutive terms (t_n - t_(n-1)) in proving the properties of an AP?
-The difference between consecutive terms (t_n - t_(n-1)) in an AP is constant. This constant difference helps prove that the series follows an arithmetic progression, as it shows the regular pattern of change between consecutive terms.
How can we prove that a series with a quadratic sum is an arithmetic progression?
-We can prove that a series with a quadratic sum is an AP by showing that the difference between consecutive terms is constant. If this difference is consistent, the series is confirmed to be an AP.
What role does the coefficient of n^2 play in identifying an arithmetic progression?
-The coefficient of n^2 in the quadratic expression for the sum of terms represents the half of the common difference. By examining this coefficient, we can identify the common difference and confirm that the series is an arithmetic progression.
What is the relationship between the quadratic sum and the common difference in an arithmetic progression?
-In an arithmetic progression, the quadratic sum’s second-degree term (n^2) indicates the common difference. The common difference is directly related to the coefficient of n^2, and its value can be determined by analyzing this coefficient.
What happens when the constant term in a quadratic sum is zero?
-When the constant term in a quadratic sum is zero, it suggests that the series is an arithmetic progression. The quadratic form with no constant term indicates a consistent change in the terms, which is characteristic of an AP.
What is the formula for finding the common difference from the quadratic sum?
-To find the common difference from the quadratic sum, observe the coefficient of n^2 in the formula. This coefficient, when doubled, gives the value of the common difference in the arithmetic progression.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

ARITHMETIC PROGRESSIONS in 30 Minutes || Mind Map Series for Class 10th

Barisan dan deret Geometri kelas 10
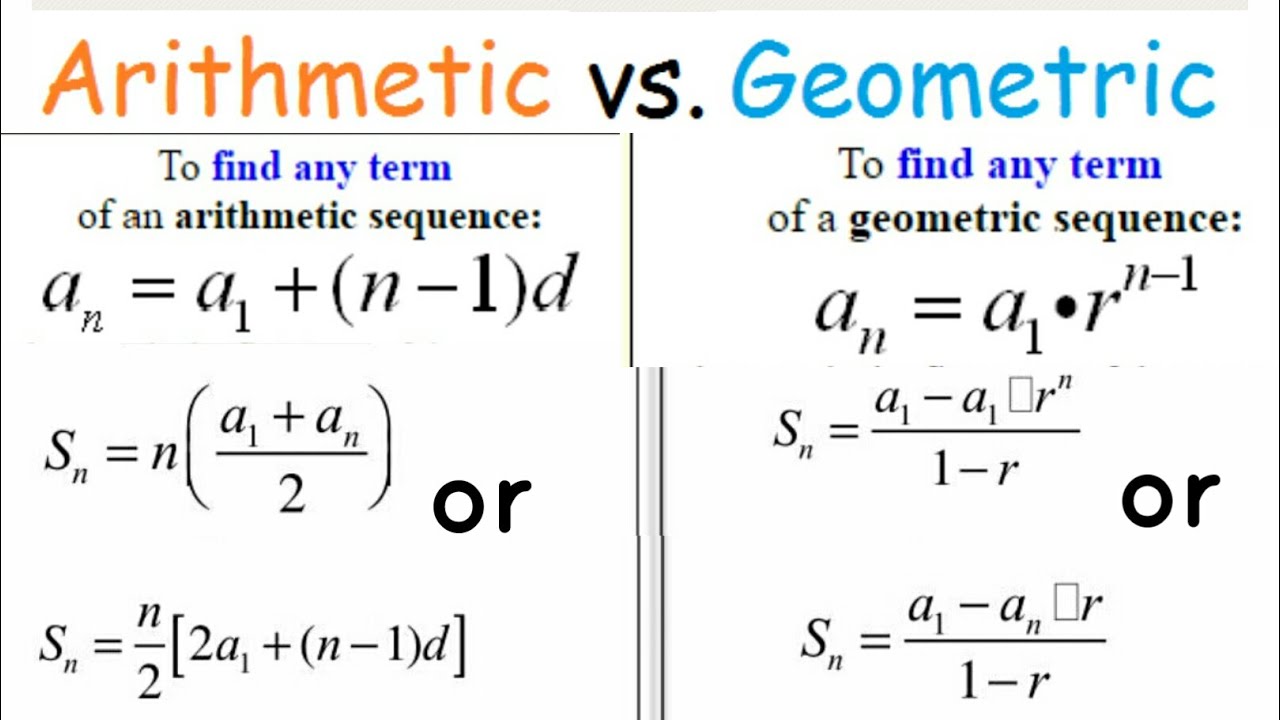
Arithmetic Sequence vs Geometric Sequence

Progressão Aritmética PA: Soma dos Termos (aula 6 de 6)

Konsep Dasar Baris dan Deret Aritmatika | Matematika Kelas X Fase E Kurikulum Merdeka

Arithmetic Series - Sum of the Terms of Arithmetic Sequence
5.0 / 5 (0 votes)
