EXPLORING RANDOM VARIABLES | DISCRETE AND CONTINUOUS | PROF D

Summary

This video introduces random variables, explaining how they are functions that assign real numbers to outcomes in a random experiment. It covers the two types of random variables—discrete and continuous—and provides examples such as tossing coins, rolling dice, and testing for COVID-19 infections. The video also outlines the process for determining the values of random variables through various experiments. Viewers learn to classify variables as either discrete or continuous, based on whether their values are counted or measured, with practical examples from real-life scenarios.

Takeaways

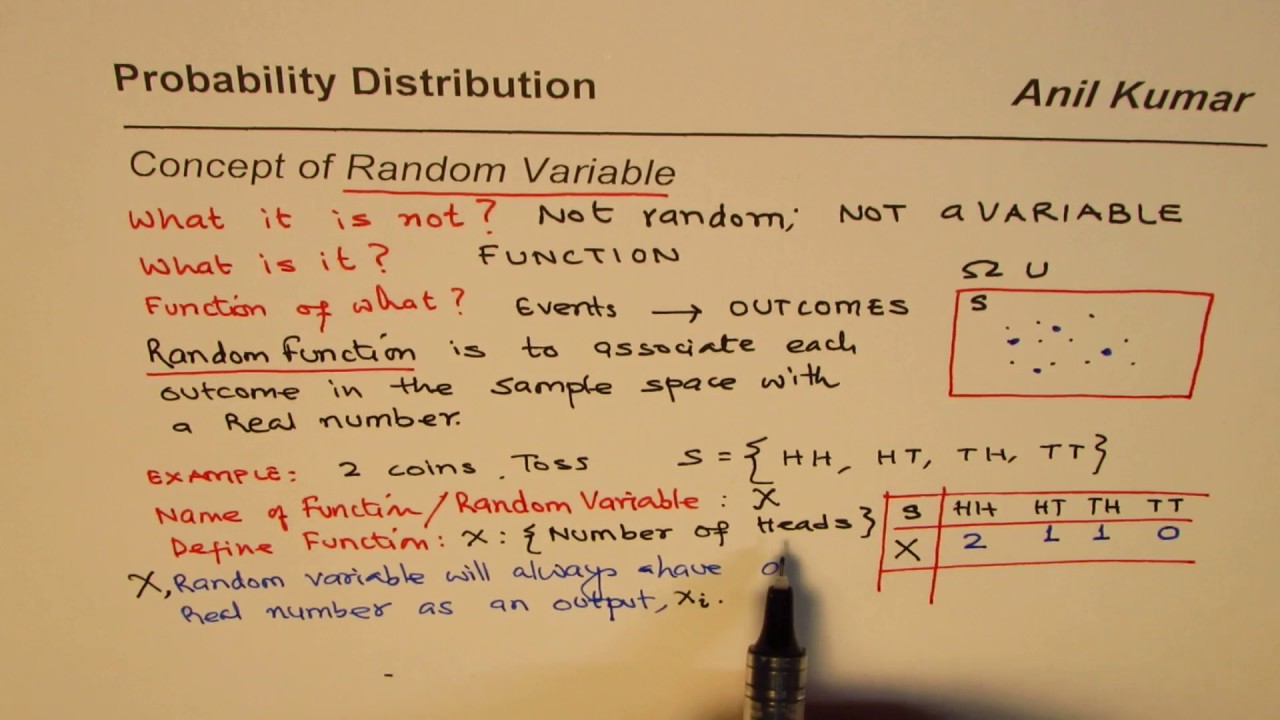
• 😀 A random variable is a function that associates a real number with each element in the sample space of a random experiment.
• 😀 The sample space refers to the collection of all possible outcomes of a random experiment.
• 😀 A random variable can be thought of as a numerical quantity derived from the outcomes of a random experiment.
• 😀 Example 1: When tossing a coin, the number of heads is a random variable.
• 😀 Example 2: When rolling two dice, the sum of the numbers rolled is a random variable.
• 😀 Example 3: Spinning a spinner and recording the number of times it stops on a particular number is also a random variable.
• 😀 The steps for finding the values of random variables in an experiment include assigning letters to represent outcomes, determining the sample space, and counting the number of random variables.
• 😀 Discrete random variables can only take a finite number of distinct values and are often represented by whole numbers.
• 😀 Continuous random variables can assume an infinite number of values within an interval and may be represented by decimals and fractions.
• 😀 The key difference between discrete and continuous random variables is that discrete variables are counted, while continuous variables are measured.
• 😀 Examples of discrete variables include the number of patients in a hospital, the number of male athletes, and the number of deaths from COVID-19, while examples of continuous variables include temperature and the amount of sugar in a cup of coffee.

Q & A

What is a random variable?

A random variable is a function that assigns a real number to each outcome in a sample space, where the values are determined by chance. It is a numerical quantity derived from the outcomes of a random experiment.

What is an example of a random variable in a coin tossing experiment?

In a coin tossing experiment, the number of times the coin turns up heads is an example of a random variable.

How do you determine the values of a random variable?

To determine the values of a random variable, you assign letters to represent each outcome, determine the sample space, and count the number of occurrences of the random variable in each possible outcome.

What are the possible values of the random variable in the example of tossing three coins?

In the example of tossing three coins, the possible values of the random variable (the number of heads) are 0, 1, 2, and 3.

How are random variables classified into discrete and continuous types?

Discrete random variables can only take a finite number of distinct values and are represented by non-negative whole numbers. Continuous random variables can take an infinite number of values in an interval and can be represented by fractions and decimals, often derived from measurements.

What is an example of a discrete random variable?

An example of a discrete random variable is the number of patients per day at a hospital, which is counted in whole numbers.

What is an example of a continuous random variable?

An example of a continuous random variable is the temperature of COVID-19 patients, which can take on an infinite number of values, including fractions and decimals.

How is the number of deaths from COVID-19 categorized as a random variable?

The number of deaths from COVID-19 is categorized as a discrete random variable because it is counted in whole numbers and has finite possible outcomes.

What differentiates discrete random variables from continuous ones?

The key difference is that discrete random variables are countable and take finite values, while continuous random variables can assume an infinite number of values, often resulting from measurements.

How would you classify the amount of sugar in a cup of coffee?

The amount of sugar in a cup of coffee is a continuous random variable because it is measured and can take any value within a range, including fractions or decimals.