Calculo Integral 03: Notación Sigma. Sigma notation.
Summary
TLDRIn this lesson, the concept of Sigma notation for summing infinite series is introduced. The instructor demonstrates how to represent a series using Sigma notation, providing examples with varying limits and terms. Key properties of Sigma notation, such as how constants can be factored out and how sums are distributed over addition and subtraction, are explained. The class also covers common series formulas, such as those for the sum of squares and cubes. The lesson concludes with a practical exercise and a preview of the next class, which will introduce the concept of the definite integral.
Takeaways
- 😀 Sigma notation is a simpler way to represent infinite series and sums.
- 😀 The index used in the Sigma notation (e.g., 'i', 'j', or 'k') can vary, and it’s common to use negative indices.
- 😀 Sigma notation sums terms based on an index that ranges from a lower limit to an upper limit.
- 😀 A constant in the summation expression can be factored out of the Sigma notation.
- 😀 When summing constants, the result is the constant multiplied by the number of terms in the sum.
- 😀 The sum of the first 'n' positive integers is given by the formula n(n+1)/2.
- 😀 The sum of the squares of the first 'n' integers is n(n+1)(2n+1)/6.
- 😀 The sum of cubes of the first 'n' integers is the square of the sum of the first 'n' integers, i.e., (n(n+1)/2)².
- 😀 When applying properties of Sigma notation, one can separate the sum of different terms (e.g., sum of squares, sum of linear terms).
- 😀 A common mistake is assuming a sum of constants results in the constant alone, when it should be multiplied by 'n' (the number of terms).
- 😀 Understanding how to apply Sigma notation properties helps solve complex expressions, such as finding the limit of sums as 'n' approaches infinity.
Q & A
What is the main purpose of using Sigma notation in the class?
-The main purpose of using Sigma notation is to simplify the representation of an infinite series and to easily express summations of terms in a concise way.
What does Sigma notation represent when summing from i = 1 to n?
-Sigma notation represents the sum of terms generated by the expression i squared, where the value of i is substituted from 1 to n.
Can the index of summation in Sigma notation be any letter?
-Yes, the index can be any letter, although i, j, and k are commonly used for simplicity, especially when multiple indices are needed.
What does the property of Sigma notation say about multiplying by a constant?
-The property of Sigma notation states that a constant factor, which does not depend on the index, can be taken outside the summation symbol.
What is the common error when summing a constant c multiple times in Sigma notation?
-A common error is assuming that the sum of a constant c from i = 1 to n is simply c. The correct result is n times c, since you are summing c n times.
How is the sum of the first n integers expressed using Sigma notation?
-The sum of the first n integers is expressed as n * (n + 1) / 2.
What is the formula for the sum of the squares of the first n positive integers?
-The formula for the sum of the squares of the first n positive integers is n * (n + 1) * (2n + 1) / 6.
What is the formula for the sum of the cubes of the first n natural numbers?
-The formula for the sum of the cubes of the first n natural numbers is (n * (n + 1) / 2) squared.
How was the summation of the expression (i - 1) * (i + 3) simplified in the script?
-The summation was simplified by first expanding the product (i - 1) * (i + 3) to i^2 + 2i - 3, and then splitting the summation into three parts: the sum of i^2, 2 times the sum of i, and the sum of -3.
What was the final result of the limit calculation in the script?
-The final result of the limit calculation as n approaches infinity was 7/3.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

GENERATING PATTERNS IN SERIES || GRADE 10 MATHEMATICS Q1

01. Notación sigma (Sumatorias) ¿qué es? ¿cómo se usa?

Notasi Sigma Matematika Kelas 11 • Part 1: Pengertian Notasi Sigma

Barisan Deret ( Notasi Sigma )

Integral Tentu - Apa hubungannya dengan notasi sigma? | Integral Tentu (Part 1) | Kalkulus
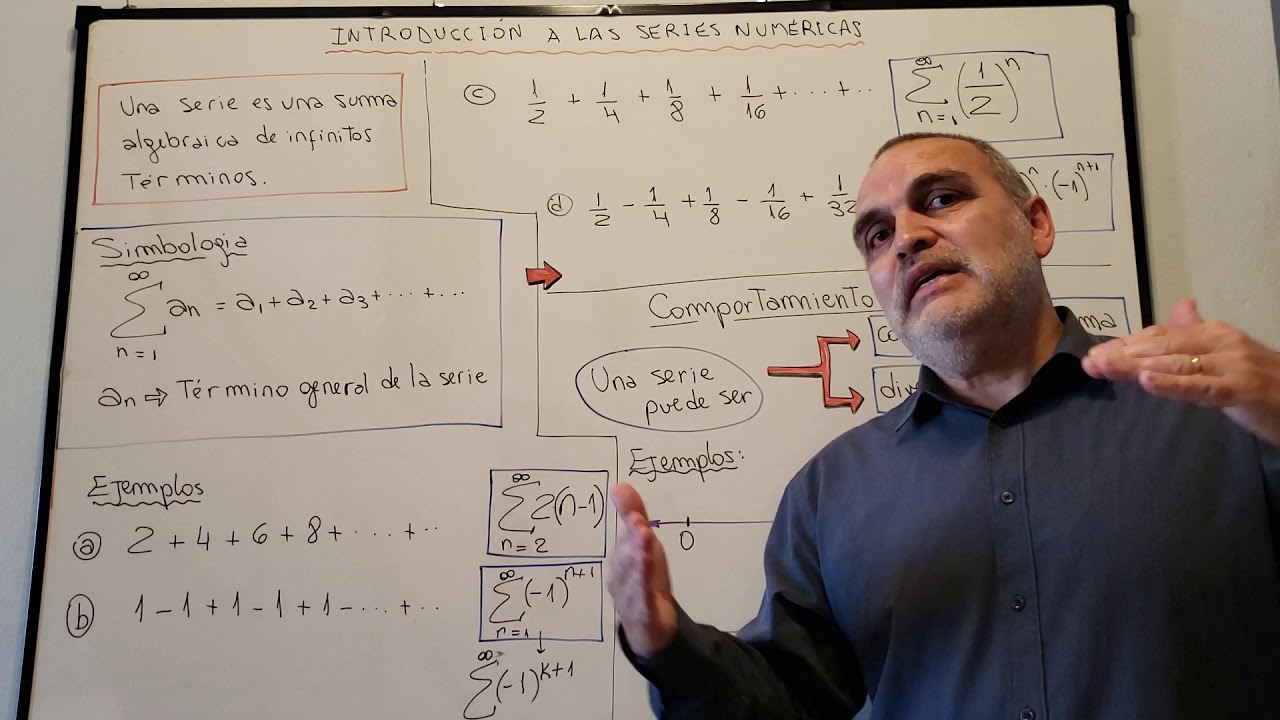
Introducción a las Series Numéricas.
5.0 / 5 (0 votes)
