Introducción a las Series Numéricas.

Summary

This introductory video lesson explains the concept of numerical series, their symbolic representation, and how they behave. It covers the definition of a series as an algebraic sum of infinite terms, offering examples of both convergent and divergent series. The presenter demonstrates different ways to represent series, such as summing even numbers, alternating sums, and powers of fractions. The lesson also explores the difference between convergent and divergent series, highlighting how the sum of terms in a convergent series approaches a specific value, while a divergent series continues to grow without bound.

Q & A

What is the main focus of the video transcript?

The main focus of the video transcript is an introduction to numerical series, specifically how to define, represent, and analyze the behavior of series, with examples of convergent and divergent series.

How are numerical series represented symbolically?

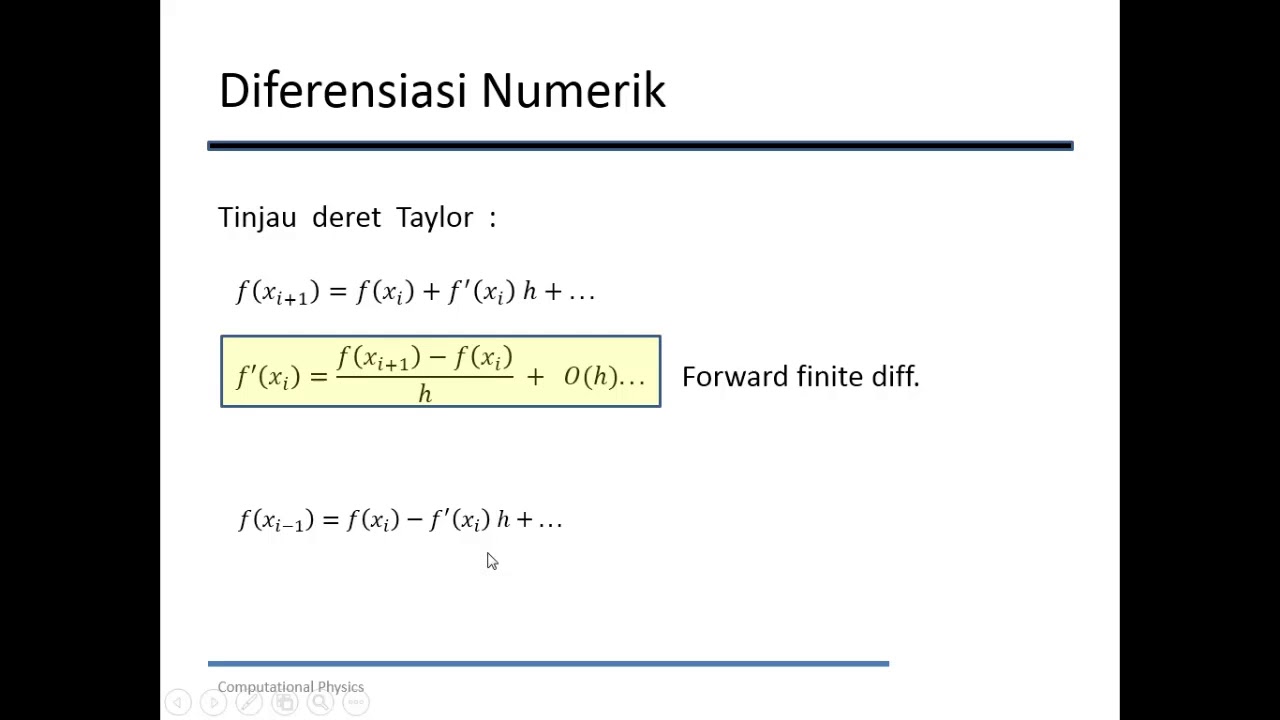
Numerical series are represented symbolically using summation notation, such as the summation from n = 1 to infinity, where each term is represented by a general expression depending on n.

What is the difference between a convergent and a divergent series?

A convergent series has a finite sum that approaches a specific value as the terms are added, while a divergent series does not have a finite sum and its terms keep increasing or oscillating without settling to a particular value.

Can you give an example of a divergent series from the transcript?

An example of a divergent series in the transcript is the sum of even numbers (2, 4, 6, 8, ...), where the sum keeps growing without bound because the terms are getting larger.

What example is given to illustrate a convergent series?

The transcript uses the series of fractions like 1/2, 1/4, 1/8, 1/16, ... to illustrate a convergent series. As more terms are added, the sum gets closer and closer to 1 but never quite reaches it.

How does the series of alternating numbers (1, -1, 1, -1, ...) behave in terms of convergence or divergence?

The alternating series (1, -1, 1, -1, ...) is an example of a divergent series. While the partial sums oscillate between 1 and 0, they never settle at a fixed value, so the series does not have a finite sum.

What does it mean when we say a series has a 'sum' in the context of convergence?

When a series is convergent, it means that the sum of the infinite terms approaches a specific value as more terms are added. Although the sum never fully reaches that value, it gets infinitely close to it.

What is the role of the 'summation notation' in representing series?

Summation notation provides a compact and symbolic way to represent the sum of infinite terms in a series. It allows for easy expression of complex series with variables and operations applied to them.

How is the convergence of the series demonstrated using a calculator in the transcript?

The convergence of a series is demonstrated by adding successive terms using a calculator. As the terms are added, the sum gets closer to 1, showing that the series converges towards this value.

What intuition does the transcript give regarding the concept of 'closeness' in a convergent series?

The transcript emphasizes that while a convergent series may never exactly reach its final sum (e.g., it gets infinitely close to 1), the terms of the series continue to get closer and closer, illustrating the concept of convergence as an approximation.