MekFlu #1: Prinsip Persamaan Bernoulli
Summary
TLDRThis video lecture explains Bernoulli's equation in fluid dynamics, covering its derivation, key principles, and applications. It starts with a review of basic fluid properties and the distinction between fluid statics and dynamics. The lecture then explores how Newton's second law is used to derive Bernoulli’s equation, assuming incompressible and inviscid flow. The equation, which relates pressure, velocity, and height, is fundamental to understanding fluid behavior in various applications, such as airflow over wings and fluid flow in pipes. The video concludes with real-world examples and an overview of the equation's significance in engineering.
Takeaways
- 😀 The lecture focuses on Bernoulli's equation, a fundamental principle in fluid mechanics, used in various engineering fields like environmental engineering.
- 😀 Bernoulli's equation was developed by Daniel Bernoulli in the 18th century, and it plays a crucial role in understanding fluid behavior during flow.
- 😀 Bernoulli's equation is derived using Newton's second law of motion, applying it to a fluid particle moving along a streamline.
- 😀 The equation is based on the assumption of incompressible (constant density) and inviscid (no friction) flow conditions.
- 😀 The two primary forces acting on a fluid are gravitational force (weight of the fluid) and pressure force, both of which are crucial in deriving Bernoulli's equation.
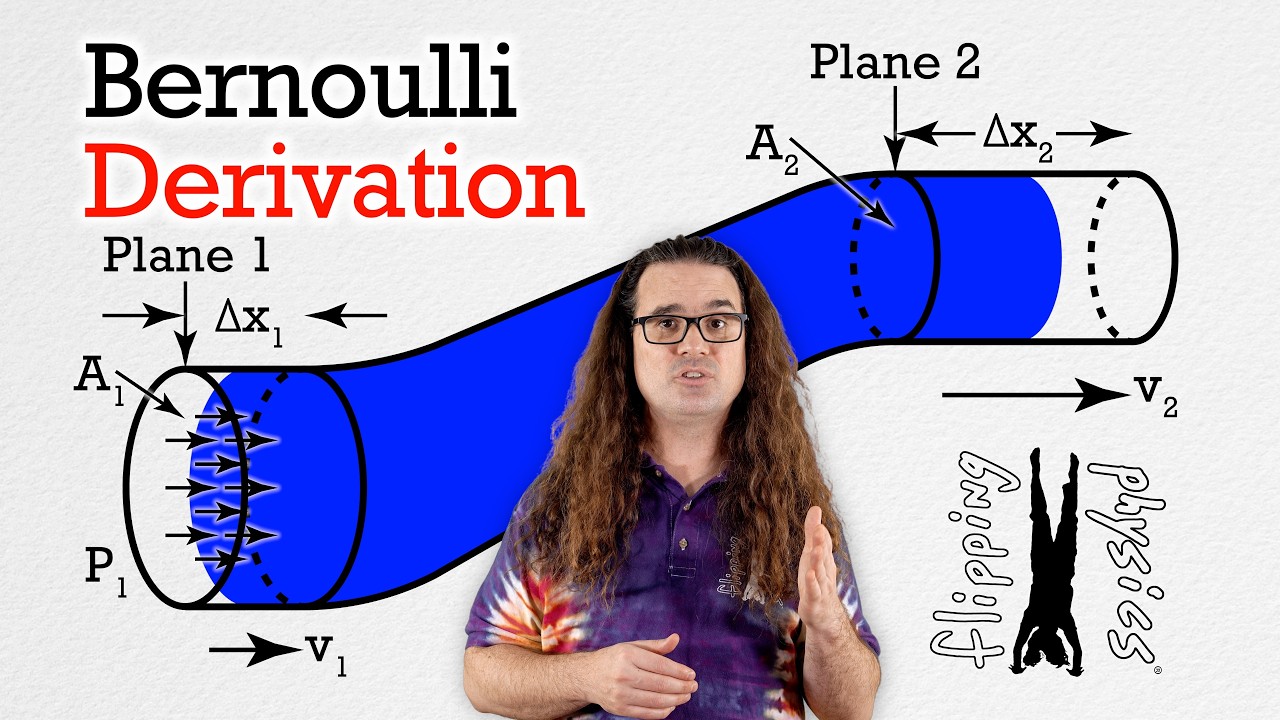
- 😀 The final form of Bernoulli’s equation is: P + 1/2 ρv² + ρgz = constant, where P is pressure, ρ is fluid density, v is velocity, g is gravitational acceleration, and z is height.
- 😀 Bernoulli’s equation reflects the conservation of energy along a streamline, where the sum of pressure energy, kinetic energy, and potential energy is constant.
- 😀 The equation implies that an increase in the speed of a fluid results in a decrease in pressure or potential energy, and vice versa.
- 😀 The lecture also introduces fluid dynamics (forces causing movement in fluids), contrasting it with fluid statics (fluids at rest).
- 😀 Assumptions in Bernoulli’s equation simplify the real-world complexity of fluid behavior by ignoring viscosity and assuming constant density.
- 😀 The lecture lays the foundation for applying Bernoulli’s equation to solve practical problems in fluid mechanics, such as gliders and air flow, to be covered in future sessions.
Q & A
What is the main focus of the lecture in terms of fluid mechanics?
-The main focus of the lecture is the derivation and understanding of Bernoulli's equation, which relates the pressure, velocity, and height of a fluid along a streamline.
Who is Daniel Bernoulli, and why is his work significant in fluid mechanics?
-Daniel Bernoulli (1700-1782) was a Dutch-Swiss scientist whose work laid the foundation for the study of fluid dynamics. His publication *Hydrodynamica* in 1738 introduced what is now known as Bernoulli's equation, a critical concept in fluid mechanics.
What assumptions are made when deriving Bernoulli's equation?
-The assumptions made are that the fluid is incompressible, the flow is steady, and viscous effects are neglected (ideal fluid). This simplifies the complex behavior of fluids in dynamic flow.
How is Newton's second law applied in the derivation of Bernoulli's equation?
-Newton's second law is applied to a small fluid particle moving along a streamline. The rate of change of momentum is related to the forces acting on the particle, including gravitational and pressure forces. This leads to the formulation of Bernoulli's equation.
What types of forces are considered when deriving Bernoulli's equation?
-The forces considered are gravitational forces (weight of the fluid element) and pressure forces. Viscous forces are ignored, as the flow is assumed to be inviscid (no friction).
What is the significance of the term 'streamline' in the context of Bernoulli's equation?
-A streamline represents the path along which a fluid particle flows. The Bernoulli equation applies to fluid moving along these streamlines, where the pressure, velocity, and height are related.
What is the final form of Bernoulli's equation?
-The final form of Bernoulli's equation is: P + ½ρv² + ρgz = constant, where P is the pressure, ρ is the density, v is the velocity, g is gravitational acceleration, and z is the height above a reference point.
Why is Bernoulli's equation important in fluid mechanics?
-Bernoulli's equation is important because it provides a way to understand and predict the behavior of fluid flow. It shows how pressure, velocity, and height are interconnected and is used in a wide range of applications, such as aviation, hydraulics, and pipe flow analysis.
What is the role of viscosity in the Bernoulli equation, and why is it neglected in its derivation?
-Viscosity is the internal friction of the fluid, but it is neglected in the derivation of Bernoulli's equation because the equation assumes an ideal, inviscid fluid. This simplifies the analysis and focuses on the effects of pressure and gravity on fluid motion.
How does the concept of 'incompressibility' affect the use of Bernoulli's equation?
-Incompressibility means that the fluid density remains constant during flow. This assumption is critical for the validity of Bernoulli's equation, as it allows the equation to relate pressure, velocity, and height without needing to account for changes in fluid density.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级5.0 / 5 (0 votes)