Tanx, Secx, Cscx, Cotx & Their Derivatives
Summary
TLDRIn this informative video, the host derives the derivatives of tangent and secant functions while also introducing the derivatives of cosecant and cotangent. By using the quotient rule and trig identities, viewers learn to navigate through complex derivatives, solidifying their understanding of trigonometric functions. The video emphasizes important patterns for memorization and provides practice problems for application. It culminates with finding the equation of the tangent line to the curve of y = tan(x) at a specified point, reinforcing the practical aspects of calculus. This engaging lesson is designed for students eager to enhance their derivative skills.
Takeaways
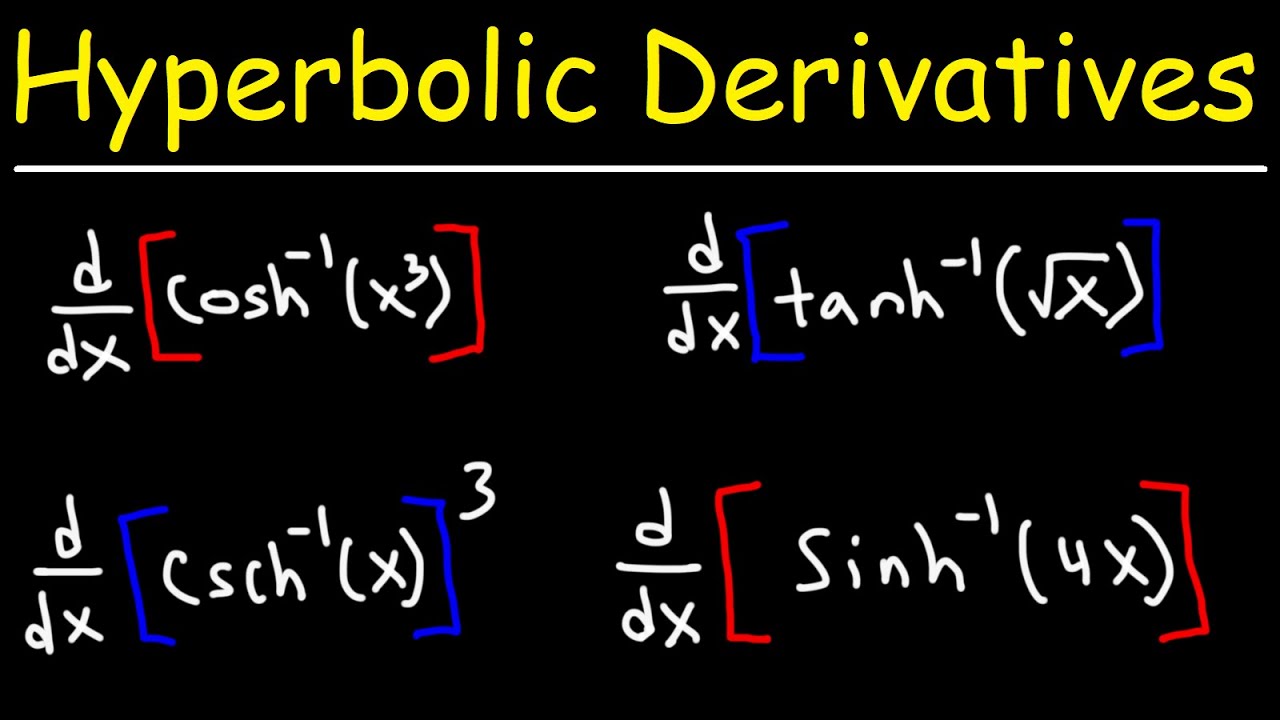
- 😀 The video focuses on deriving the derivatives of tan x and sec x, as well as introducing cosecant x and cotangent x.
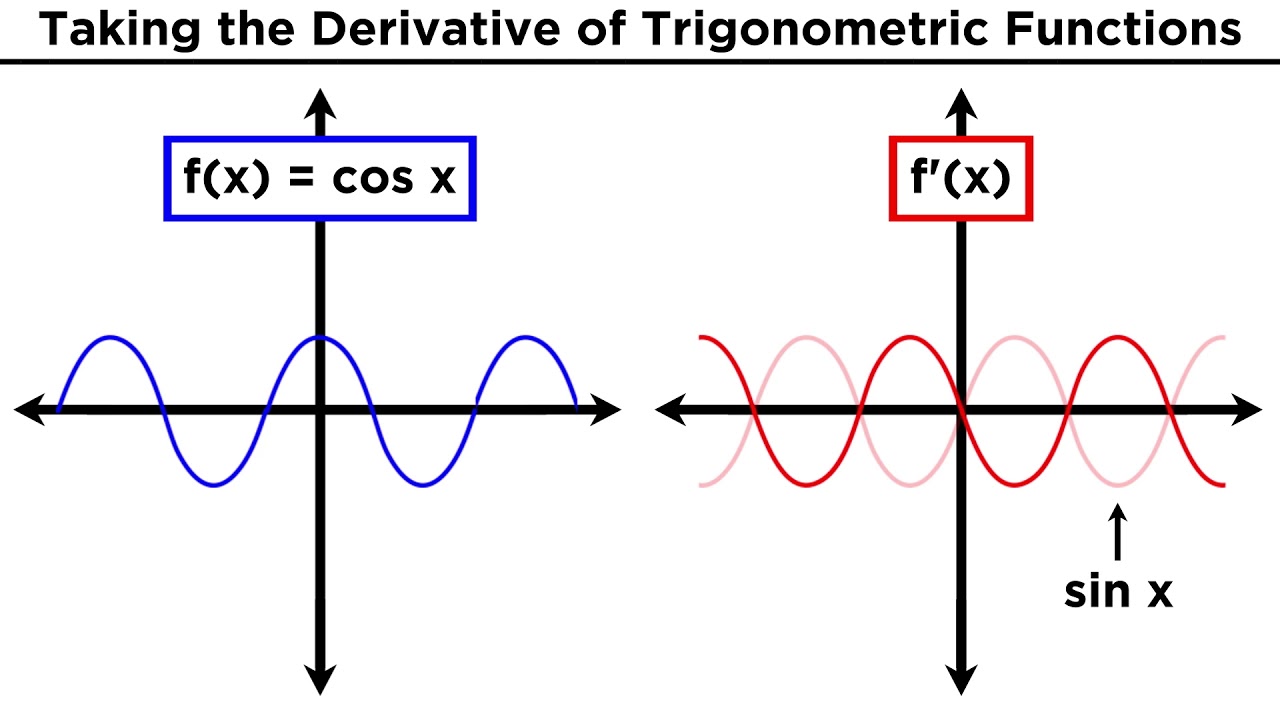
- 📚 Review of basic derivatives: the derivative of sin x is cos x, and the derivative of cos x is -sin x.
- 📏 The quotient rule is essential for deriving tan x, as it is expressed as sin x / cos x.
- ✍️ The derivative of tan x is derived to be sec² x using the quotient rule and the Pythagorean identity.
- 🔍 The derivative of sec x is sec x tan x, derived through a similar process of expressing it as 1/cos x.
- 🔄 Patterns in derivatives: tan x and sec x always appear together in differentiation.
- 🔠 Trig functions that start with 'c' (cosecant and cotangent) have negative derivatives, mirroring the negative derivative of cos x.
- 💡 Practice problems are provided to reinforce understanding of trig derivatives, demonstrating application of the quotient rule.
- 📏 A specific example finds the tangent line to y = tan x at x = π/4, using the derived derivative to find the slope.
- 👍 Viewers are encouraged to like and subscribe to the channel for more lessons on derivatives and other mathematical concepts.
Q & A
What are the derivatives of tan(x) and sec(x) as explained in the video?
-The derivative of tan(x) is sec^2(x) and the derivative of sec(x) is sec(x)tan(x).
Why is the quotient rule necessary for deriving tan(x) and sec(x)?
-The quotient rule is necessary because tan(x) can be expressed as sin(x)/cos(x), which is a quotient of two functions.
What are the derivatives of the remaining trigonometric functions mentioned?
-The derivative of cosecant(x) is -cosec(x)cot(x) and the derivative of cotangent(x) is -cosec^2(x).
How can one derive the formula for the derivative of tan(x)?
-To derive the formula for the derivative of tan(x), use the quotient rule on sin(x)/cos(x), simplifying to sec^2(x) by applying the Pythagorean identity.
What is the significance of the Pythagorean identity in this context?
-The Pythagorean identity states that sin^2(x) + cos^2(x) = 1, which helps simplify the numerator in the derivative calculation of tan(x).
What patterns were identified to remember the derivatives of trigonometric functions?
-A key pattern is that derivatives of tan(x) and sec(x) appear together, and derivatives of cosecant(x) and cotangent(x) also pair together with negative signs.
What is the structure set up before applying the quotient rule?
-The structure involves defining U as the numerator and V as the denominator, clearly identifying their derivatives, U' and V', before applying the quotient rule.
How do you find the derivative of the function f(x) = sec(x) * cosec(x)?
-To find the derivative, apply the product rule by calculating the derivatives of sec(x) and cosec(x) and combining them using the product rule formula.
What is the equation of the tangent line to the curve y = tan(x) at x = π/4?
-The equation of the tangent line is y - 1 = 2(x - π/4), where the slope is derived from the derivative sec^2(π/4) which equals 2.
Why is it useful to express functions like sec(x) and tan(x) in different forms?
-Expressing functions in different forms, such as sec(x) as 1/cos(x) and tan(x) as sin(x)/cos(x), simplifies calculations and can reveal cancellations when deriving.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级5.0 / 5 (0 votes)