Finding factors of a number | Factors and multiples | Pre-Algebra | Khan Academy
Summary
TLDRThis script is a detailed explanation of finding all the factors of 120. It starts with the obvious factors, 1 and 120, and then systematically checks divisibility by 2, 3, 4, 5, 6, 8, 10, and other numbers up to 11. The video uses various divisibility rules, such as checking the sum of digits for 3 and ignoring the last digit for 4, to determine factors. It also demonstrates how to use long division to confirm divisibility. The factors found are 1, 2, 3, 4, 5, 6, 8, 10, 12, 15, 20, 24, 30, 40, 60, and 120, providing a comprehensive list of all numbers that 120 is divisible by.
Takeaways
- 🔢 The number 120 is divisible by 1 and itself, with 1 being the smallest factor and 120 being the largest.
- 👉 120 is an even number, hence divisible by 2, with 60 being the corresponding factor when divided by 2.
- 📐 The divisibility rule for 3 is satisfied if the sum of the digits is divisible by 3, and 120 meets this criterion with factors 3 and 40.
- 📘 To check divisibility by 4, look at the last two digits; since 20 is divisible by 4, 120 is too, with 30 being the factor.
- 💯 A number ending in 0 or 5 is divisible by 5, confirming 5 and 24 as factors of 120.
- 🔄 Being divisible by both 2 and 3 confirms divisibility by 6, resulting in factors 6 and 20.
- ❌ The number 7 does not divide evenly into 120, as shown by the long division process.
- 🔢 Divisibility by 8 is confirmed by the long division process, with 15 being the factor.
- ❌ The sum of the digits of 120 is 3, which is divisible by 3 but not by 9, thus 120 is not divisible by 9.
- 🔄 A number ending in 0 is divisible by 10, making 10 and 12 factors of 120.
- ❌ 11 does not divide evenly into 120, leaving a remainder, thus 11 is not a factor.
Q & A
What is the smallest factor of 120?
-The smallest factor of 120 is 1, as every whole number is divisible by 1.
What is the largest factor of 120?
-The largest factor of 120 is 120 itself, as no number larger than 120 can divide into 120 without a remainder.
Why is 2 a factor of 120?
-2 is a factor of 120 because 120 is an even number, and all even numbers are divisible by 2.
How can you determine if a number is divisible by 3?
-A number is divisible by 3 if the sum of its digits is divisible by 3. For 120, 1+2+0=3, which is divisible by 3, so 120 is divisible by 3.
What is the divisibility rule for 4?
-A number is divisible by 4 if the number formed by its last two digits is divisible by 4. For 120, the last two digits are 20, which is divisible by 4.
Why is 5 a factor of 120?
-5 is a factor of 120 because it ends with a 0 or a 5, which means it is divisible by 5.
What is the significance of the number 6 in relation to the factors of 120?
-6 is a factor of 120 because it is divisible by both 2 and 3, and 120 is divisible by both of these numbers.
Why does the process of finding factors stop at 11 for the number 120?
-The process stops at 11 because the square root of 120 is between 10 and 11, and factors come in pairs. Since 12 is already a factor, there are no factors greater than 11 that have not been accounted for.
How can you quickly determine the factors of 120 using the divisibility rules mentioned in the script?
-You can quickly determine the factors of 120 by testing divisibility using the rules for 2 (even number), 3 (sum of digits divisible by 3), 4 (last two digits divisible by 4), 5 (ends in 0 or 5), and 6 (divisible by both 2 and 3). For other numbers, you can perform division or use the divisibility rules specific to those numbers.
What is the complete list of factors for the number 120?
-The complete list of factors for 120 is 1, 2, 3, 4, 5, 6, 8, 10, 12, 15, 20, 24, 30, 40, 60, and 120.
Why is 7 not a factor of 120?
-7 is not a factor of 120 because when you divide 120 by 7, you get a remainder, indicating that 7 does not divide evenly into 120.
What is the significance of the number 8 as a factor of 120?
-8 is a factor of 120 because when you divide 120 by 8, you get 15 with no remainder, indicating that 8 divides evenly into 120.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

Mencari akar-akar dari Bilangan Kompleks

🗣️SEBENTAR LAGI PUASA‼️ YUK PAHAMI HAL-HAL YANG MEMBATALKAN PUASA❗ | BUYA YAHYA
Vector Equations of Lines - Mathematics T STPM Semester 1

Basic Concepts of Economics - Needs, Wants, Demand, Supply, Market, Utility, Price, Value, GDP, GNP
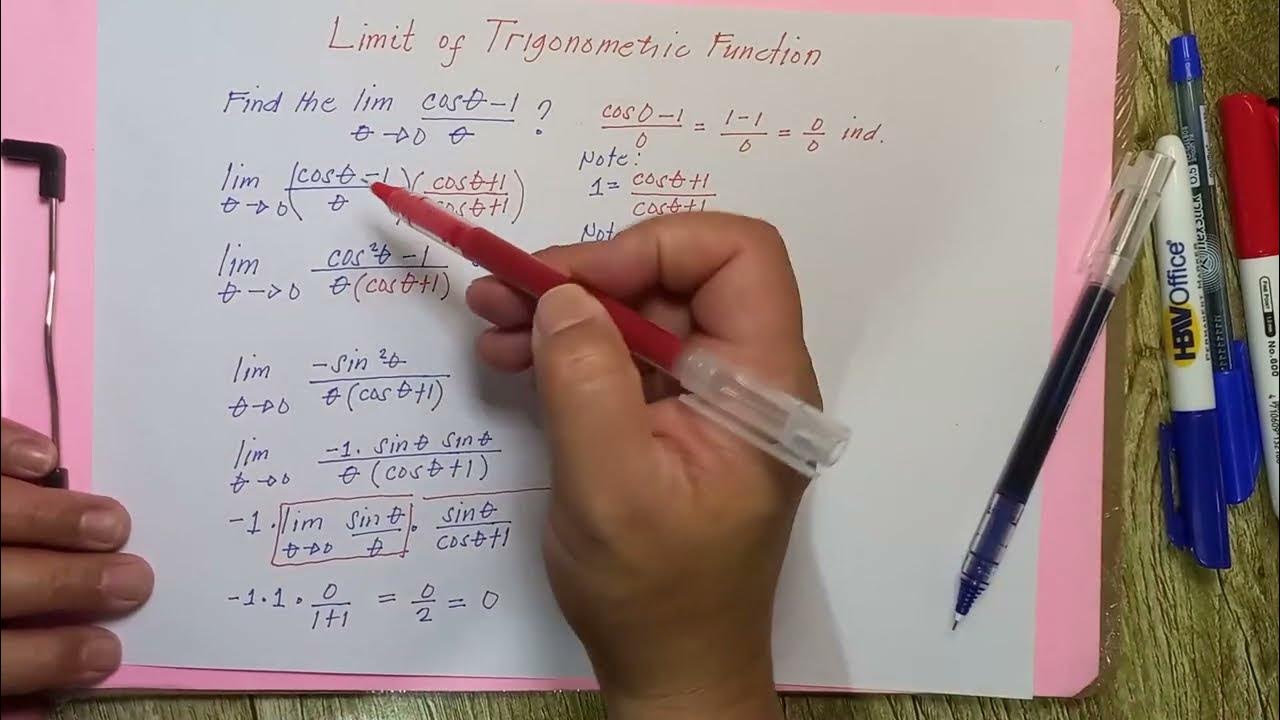
Limit of cosØ-1 over Ø as Ø approaches 0 is 0 I Special limit of Trigonometric Functions

{Unique} Fact Overlay And Video Effects | Transition Overlay
5.0 / 5 (0 votes)
