Limit of cosØ-1 over Ø as Ø approaches 0 is 0 I Special limit of Trigonometric Functions
Summary
TLDRThis video script delves into the process of finding the limit of a trigonometric function as Theta approaches zero. It explores the indeterminate form issue when directly substituting zero, then employs the conjugation method to rationalize the expression. The script explains the use of trigonometric identities, specifically the Pythagorean identity, to simplify the problem. It also covers the limit of a product involving sine and tangent functions, illustrating the transformation of tangent into sine and cosine, and the application of limit theorems to reach the conclusion that the limit is one. The detailed explanation aims to guide viewers through the intricacies of limit calculations in trigonometry.
Takeaways
- 📚 The focus of the lesson is to find the limit of a trigonometric function, specifically cosine Theta minus 1 divided by Theta as Theta approaches zero.
- 🚫 Direct substitution results in an indeterminate form (0/0), which indicates that the limit does not exist without further analysis.
- 🔍 The method of conjugation is introduced to rationalize the expression, which involves multiplying the numerator and denominator by the conjugate of the numerator.
- 🔢 The Pythagorean trigonometric identity is used, where sin squared Theta plus cosine squared Theta equals 1, to simplify the expression.
- 🔄 The process involves transmuting the expression to involve sine squared Theta, which is equivalent to 1 minus cosine squared Theta, to facilitate simplification.
- 📉 The limit of sine squared Theta over Theta as Theta approaches zero is used, which is a key step in solving the limit.
- 🔑 The limit of Theta over sine Theta as Theta approaches zero is identified as a crucial theorem, which states that this limit is equal to one.
- 📌 Factoring is used to simplify the expression, allowing for the separation of terms and the application of limits to each factor individually.
- 🎯 The final result of the limit is determined to be zero after applying the above methods and simplifications.
- 📝 The script also discusses another limit involving the product of Theta and sine Theta over the square of tangent Theta as Theta approaches zero, which is transformed into sine squared Theta over cosine squared Theta.
- 🧩 The final limit of the second problem is found to be one, after simplifying and applying the limit of the ratio of Theta to sine Theta as Theta approaches zero.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is finding the limit of a trigonometric function, specifically the limit of (cosine Theta - 1) / Theta as Theta approaches zero.
Why is direct substitution not applicable when Theta approaches zero in the given problem?
-Direct substitution is not applicable because it results in an indeterminate form of 0/0, which means the limit cannot be determined by simply substituting Theta with zero.
What method is suggested to resolve the indeterminate form of 0/0 in the problem?
-The method of conjugation or rationalization is suggested to resolve the indeterminate form. This involves multiplying the numerator and the denominator by the conjugate of the numerator.
What is the conjugate of the numerator in the given problem?
-The conjugate of the numerator, which is cosine Theta - 1, is cosine Theta + 1.
How does the Pythagorean trigonometric identity help in solving the problem?
-The Pythagorean trigonometric identity, which states that sin^2(Theta) + cos^2(Theta) = 1, helps in transforming the expression into a form that can be simplified and factored to find the limit.
What is the significance of the limit of sin(Theta)/Theta as Theta approaches zero in the script?
-The limit of sin(Theta)/Theta as Theta approaches zero is significant because it is equal to 1. This fact is used to simplify the expression and find the limit of the given trigonometric function.
What is the final result of the limit of (cosine Theta - 1) / Theta as Theta approaches zero?
-The final result of the limit of (cosine Theta - 1) / Theta as Theta approaches zero is zero.
What is the second problem discussed in the script related to the limit of a trigonometric function?
-The second problem discussed is finding the limit of (Theta * sin(Theta)) / (tangent(Theta)^2) as Theta approaches zero.
How is the tangent function in the second problem converted to sine and cosine functions?
-The tangent function is converted by expressing it as the sine function squared over the cosine function squared, i.e., sin^2(Theta) / cos^2(Theta).
What is the final result of the limit of (Theta * sin(Theta)) / (tangent(Theta)^2) as Theta approaches zero?
-The final result of the limit of (Theta * sin(Theta)) / (tangent(Theta)^2) as Theta approaches zero is one.
Why is it important to rationalize the expression in the script?
-Rationalizing the expression is important to eliminate the complex or indeterminate forms, allowing for a clearer path to finding the limit of the given trigonometric function.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Limite de una función real de variable real
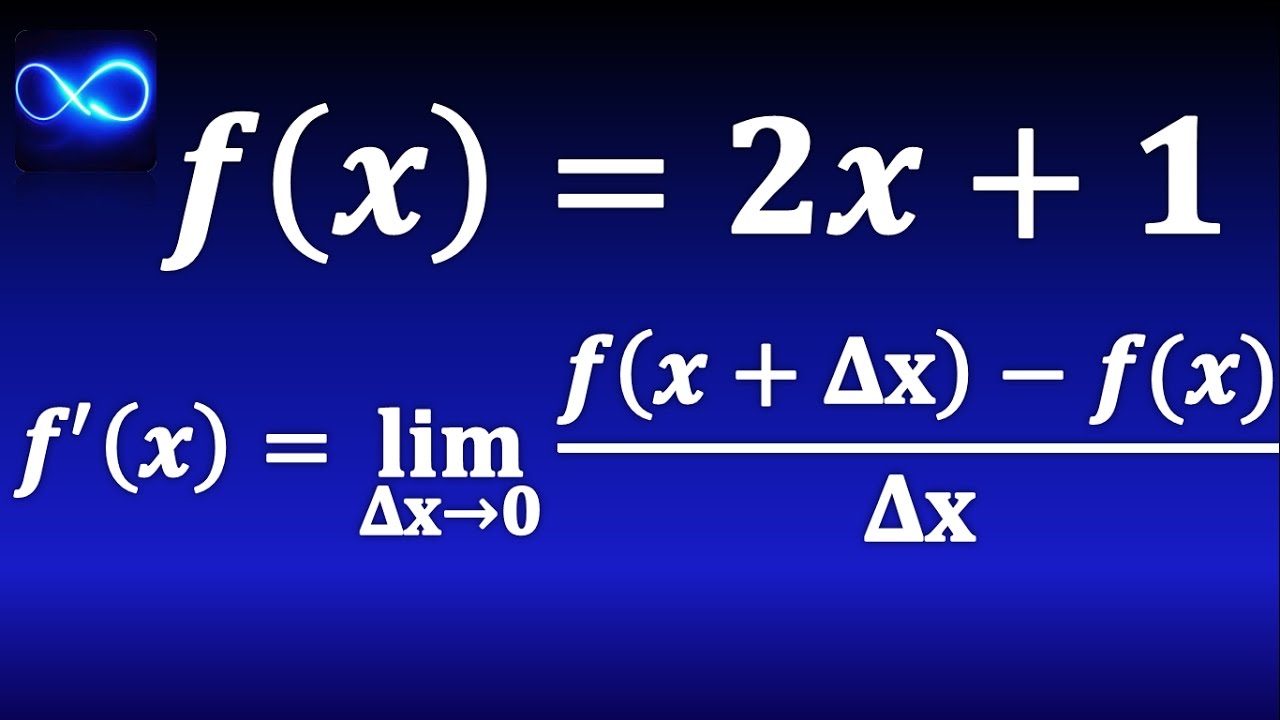
Derivative by increment method (By definition with limit)

Calculating slope of tangent line using derivative definition | Differential Calculus | Khan Academy
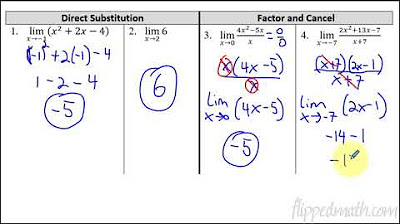
Calculus AB/BC – 1.6 Determining Limits Using Algebraic Manipulation

Derivative definition

3 Step CONTINUITY Test | Piecewise Functions | Differential Calculus | Tagalog/Filipino
5.0 / 5 (0 votes)