Trapezoidal Rule: Basic Form

Summary

The script discusses the importance of integration in calculus, starting with the concept of areas under curves. It highlights Riemann's sum and the close relationship between integration and differentiation. The instructor explains why we need alternative methods like the trapezoidal rule and Simpson's rule for functions that are difficult to integrate directly. The trapezoidal rule is introduced as a way to approximate areas under curves by dividing the area into trapezoids, emphasizing its dependency on the function's nature for accuracy.

Takeaways

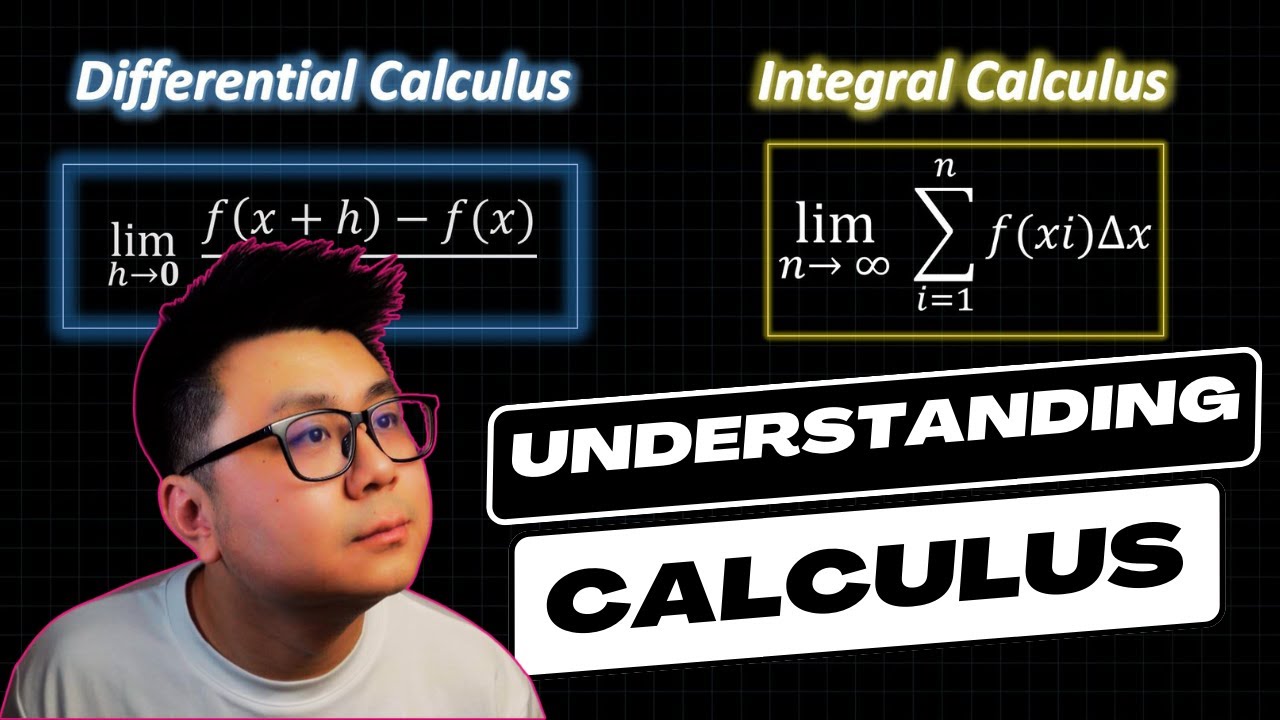
• 📚 The main topic discussed is integration, specifically the calculation of areas under curves, which is foundational to calculus.
• 👨‍🎓 Riemann's method of using sums and limits is highlighted as a clever and efficient approach to integration, closely related to differentiation.
• 🔍 The script introduces the need for alternative methods of integration when dealing with functions that are difficult to integrate directly or when the function's rule is unknown.
• 📈 The trapezoidal rule is introduced as a method for approximating areas under curves, especially useful when exact integration is challenging.
• 📐 The trapezoidal rule is likened to the Riemann sum, but using trapezoids instead of rectangles to improve the approximation of areas.
• 📝 The formula for the area of a trapezoid is given as the average of the two parallel sides multiplied by the height, which in the context of integration is the integral from a to b of f(x)dx.
• 🔢 The importance of understanding function values, specifically f(a) and f(b), is emphasized for calculating the heights of the trapezoids in the approximation.
• 🔄 The script explains that the accuracy of the trapezoidal rule is highly dependent on the nature of the function being integrated.
• 🔼 To improve the approximation, the script suggests using more trapezoids, effectively increasing the number of function evaluations and reducing the error.
• 📚 The lecture also mentions Simpson's rule as another method for integration that will be covered, indicating a progression from simpler to more complex techniques.

Q & A

What is the main topic being discussed in the script?

The main topic being discussed is integration, specifically focusing on methods to calculate areas under curves, and the introduction of the trapezoidal rule as an approximation technique.

Why is the Riemann sum and the concept of limits important in the context of this discussion?

The Riemann sum and the concept of limits are important because they form the foundation of the integration process, which is central to calculating areas under curves. They provide a method to approximate these areas using sums, which is essential when dealing with functions that are difficult to integrate directly.

What is the trapezoidal rule and how does it relate to the Riemann sum?

The trapezoidal rule is a method for approximating the definite integral of a function by dividing the area under the curve into trapezoids instead of rectangles. It relates to the Riemann sum in that it's an extension of the idea of approximating areas by using simpler shapes, but it improves upon the Riemann sum by using trapezoids which can provide a better approximation.

Why might one need to use the trapezoidal rule instead of direct integration?

One might need to use the trapezoidal rule instead of direct integration when dealing with functions that are difficult to integrate directly or when the exact form of the function is unknown, but measurements of the curve are available.

What is the formula for calculating the area of a single trapezium as described in the script?

The formula for calculating the area of a single trapezium is given by \( \frac{1}{2} \times (f(a) + f(b)) \times (b - a) \), where \( f(a) \) and \( f(b) \) are the function values at points \( a \) and \( b \), and \( b - a \) is the width of the trapezium.

How does the number of trapeziums used affect the accuracy of the approximation?

The more trapeziums used, the closer the approximation becomes to the actual area under the curve. This is because increasing the number of trapeziums reduces the error in the approximation, making the overall estimate more accurate.

What is the significance of the term 'function values' in the context of the trapezoidal rule?

In the context of the trapezoidal rule, 'function values' refer to the values of the function at specific points, which are used as the heights of the trapeziums. These values are crucial for calculating the area of each trapezium and thus the total area under the curve.

Why is it important to know the function values when using the trapezoidal rule?

Knowing the function values is important because they determine the heights of the trapeziums used in the approximation. Without these values, one cannot construct the trapeziums or calculate their areas accurately.

What is the potential drawback of using the trapezoidal rule for certain functions?

The potential drawback of using the trapezoidal rule is that it can provide a poor approximation for functions with rapidly changing slopes or curves that are not well-represented by trapezoidal shapes, leading to significant errors in the calculated area.

What is the advantage of using the trapezoidal rule over other integration methods in certain situations?

The advantage of using the trapezoidal rule is its simplicity and applicability when the exact form of the function is unknown or difficult to integrate. It allows for the estimation of areas under curves using only the function values at specific points.

Outlines

00:00

📐 Introduction to Integration and the Trapezoidal Rule

The script begins by discussing the concept of integration, specifically the calculation of areas under curves. It mentions the historical development by Riemann, who used the idea of sums and limits to create calculus. The speaker emphasizes the precision and speed of this method, questioning why one would revert to less accurate and slower methods. Two reasons are given for learning alternative methods: first, the existence of functions that are difficult to integrate, and second, situations where the function itself is unknown, only measurements are available. The speaker introduces the trapezoidal rule as a method for approximating areas under curves, explaining that it is an improvement over the Riemann sum using rectangles. The process involves creating a trapezium by taking the tops of two function values and calculating its area using the average of the bases and the height, which corresponds to the horizontal distance between the function values.

05:00

📏 Understanding the Trapezoidal Rule in Detail

This paragraph delves deeper into the trapezoidal rule, explaining how to calculate the area of a trapezium using the average of the two parallel sides (function values at points a and b) and the perpendicular height (the horizontal distance between a and b). The speaker clarifies terminology, such as 'function values,' which are the outputs of the function at specific points. The paragraph highlights that the trapezoidal rule's effectiveness depends on the type of function being analyzed. The speaker suggests that using more trapeziums can improve the approximation's accuracy, reducing the error. The process is generalized for multiple trapeziums, indicating that increasing the number of trapeziums results in a closer approximation to the actual area under the curve.

Keywords

💡Integration

Integration in the context of the video refers to the process of finding the area under a curve, which is a fundamental concept in calculus. It is mentioned as being closely related to differentiation and is achieved through methods like the Riemann sum. The video discusses integration as a fast and precise method for calculating these areas, which is why it is central to the topic being discussed.

💡Riemann Sum

The Riemann Sum is a technique used in calculus to approximate the area under a curve by dividing it into rectangles. The video credits Riemann for his clever idea that contributed to the development of integration. It is used as a basis for the Trapezoidal Rule, which is a method for approximating areas under curves that the video aims to teach.

💡Differentiation

Differentiation is a mathematical operation that is the inverse of integration and is used to find the rate at which a function changes. In the video, differentiation is mentioned in contrast to integration, highlighting that while it's easy to differentiate certain functions, integrating them can be more challenging, which justifies the need for alternative methods like the Trapezoidal Rule.

💡Trapezoidal Rule

The Trapezoidal Rule is a numerical method for estimating the definite integral of a function. It is introduced in the video as an alternative to integration when the function is difficult to integrate directly. The rule works by approximating the area under a curve with trapezoids instead of rectangles, which can provide a better approximation.

💡Function Values

Function values refer to the output of a function for a given input. In the context of the video, function values are crucial for calculating the heights of the trapezoids in the Trapezoidal Rule. The video emphasizes the importance of understanding function values, as they are used to determine the 'heights' of the trapezoids that approximate the area under the curve.

💡Approximation

Approximation in the video refers to the process of estimating a value or quantity that is not exactly known. The Trapezoidal Rule is an example of an approximation method used when exact integration is difficult. The video discusses how increasing the number of trapezoids can improve the accuracy of the approximation.

💡Primitive

A primitive of a function is another term for its antiderivative, which is used in integration to find the original function from its derivative. The video mentions that if one knows the primitive of a function, they can easily find the area under the curve by evaluating it at certain bounds. However, the video also points out that sometimes finding the primitive is not feasible, which is where the Trapezoidal Rule comes into play.

💡Area Under a Curve

The area under a curve is a central theme of the video, referring to the space enclosed by the curve and the x-axis. The video discusses various methods for calculating this area, emphasizing the importance of integration and the Trapezoidal Rule as tools for this purpose. The area under a curve is a fundamental concept in calculus with applications in various fields.

💡Numerical Methods

Numerical methods are mathematical techniques used to find approximate solutions to mathematical problems, especially where exact solutions are difficult or impossible to obtain. The Trapezoidal Rule is an example of a numerical method discussed in the video for approximating the area under a curve when integration is not straightforward.

💡Integration by Parts

Integration by Parts is a specialized technique in calculus for integrating products of functions. The video briefly mentions it as a 'fancy method' that can be used to integrate certain functions that are not easily integrable by basic methods. It is introduced to highlight the complexity of some integrations and the need for advanced techniques.

💡Simpson's Rule

Simpson's Rule is another numerical method for approximating the definite integral of a function, which is mentioned at the end of the video as a topic for further discussion. It is similar to the Trapezoidal Rule but uses parabolic approximations instead of trapezoids, potentially offering a more accurate approximation for certain types of functions.

Highlights

Integration is fundamentally about calculating areas under curves.

Riemann's sum and limits concept was pivotal in developing integration.

Integration is closely related to differentiation, offering a precise and efficient method.

The method's efficiency is why it remains the cornerstone of calculus.

The need for alternative methods arises when dealing with functions that are difficult to integrate.

Alternative integration methods become necessary when the function's rule is unknown.

The trapezoidal rule is introduced as a method for approximating areas under curves.

The trapezoidal rule is an improvement over the Riemann sum using rectangles.

A single trapezium can be used to approximate an area under a curve.

The area of a trapezium is calculated using the average of the bases and the height.

Function values at the endpoints are crucial for determining the heights of the trapezium.

The trapezoidal rule provides an approximation that can be improved by increasing the number of trapeziums.

The accuracy of the trapezoidal rule depends on the nature of the function being integrated.

Simpson's rule is mentioned as another method for approximating areas under curves.

The concept of function values is emphasized for its importance in calculating areas.

The trapezoidal rule is generalized for use with multiple trapeziums to improve approximation accuracy.

Transcripts

um

you will remember

this topic i've been calling it

integration right but really what it is

is um is areas under curves that's where

we started remember

and um

it just so happened that

riemann and his clever idea of riemann

sum and limits and calculus blah blah

blah blah blah worked out integration

this process was so close to

differentiation

was a really great way of doing it

it's not just great it's fast it's

precise you know um and that's why

because it's so good that's why we keep

using it like you know if you've got a

fast precise method it makes sense to

build your topic around that method okay

so being that we have a fast precise

method for calculating areas under

curves why would we go backwards

why would we come up with a method or

actually methods too um that are not

only less accurate

but as you'll discover most of the time

it's usually slower because

that is on integrated fireball okay now

two reasons number one um

we've so far been working with you know

functions where we know how to integrate

so

uh stuff like this

you know we can eat for breakfast yeah

right it's not that hard to think of

functions which we can differentiate but

we can't

integrate and you know it's not

complicated with example

suppose i wanted an area under this verb

now you've known this about this

function for months now it's not hard to

differentiate

how do you integrate and therefore find

the area underneath okay now at the

moment you guys can't

um

if you want to later on i'll teach you

how to integrate this function it

requires a fancy um a fancy method

really a fancier name called integration

by parts but the point is

like it's hard to work with okay but we

can still work out this area now firstly

what if my function is tough to

integrate secondly um what if i don't

even know what the function

is

like what if you've got

some measurements okay and what you want

is the area underneath okay but what

actually governs this

function you don't know what its rule is

okay you don't know what the x to the

power of this or whatever you've just

got measurements now think back when did

we learn how to deal with situations

like that yes questions you have

questions like this

and they're like here's a lake okay

we have we have these measurements

now work out what the area is okay so

yes

okay good question we'll get to i'm not

going to probably not send a cover today

but the short answer is

number one because you can it's not that

hard number two because it's useful like

you get blue shapes you can work out the

areas that's a handy skill

now anyway

this is why we're going to learn methods

for epoxy

we're going to learn two the first one

is called a trapezoidal rule

and um you might want to make that

subheading

the second one is called simpson's rule

but i anticipate we'll get to it

tomorrow actually i'm pretty sure

sorry

okay

so how does trapezoidal rule work um

suppose i want to find an area like this

so did we learn um

welcome to the present robbie okay now

if i want to find out what this area is

trapezoidal rule looks very similar to

the whole idea of the riemann sum which

is chopping up an area into you know

shape which i don't know intershapes

that do know okay now what did you use

for the testimony of right hand

rectangles right and when you turn it

into an integral then you just have a

whole button okay but it doesn't take

that much to realize that you can do

better than a rectangle

by using a trapezium okay so let's just

take the simplest case from a single

trapezium right if this is the area that

i'm after okay so

that's what i want

okay

i can make a trapezium that will be at

least in the right ballpark as this by

taking the tops of the two function

values okay and just drawing them

there's my trapezium

okay

now it's important to note that uh you

know these are vertical so that's a

right angle there right so how i go

about working uh calculating sorry the

area of this trapezium what is the area

of entropy

like if i had

the average of the bases

and then you take the average of the two

parallel sides okay now you might notice

i've deliberately not called them a and

b even though that's what we'd normally

call them why

yeah

because my a b and my lower bounds okay

so that's why i just

watch out so

n plus n

you add them and then taking on two

gives you the average and then you

multiply

so this is a standard way right now what

do each of these things correspond to in

our diagram where's the perpendicular

height of this trapezium

that horizontal distance in there okay

now in this case it's b minus a

it's a

which equals a

that's a more surprising result than i

thought it was okay so this b was a

right now what are these two parallel

sides equal to

well that's x equals a and that's x

equals b

and this is f of x okay what's the

height of the first one

and the second one is

now these are important um make a note

of this

we need to introduce in terminology the

quicker idea of the better because we'll

start to get confused later

um these guys f of a and f of b

right because i get these heights right

or you know bases if you like by

evaluating the function taking these

values and putting them into the

function okay we call them function

values

okay

the questions will refer to certain

numbers of function values so it's

important you know what they are

right now now that i've got my pieces

what's the area so i can say number one

the area is equal to the integral from a

to b

of

f

okay

but

i'm now going to sorry not equal to i'm

going to say that's approximately equal

to this trapezium that i've just

manufactured okay so it's this

perpendicular height on two so

b minus a whole one with two okay

and then i'm going to take uh the

average of the i don't know if it will

retain the image i'm just going to add

up the two

okay so you see normally we would have

said at this point you know what's

actually equal to if you know what the

primitive is

then you evaluate at your upper lower

bounds but the point is i don't know

what the primitive is or i can't be

bothered to calculate it so you can see

if we're going from here to here number

one is approximate number two i just

need to know what the original function

is this way

now as you can probably imagine um this

trapezium that we've just manufactured

might be a good approximation or it

might be a lousy approximation right so

if i give you a function like say

i don't know let's think of one um

i draw one down here something like this

okay and suppose i want to find its area

from say there's my a

um up to here to b right well the

trapezium you make out of this is like

five times the size of the area that i'm

actually after okay

trapezoidal rule um highly dependent on

you know what kind of function you are

as to how close you get right

but

i can still use trapeziums in a

situation like this to get a pretty good

approximation what would i do instead

i would i would just do the same thing

riemann did which is have more of them

okay so if i put energy in here

and so on the more trapeziums i get

pcr

the more shapes i get

the closer i'll get the less this um

this error area will be

okay so how do i generalize draw

yourself another graph um just like this

one

and when

now i'm going to go from this is kind of

like basic trapezoidal rule one

trapezium

two function values what happens if we

have more so