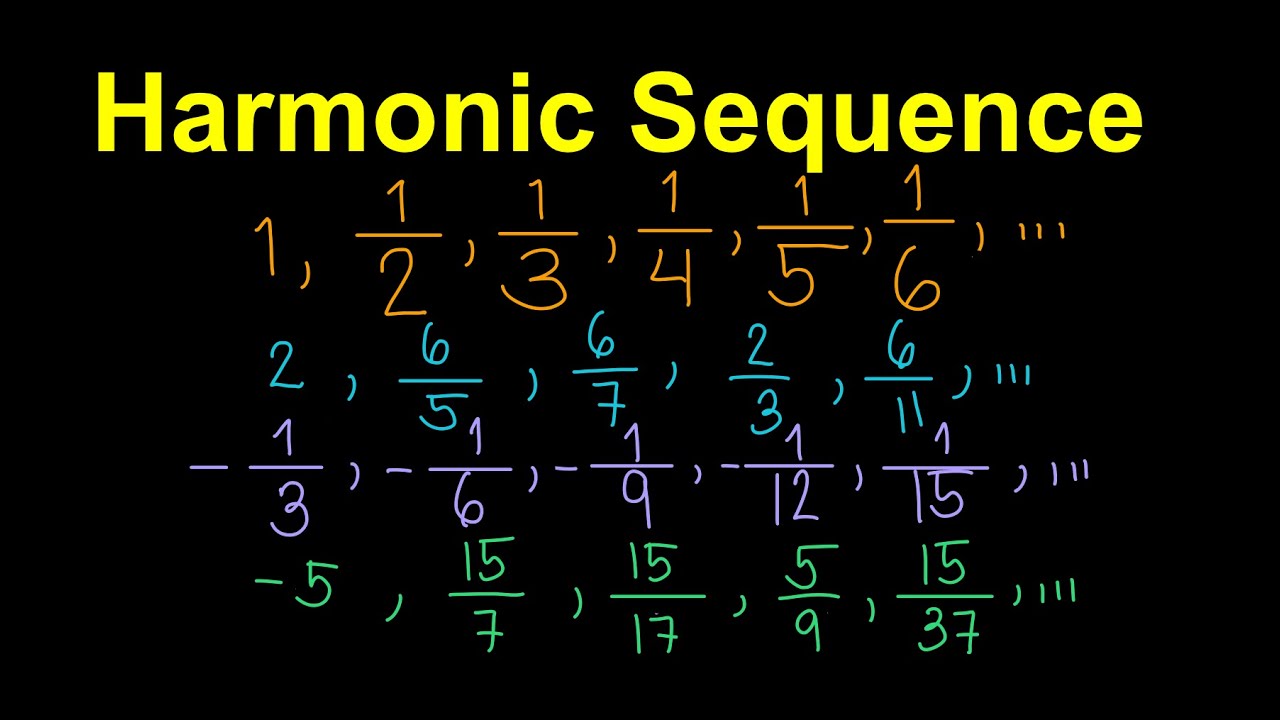
HARMONIC SEQUENCE | GRADE 10 MATHEMATICS Q1

Summary

This video tutorial delves into the concept of harmonic sequences, exploring their connection to music and mathematics. It explains how harmonic sequences are derived from the reciprocals of arithmetic sequences, using examples of guitar strings to illustrate the point. The video teaches viewers how to find the nth term of a harmonic sequence by reversing the arithmetic sequence formula. Practical examples, including calculating specific terms of harmonic sequences, are provided to solidify understanding. The guide encourages engagement by prompting viewers to apply these concepts to solve related mathematical problems.

Takeaways

• 🎵 The term 'harmonic' is commonly associated with musical sounds and is used in both math and music.
• 📏 In music, harmonics are tones at frequencies that are multiples of the fundamental frequency, such as the second harmonic being twice the fundamental frequency.
• 🎸 On a guitar, the length of the string is proportional to the number of vibrations per second, which affects the sound's harmony.
• 🔢 A harmonic sequence in math is a sequence where the string lengths are in the proportion of x, x/2, x/3, x/4, and so on, producing a harmonious sound when played.
• ♫ The reciprocal of a harmonic sequence forms an arithmetic sequence, where the difference between consecutive terms is constant.
• 🔄 The formula for the nth term of a harmonic sequence is the reciprocal of the formula for the nth term of an arithmetic sequence.
• 📐 To find the next terms of a harmonic sequence, first determine the common difference of the corresponding arithmetic sequence and then apply the harmonic formula.
• 🎼 Examples in the script demonstrate how to find the next terms and specific terms of harmonic sequences by using arithmetic sequences.
• 📉 The process involves identifying the first term and common difference of the arithmetic sequence, then using these to find terms in the harmonic sequence.
• 📚 The script provides a comprehensive guide to understanding the relationship between harmonic and arithmetic sequences and how to calculate terms within them.

Q & A

What is the relationship between harmonic and arithmetic sequences?

A harmonic sequence is the reciprocal of an arithmetic sequence. If the reciprocals of a sequence form an arithmetic sequence, then the original sequence is called a harmonic sequence.

What is the formula for finding the nth term of a harmonic sequence?

The nth term of a harmonic sequence is given by the formula \( \frac{1}{a_1 + (n - 1)d} \), where \( a_1 \) is the first term and \( d \) is the common difference of the corresponding arithmetic sequence.

How does the length of a string on a musical instrument like a guitar relate to harmonics?

The length of a string on a guitar is proportional to the number of vibrations per second, which affects the harmonics produced. A set of strings with lengths proportional to each other produces a harmonious sound.

What is a second harmonic in the context of music?

A second harmonic in music is a tone that has a frequency twice that of the fundamental frequency.

Can you provide an example of how to find the next term in an arithmetic sequence given the first few terms?

To find the next term in an arithmetic sequence, first determine the common difference by subtracting the first term from the second term. Then, add this difference to the last term provided to find the next term.

How do you find the harmonic sequence given an arithmetic sequence?

To find the harmonic sequence from an arithmetic sequence, take the reciprocal of each term in the arithmetic sequence.

What is the difference between an arithmetic and a harmonic sequence in terms of their formulas?

The formula for the nth term of an arithmetic sequence is \( a_n = a_1 + (n - 1)d \), while for a harmonic sequence, it is the reciprocal of this, \( \frac{1}{a_1 + (n - 1)d} \).

How can you determine the common difference of a sequence from the given terms?

To determine the common difference \( d \) of a sequence, subtract the preceding term from the current term, i.e., \( d = a_n - a_{n-1} \).

What is the significance of the reciprocal in the context of harmonic sequences?

In the context of harmonic sequences, the reciprocal of each term forms an arithmetic sequence, which is used to identify and work with harmonic sequences.

Can you explain how to find the nth term of an arithmetic sequence using the formula?

To find the nth term of an arithmetic sequence, use the formula \( a_n = a_1 + (n - 1)d \), where \( a_1 \) is the first term and \( d \) is the common difference.

Outlines

00:00

🎶 Harmonic Sequences and Musical Connections

This paragraph introduces the concept of harmonic sequences, primarily in the context of music and mathematics. It explains that harmonics in music refer to tones produced at frequencies that are multiples of the fundamental frequency. The paragraph uses the example of a guitar, where the length of the string is proportional to the frequency of vibrations, to illustrate how a set of strings with proportional lengths can produce harmonious sounds. It then defines a harmonic sequence as one where the reciprocals form an arithmetic sequence, and provides the formula for finding the nth term of a harmonic sequence, which is the reciprocal of the formula used for arithmetic sequences.

05:11

🔢 Arithmetic to Harmonic: A Mathematical Transformation

The second paragraph delves into the process of deriving a harmonic sequence from an arithmetic sequence. It guides through an activity where given an arithmetic sequence, one must find the next terms by identifying the common difference and applying it to the sequence. The reciprocal of each term in the arithmetic sequence forms the harmonic sequence. The paragraph provides examples of how to calculate the next terms in both sequences, emphasizing the reciprocal relationship between them. It also touches on methods for dealing with fractions, such as finding the least common denominator (LCD), to simplify the process.

10:11

📚 Practical Examples of Harmonic Sequences

This paragraph presents practical examples of finding terms in harmonic sequences. It demonstrates how to calculate the eighth term and the twenty-fifth term of a given harmonic sequence by first determining the corresponding arithmetic sequence and then applying the formula for the nth term of an arithmetic sequence. The process involves identifying the first term (a_sub_1), the common difference (d), and the term number (n), and then substituting these values into the formula to find the reciprocal, which gives the term in the harmonic sequence. The examples provide a clear step-by-step approach to solving such problems.

15:12

📢 Conclusion and Call to Action

The final paragraph serves as a conclusion to the video script, encouraging viewers to engage with the content by liking, subscribing, and enabling notifications for more video tutorials. It positions the channel as a guide for learning math lessons, suggesting that the content is educational and aimed at helping viewers understand mathematical concepts.

Keywords

💡Harmonic

In the context of the video, 'harmonic' refers to musical sounds and their mathematical relationships, particularly in the study of frequencies. Harmonics are overtones or partials that are whole number multiples of the fundamental frequency. For instance, the second harmonic is a frequency that is twice the fundamental frequency. In music, this concept is crucial for understanding how different notes and sounds are produced and how they interact to create harmonious melodies. The video uses the example of a guitar string to illustrate how the length of the string is proportional to the frequency of its vibrations, and thus, strings of different lengths produce harmonic sounds when played.

💡Fundamental Frequency

The 'fundamental frequency' is the lowest frequency of a periodic waveform, which in music, corresponds to the pitch of the note produced. In the video, it is mentioned in relation to harmonics, where harmonics are tones at frequencies that are integer multiples of the fundamental frequency. The fundamental frequency sets the base for the creation of overtones, which are essential for the richness and complexity of musical sounds.

💡Second Harmonic

The 'second harmonic' is a specific type of harmonic that is exactly twice the frequency of the fundamental frequency. It is one of the overtones that can be heard in addition to the main pitch of a note. The video explains that in the context of a guitar, the second harmonic is produced when the string is plucked or struck at its midpoint, causing it to vibrate at twice the rate of the fundamental frequency.

💡Harmonic Sequence

A 'harmonic sequence' is a sequence of numbers where each term is the reciprocal of an arithmetic sequence. In the video, the concept is introduced through the example of a guitar string, where the sequence of string lengths that produce harmonious sounds is x, x/2, x/3, x/4, and so on. The reciprocal of this sequence, which is 1, 2, 3, 4, forms an arithmetic sequence, illustrating the relationship between harmonic and arithmetic sequences.

💡Arithmetic Sequence

An 'arithmetic sequence' is a sequence of numbers with a constant difference between consecutive terms. The video explains that the reciprocals of a harmonic sequence form an arithmetic sequence. For example, if the harmonic sequence is 1/x, 1/(x/2), 1/(x/3), 1/(x/4), then taking the reciprocal gives the arithmetic sequence 1, 2, 3, 4. This relationship is key to understanding how harmonic sequences are derived from arithmetic ones.

💡Reciprocal

The 'reciprocal' of a number is 1 divided by that number. In the video, the concept of reciprocals is central to the relationship between harmonic and arithmetic sequences. By taking the reciprocal of each term in an arithmetic sequence, one can obtain a harmonic sequence, and vice versa. The video demonstrates this by showing how the reciprocal of the sequence 1, 1/2, 1/3, 1/4 is 1, 2, 3, 4, which is an arithmetic sequence.

💡Common Difference

The 'common difference' in an arithmetic sequence is the constant amount by which each term increases from the previous one. The video uses this concept to find the next terms in an arithmetic sequence, which is then used to determine the corresponding terms in a harmonic sequence. For example, if the common difference is 4, the next term after 13 in an arithmetic sequence would be 13 + 4 = 17.

💡Nth Term

The 'nth term' of a sequence refers to the term in a specific position within the sequence. The video discusses how to find the nth term of both arithmetic and harmonic sequences using formulas. For an arithmetic sequence, the nth term is found using the formula a_n = a_1 + (n - 1)d, where a_1 is the first term and d is the common difference. For a harmonic sequence, the nth term is the reciprocal of the nth term of the corresponding arithmetic sequence.

💡Formula

A 'formula' in mathematics is a concise way of expressing information symbolically. The video explains how formulas are used to calculate the nth term of arithmetic and harmonic sequences. The formula for the nth term of an arithmetic sequence is a_n = a_1 + (n - 1)d, and for a harmonic sequence, it is the reciprocal of this, 1/(a_1 + (n - 1)d). These formulas are essential tools for understanding and predicting sequences in mathematics.

💡Activity

In the context of the video, 'activity' refers to the practical exercises or examples provided to help viewers understand and apply the concepts being taught. The video includes activities where viewers are guided through finding the next terms of given arithmetic and harmonic sequences, which helps to reinforce the theoretical concepts with practical application.

Highlights

Harmonic sequence is often associated with musical sounds and is related to the frequency of tones.

The second harmonic is a tone with twice the frequency of the fundamental frequency.

Harmonic sequence in music is produced by strings whose lengths are proportional.

The sequence of string lengths in a guitar that produce a harmonious sound is x, x/2, x/3, x/4, and so on.

The harmonic sequence is the reciprocal of an arithmetic sequence.

The n-th term of a harmonic sequence is given by the reciprocal of the n-th term of an arithmetic sequence.

To find the next terms of an arithmetic sequence, subtract the first term from the second to find the common difference.

The harmonic sequence is derived by taking the reciprocal of each term in the arithmetic sequence.

The formula for the n-th term of a harmonic sequence is 1/(a_1 + (n-1)d), where a_1 is the first term and d is the common difference.

Examples are provided to demonstrate finding the next terms of a harmonic sequence given an arithmetic sequence.

The reciprocal of an arithmetic sequence is used to find the next terms of a harmonic sequence.

The common difference in an arithmetic sequence can be found by subtracting consecutive terms.

The formula for the nth term of an arithmetic sequence is used to find the nth term of a harmonic sequence by taking the reciprocal.

The eighth term of a harmonic sequence is found by applying the formula for the nth term of an arithmetic sequence.

The twenty-fifth term of a harmonic sequence is calculated using the arithmetic sequence formula and taking the reciprocal.

The video provides a comprehensive guide to understanding and finding terms in harmonic and arithmetic sequences.

Transcripts

[Music]

in this video we will discuss harmonic

sequence

so where do we usually heard the word

harmonic we usually heard this word

in math and in music the term harmonic

is often associated with musical sounds

and strange instruments a tone which is

twice the frequency of the fundamental

frequency

is called the second harmonic while a

tone which is

twice the frequency of the fundamental

frequency

is what we call the third harmonic

all right so example i have here a

guitar

in a guitar the length of the string is

proportional to the number of vibrations

of the string per second

but it's actually proportional to the

number of

vibrations of the string per second

so does a set of strings whose length

are proportional produces a harmonious

sound

if x is the length of the string of a

guitar

then the sequence of the lengths of the

string

is x x over two x over three

and x over four and so on or it produces

a sequence a harmonic sequence which

is one one half one third

and one fourth and so on so we have the

harmonic sequence one

one half one third and one fourth

now if we will be getting the reciprocal

of this harmonic sequence

we will have one two

three four and so on so when we say

reciprocal

um a tongue denominator nothing

numerator and then numerator

no need to write once adding denominator

all right

and uh if as you can see one two three

four is an example

of arithmetic sequence

so what do you think is the relationship

of these

two all right so let's define

harmonic sequence uh how does a

arithmetic

sequence connected to harmonic sequence

okay so it this is a sequence whose

reciprocals

form an arithmetic sequence which is

called a harmonic sequence so in other

words

an um army uh harmonic sequence

is just the reciprocal of the

arithmetic sequence so a four hostile

dance award no reciprocal

synaptic harmonic sequence this is just

the reciprocal of your arithmetic

sequence

all right so the n term of a harmonic

sequence is given by

so also we will be using the formula

in finding the nth term of the liquid

arithmetic sequence so

in finding the n term of the

arithmetic sequence we are using the

formula a sub 1

plus the quantity of n minus 1 times the

d or the common difference now since we

are getting the harmonic sequence

we will just use the reciprocal of the

formula of finding the n term of the

arithmetic sequence and that is

1 over a sub 1 plus the quantity

of n minus 1 times d which is your

common difference so as you can see

this is just the reciprocal of

the formula of finding the n term of

your arithmetic sequence so reciprocal

so this is the formula for harmonic

sequence

all right so

let's have first an activity so let's

see

if you can get the a harmonic sequence

given b given the arithmetic sequence

so i have here 1 5 9

13 okay so for us to find

the next terms of the given arithmetic

sequence

of course we have to look for d

or common difference

we have to subtract uh your first term

from

your second term or a sub 2 minus

a sub 1 okay so 5 minus 1 that is 4

so therefore our common difference or

jung di nathan

is equal to 4 so nothing

so second term minus your first term

all right so 13 so what do you think is

the next term so 13 plus 4 that is 17

okay let us apply the harmonic sequence

which is the reciprocal of your

arithmetic sequence so we will have

one okay since the reciprocal is one is

also one is still one okay and then one

over five

one over nine one over thirteen since

our next terminating detail is seventeen

so therefore our next term didn't do i

one over

seventeen so next we have six

two negative two negative six so for us

to find the

next term how uh what are you going to

find you have to look for the common

difference which is your d

so two minus six that is

negative four so the d or your common

difference is negative

four so therefore the next term here is

negative six

plus negative four that is negative

ten okay so the next term here is

negative ten

so let us get the harmonic sequence

so it's just the reciprocal so one over

six

one over two negative one over two

negative one over six now this since

this is negative ten so young next in

detail is

negative 1 over 10. next i have 7

15 over 2 8 17 over 2

and so on so how are we going to find

the common difference so again 15

over 2 minus seven so that is

one half but nothing one half because

say we will have

a 15 over two minus seven so you can

um unsha find the lcd and then subtract

okay so the common difference is one

half so hindi

nothing solution okay so one half

uncommon difference nothing

so therefore 17 over two plus one half

since similar fraction sila

so 17 plus 1 that is 18 and then copy

the common denominator

which is 2. so 18 divided by 2 therefore

um

next not indeed the next term nothing is

9 okay so let us

find the comma a harmonic sequence of

the given arithmetic sequence

so that is one over seven reciprocal

so remember um

and denominator so therefore a

reciprocal

is 2 over 15 and then this one since

this is 8 over 1 so 1 over 8

and then this one 2 over 17 so since i'm

next not in d

to i

so remember that your harmonic sequence

is

just the reciprocal of your arithmetic

sequence that's why these two are

related to

each other okay so let's have an example

find the next two terms of the harmonic

sequence

so you are asked to find the next two

terms of the harmonic sequence

so again for us to find the next terms

sequence

harmonic sequence is just a reciprocal

of arithmetic sequence

difference so 26 minus 30 that is

negative

4 so therefore 22 plus negative 4

that is 18 and then 18

plus negative 4 that is 14

okay

18 that is 1 over 18 and reciprocal

14 that is 1 over 14. so find the next

two terms

the next two terms are one over eighteen

and one over

fourteen next so i have here four over

fifteen

two over nine four over twenty one one

over six

so you are asked to find the next two

terms so again

arithmetic sequence which is the

reciprocal so we will have 15 over 4

9 over 2 21 over 4

since this is 1 over six so six nalang

sha

okay so nothing find the common

difference

so nine over two minus fifteen

over four okay so

it's not end so

actually when you are solving fractions

you can make use of

the lcd or the butterfly method okay

so um parameters

9 times 4 that is 36

and then this one 15 times 2 that is 30

so

36 minus 30 that is 6

and then 2 times 4 that is 8. so

multiply neutral

multiplying multiplied so this is 36

minus 30 that is 6

over 2 times 4 that is 8. so

6 over 8 that

is three-fourths pagner just nothing six

over eight that is three-fourths

so therefore and the common difference

nito i three fourths omega attack and

three fourths are six

so six plus three-fourths

six times four that is twenty-four

and then three times one divide my

pattern one taijan

so three times one so 24 plus 3 times 1

3 so 24 plus 3 that is 27

and then 1 times 4 that is 4 so 27 over

4. so this

is 27 over 4. next

italian and 20's and 3 27 over four

now since similar fraction

the numerator that is 27 plus 30

at plus 3 that is 30 and then copy

the common denominator which is 4 so 30

over 4 but the pinch

reduce the lowest term so that is 15

over 2.

so therefore this is 15 over 2. now

this is the arithmetic sequence

27 and 2 over 15.

let's have another example so find the

eighth term of the harmonic sequence

so i have here one-half one-fourth one

over six and so on

so first get the arithmetic the

reciprocal or the arithmetic sequence of

the given or harmonic sequence

and that is 2 4 6. so again when we are

getting the

arithmetic sequence we are just getting

its reciprocal

okay so let us identify a sub 1 since we

are getting the eighth term so masha

dershong malayo

we can apply the formula uh the formula

for the

arithmetic finding the n term of

arithmetic sequence so since harmonic

sequence it or reciprocal

so a sub 1 over a sub 1 is 2 our

d the common difference is 2 has a 4

minus 2 that is 2

and then our n since 8 terms so our n is

8.

now let us apply the formula of finding

the nth term of our harmonic sequence

so again this is the formula in finding

the n term for arithmetic sequence

now since harmonic

okay so let us substitute the values so

we will have 1 over

your a sub 1 is 2 plus your n is 8 and

then minus

one your common difference is two so

eight minus one that is seven

seven times two is fourteen fourteen

plus two so we will have one over

sixteen so therefore

the eighth term is one over sixteen so

eighth term nothing is one over sixteen

next

find the twenty-fifth term of this

harmonic sequence so i have one fourth

one over fourteen one over twenty four

and so on

so we will have so nate reciprocal that

is four

fourteen twenty four so we are looking

for the twenty-fifth

term so let us identify first a sub one

which is your fourth so your first term

d the common difference is 10 y

10 because 14 minus 4 that is 10

and then your n is 25 since we are

looking for the 25th term

okay um by uh you're

applying the formula so we have 1 over a

sub 1 plus the quantity of n

minus 1 times the common difference so

substitute the values we have one

your a sub 1 is 4 your n is 25

minus 1 your common difference is 10. so

25 minus 1 that is 24 times 10

that is 240 plus 4

so we will have 1 over 244 so

therefore our 25th term

is 1 over 244

hope you learned something don't forget

to like subscribe and hit the

notification bell for

updated ko for more video tutorials this

is your guide in learning your math

lessons

your walmart channel