Introduction to Sequence I Señor Pablo TV
Summary
TLDRThis tutorial introduces sequences to grade 10 students, covering arithmetic, geometric, harmonic, and Fibonacci sequences. It defines a sequence as an ordered set of numbers following a pattern or rule, and demonstrates how to identify and generate terms in various sequences, including examples of arithmetic progression and geometric progression.
Takeaways
- 📚 A sequence is an ordered set of numbers following a specific pattern or rule.
- 🔢 The terms of a sequence are denoted as a_1, a_2, a_3, ..., representing the first, second, third, and subsequent terms.
- 🔑 The script introduces four types of sequences: arithmetic, geometric, harmonic, and Fibonacci.
- 📈 An arithmetic sequence follows a pattern where each term is a multiple of a constant difference.
- ➗ A geometric sequence has a pattern where each term is a multiple of a constant ratio.
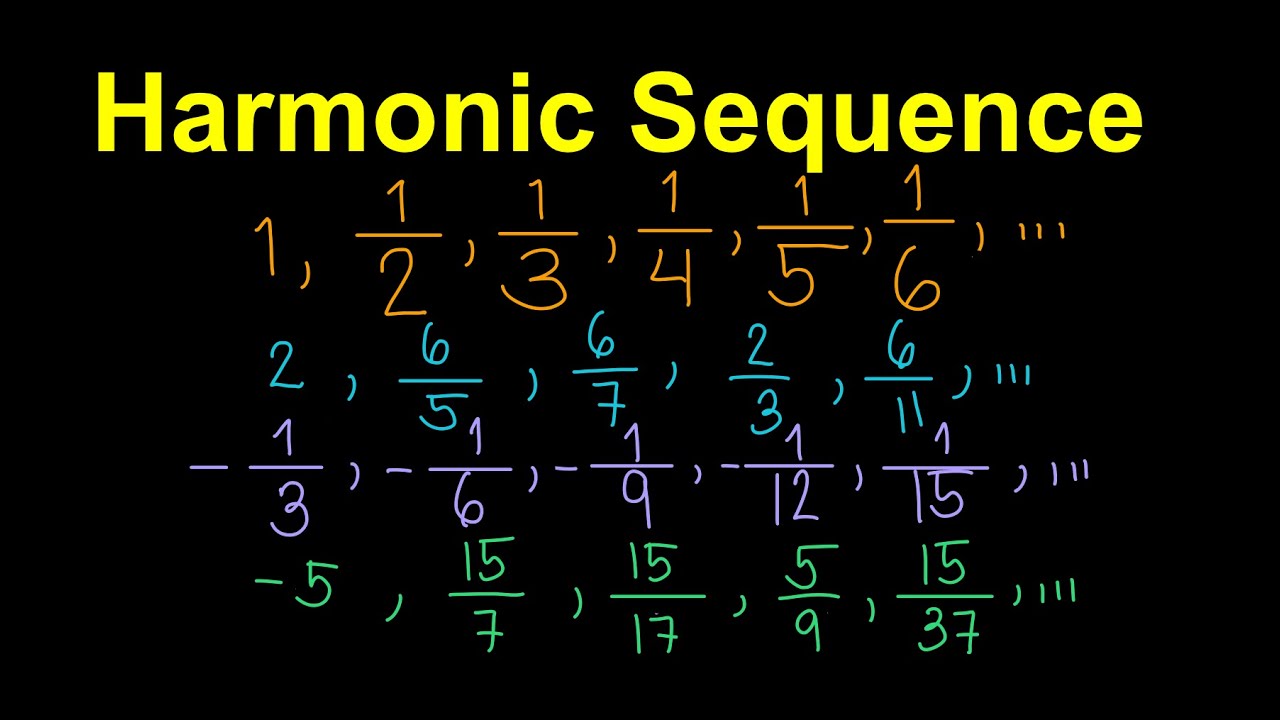
- 🎵 A harmonic sequence is a special type of sequence not detailed in the script, but typically involves reciprocals.
- 🌱 The Fibonacci sequence is a series where each term is the sum of the two preceding terms, starting from 0 and 1.
- 📝 The script provides examples to illustrate how to identify the pattern in a sequence and predict subsequent terms.
- 📉 The script also explains how to find specific terms in a sequence, given a formula, such as f(n) = 1/(2n).
- 🔢 The script demonstrates the process of finding terms in a sequence defined by a formula, like b_n = 2n - 4.
- 📚 The final takeaway is an introduction to the concept of a series, which will be discussed in a subsequent video.
- 👨🏫 The tutorial is presented by Senior Pablo TV, aiming to educate viewers on the basics of sequences.
Q & A
What is a sequence?
-A sequence is an ordered set of numbers that follow a specific pattern or rule.
How is the first term of a sequence denoted?
-The first term of a sequence is denoted as a_1.
What is the pattern in the sequence 0, 3, 6, 9, 12?
-The pattern in this sequence is the multiples of three.
What are the next two terms in the sequence 0, 3, 6, 9, 12?
-The next two terms in the sequence are 15 and 18, following the pattern of multiples of three.
What is the rule for the sequence 11, 6, 1, -4, -9?
-The rule for this sequence is subtracting five from each term to get the succeeding term.
What is the next term after -9 in the sequence 11, 6, 1, -4, -9?
-The next term after -9 is -14, continuing the pattern of subtracting five.
What is the rule for the sequence 200, 100, 50, 25?
-The rule for this sequence is dividing each term by 2.
What are the next two terms in the sequence 200, 100, 50, 25?
-The next two terms in the sequence are 12.5 and 6.25, following the pattern of dividing by two.
What is the formula for generating the first five terms of the sequence defined by f(n) = 1/(2n)?
-The formula generates terms by substituting n into f(n), resulting in 1/2, 1/4, 1/6, 1/8, 1/10 for n = 1, 2, 3, 4, 5 respectively.
What is the seventh and tenth term of the sequence defined by b(n) = 2n - 4?
-The seventh term is 10 (when n = 7), and the tenth term is 16 (when n = 10) in the sequence defined by b(n).
What are the four types of sequences mentioned in the video?
-The four types of sequences mentioned are arithmetic sequence, geometric sequence, harmonic sequence, and Fibonacci sequence.
What is the next topic to be introduced after sequences?
-The next topic to be introduced is series.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Pre-Calculus - Introduction to Sequence

pola bilangan kelas 8 part 1 (mudah)

Pola Bilangan [Part 1] - Mengenal Pola Bilangan

SOLVING PROBLEMS INVOLVING SEQUENCES (TAGALOG VERSION) | MATH 10 | MELCS Q1 – W5 | TEACHER REIMAR

Pembahasan BARISAN DAN DERET (Aritmetika & Geometri) KELAS 11 | #MatematikAsik
Harmonic Sequence (Tagalog/Filipino Math)
5.0 / 5 (0 votes)