How to Divide Polynomials using Long Division - Polynomials
Summary
TLDRIn this educational video, Trigon demonstrates how to divide polynomials using long division. Starting with dividing 6x^2 - 2x - 28 by 2x + 4, Trigon explains the process step-by-step, emphasizing that it mirrors the long division method used for whole numbers. The video illustrates dividing the leading terms, multiplying, and subtracting to find the partial quotient, which in this case is 3x - 7. Trigon then solves another polynomial division problem, reinforcing the method with a new example. The video is designed to teach viewers the algebraic process in an accessible manner.
Takeaways
- 📚 The video tutorial focuses on dividing polynomials using long division, a method similar to dividing whole numbers but applied to algebraic expressions.
- 🔢 The first example involves dividing (6x^2 - 2x - 28) by (2x + 4), where the leading terms are divided first, followed by multiplication and subtraction steps.
- 👨🏫 The instructor emphasizes the importance of dividing the leading coefficients or terms of the dividend and divisor to find the partial quotient.
- 📉 After dividing, the instructor demonstrates how to multiply the entire divisor by the partial quotient and subtract this product from the original polynomial to find the remainder.
- 🔄 The process is repeated with the new polynomial (result after subtraction) to find subsequent terms of the quotient until the remainder is zero or less than the divisor.
- 📝 The quotient obtained from the division is (3x - 7), indicating that the division process has been correctly followed and the remainder is zero.
- 🔍 In a second problem, the method is applied to (3x^3 - 4x^2 - 7x - 5) divided by (3x - 2), showing the consistency of the long division approach.
- 📈 The tutorial highlights the need to bring down terms from the dividend when the current degree of the remainder is less than the degree of the divisor.
- 📌 The final quotient for the second problem is (x^2 + 2x - 1) with a remainder of (-7), which is expressed as -7/(3x - 2).
- 💡 The video concludes with a reminder to like, subscribe, and enable notifications for updates, encouraging viewer engagement with the channel.
Q & A
What is the main topic discussed in the video?
-The main topic discussed in the video is dividing polynomials using long division.
What is the first polynomial division problem presented in the video?
-The first polynomial division problem is 6x^2 - 2x - 28 divided by 2x + 4.
How does the video explain the process of polynomial long division?
-The video explains that polynomial long division is similar to the long division of whole numbers, but in algebraic form. It involves dividing the leading terms, multiplying, and then subtracting.
What is the partial quotient obtained after the first division step in the first problem?
-The partial quotient obtained after the first division step in the first problem is 3x.
What is the result of the multiplication of the partial quotient 3x by the divisor 2x + 4?
-The result of the multiplication of the partial quotient 3x by the divisor 2x + 4 is 6x^2 + 12x.
What is the remainder after the first subtraction step in the first problem?
-The remainder after the first subtraction step in the first problem is -14x - 28.
What is the final quotient obtained after solving the first polynomial division problem?
-The final quotient obtained after solving the first polynomial division problem is 3x - 7.
How does the video handle the remainder in the division process?
-The video brings down the remainder and continues the division process by dividing the leading term of the new polynomial by the leading term of the divisor, then multiplying and subtracting as before.
What is the second polynomial division problem presented in the video?
-The second polynomial division problem is 3x^3 - 4x^2 - 7x - 5 divided by 3x - 2.
What is the final quotient and remainder obtained after solving the second polynomial division problem?
-The final quotient obtained after solving the second polynomial division problem is x^2 + 2x, and the remainder is -7/(3x - 2).
What is the advice given by the video for those who are new to the channel?
-The video advises those who are new to the channel to like, subscribe, and hit the Bell button to be updated with the latest uploads.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频
TAGALOG: Division of Polynomials - Long Division and Synthetic Division #TeacherA
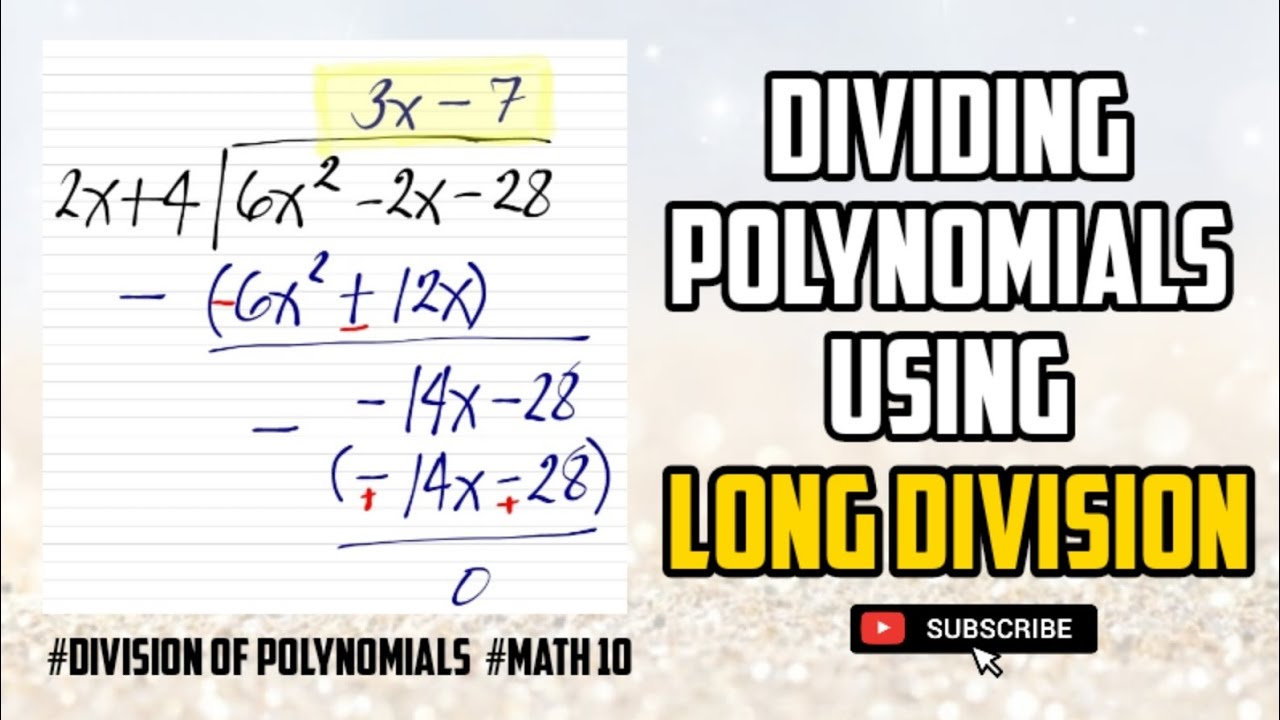
How to Divide Polynomials Using LONG DIVISION | Math 10
Polynomials - Adding, Subtracting, Multiplying and Dividing Algebraic Expressions

DIVISION OF POLYNOMIALS USING LONG DIVISION || GRADE 10 MATHEMATICS Q1
Synthetic Division of Polynomials

Integral de una división de polinomios (paso a paso)
5.0 / 5 (0 votes)
