Area of a Rectangle, Triangle, Circle & Sector, Trapezoid, Square, Parallelogram, Rhombus, Geometry

Summary

This instructional video script offers a comprehensive guide to calculating the areas of various geometric shapes, including rectangles, triangles, squares, circles, and more. It covers basic formulas for rectangles and triangles, introduces the concept of sectors and semi-circles in circles, and explains the area calculation for parallelograms, trapezoids, rhombuses, and scalene triangles. The script also touches on Heron's formula and the area of a circle with a known diameter or radius, providing a valuable resource for students of geometry.

Takeaways

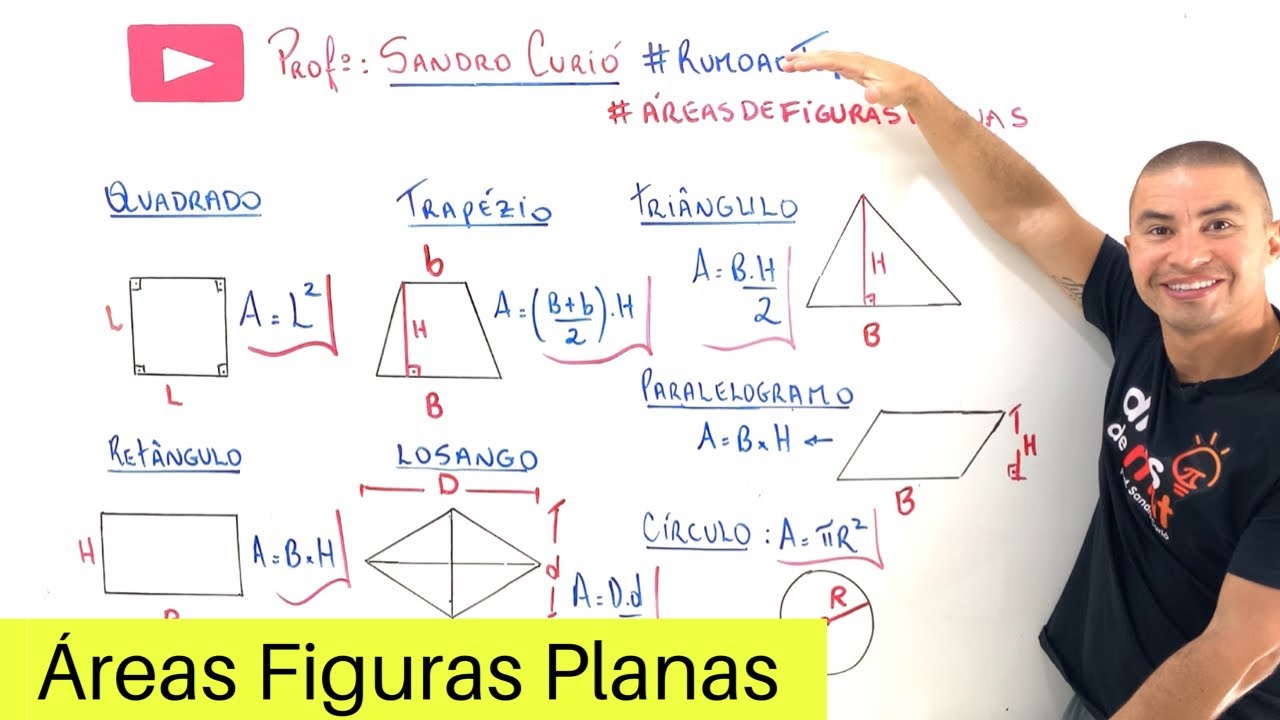
• 📏 The area of a rectangle is found by multiplying its length by its width.
• 🔺 The area of a right triangle is calculated as one-half times the base times the height.
• 📐 For a non-right triangle, the area can be found using the formula: \( \frac{1}{2} \times \text{base} \times \text{height} \), where the height is the perpendicular distance from the base.
• 🔶 The area of an equilateral triangle is given by the formula: \( \frac{\sqrt{3}}{4} \times s^2 \), where \( s \) is the length of a side.
• 🔳 The area of a square is the length of one side squared.
• ⭕ To find the area of a circle, use the formula: \( \pi \times r^2 \), where \( r \) is the radius.
• 📉 The area of a sector of a circle is the fraction of the circle's angle over 360 degrees, times the area of the whole circle.
• 🏞 The area of a parallelogram is the base times the height.
• 🔸 For a trapezoid, the area is calculated as the average of the two bases times the height: \( \frac{1}{2} \times (b1 + b2) \times h \).
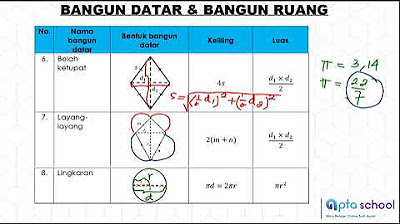
• 💠 The area of a rhombus is half the product of its diagonals: \( \frac{1}{2} \times d1 \times d2 \).
• 📐 Heron's formula can be used to find the area of a scalene triangle when all three sides are known: \( \sqrt{s(s-a)(s-b)(s-c)} \), where \( s \) is the semi-perimeter.
• 🔴 If given the diagonal of a square, the area can be found using the relationship between the diagonal and the side length in a 45-45-90 right triangle.
• 🌀 The area of a shaded region in a circle with a right triangle inside can be found by subtracting the area of the triangle from the area of the circle.

Q & A

What is the formula to calculate the area of a rectangle?

The area of a rectangle is calculated by multiplying the length by the width (Area = Length × Width).

If the base of a right triangle is 10 and the height is 8, what is the area?

The area of a right triangle is calculated as one half times the base times the height (Area = 1/2 × Base × Height). So, for a base of 10 and height of 8, the area is 40 square units.

How do you find the area of a triangle when you know the lengths of all three sides but it's not a right triangle?

You can use Heron's formula, which is the square root of s(s - a)(s - b)(s - c), where s is the semi-perimeter of the triangle (s = (a + b + c) / 2), and a, b, and c are the lengths of the sides.

What is the formula for the area of an equilateral triangle?

The area of an equilateral triangle is given by the formula (sqrt(3) / 4) × s², where s is the length of a side.

How is the area of a square calculated?

The area of a square is found by squaring the length of one of its sides (Area = Side × Side or Area = Side²).

What is the formula to find the area of a circle?

The area of a circle is calculated using the formula πr², where r is the radius of the circle.

If you have a sector of a circle with a 60-degree angle and a radius of 10, what is the area of the sector?

The area of a sector is found by taking the fraction of the circle the sector represents (in this case, 60/360) and multiplying it by the area of the entire circle (πr²). So, the area is (60/360) × π × 10² = 50π/3 square units.

What is the area of a semi-circle with a radius of 8?

The area of a semi-circle is half the area of a full circle, so it is (1/2)πr². With a radius of 8, the area is (1/2) × π × 8² = 32π square units.

How do you calculate the area of a parallelogram?

The area of a parallelogram is calculated by multiplying the base by the height (Area = Base × Height).

What is the formula for the area of a trapezoid?

The area of a trapezoid is found by taking the sum of the lengths of the two bases, multiplying by the height, and then dividing by two (Area = (Base1 + Base2) / 2 × Height).

How is the area of a rhombus determined?

The area of a rhombus is calculated by taking half the product of the lengths of the diagonals (Area = 1/2 × Diagonal1 × Diagonal2).

Outlines

00:00

📏 Rectangle and Triangle Area Calculations

This paragraph introduces the process of calculating the area of a rectangle given its length and width, using the formula (length × width). An example with dimensions 8 by 5 results in an area of 40 square units. It also covers the area of a right triangle, using the formula (1/2 × base × height), exemplified with a base of 10 and height of 8, yielding 40 square units. Additionally, it discusses the area of non-right triangles using the base and the sum of the other two sides as the height, and the area of an equilateral triangle using the formula (√3/4 × s²), where s is the side length.

05:02

🔺 Area of Geometric Shapes: Squares, Circles, and Sectors

The paragraph explains how to find the area of a square with a given side length, using the formula (side × side), and demonstrates this with a square of side length 9, resulting in an area of 81 square units. It then describes the area of a circle using the formula (π × r²), where r is the radius, and shows an example with a diameter of 10 centimeters, leading to an area of 25π square centimeters. Furthermore, it explains how to calculate the area of a sector of a circle given the angle in degrees, using the formula (angle/360) × π × r², illustrated with a 60-degree sector of a circle with a radius of 10, which gives an area of 50π/3 square centimeters.

10:03

📏 Advanced Area Calculations for Parallelograms and Trapezoids

This section delves into calculating the area of parallelograms and trapezoids. For parallelograms, the area is found using the formula (base × height), with an example given where the base is 8 and the height is 12, resulting in an area of 96 square units. It also addresses finding the area when the slant height and the sides of a right triangle are known, using the Pythagorean theorem to find the missing height. For trapezoids, the area is calculated with the formula (1/2 × (b1 + b2) × height), demonstrated with examples including a trapezoid with bases of 10 and 20 and a height of 8, yielding an area of 120 square units.

15:07

💠 Area Calculations for Rhombuses and Triangles Given Sides and Angles

The paragraph discusses the area of a rhombus using the formula (1/2 × d1 × d2), where d1 and d2 are the lengths of the diagonals, with an example of diagonals 10 and 12 resulting in an area of 60 square units. It also covers finding the area of a rhombus when the length of a side and half of a diagonal are known, using the Pythagorean theorem to find the lengths of the diagonals and then the area. Additionally, it explains how to calculate the area of a triangle when two sides and the included angle are known, using the formula (1/2 × a × b × sin(c)), where a and b are the sides and c is the included angle.

20:09

📐 Heron's Formula and Area Calculations Involving Circles and Triangles

This paragraph introduces Heron's formula for finding the area of a scalene triangle when all three sides are known, using the formula √(s(s-a)(s-b)(s-c)), where s is the semi-perimeter of the triangle. An example with sides 9, 10, and 11 is given, resulting in an area of 30√2 square units. It also explains how to find the area of a square when the length of the diagonal is known, using the Pythagorean theorem to find the side length and then squaring it to get the area. Lastly, it describes how to calculate the area of a shaded region inside a circle with a right triangle, using the formula πr² - (1/2 × base × height).

📉 Area Calculation for Shaded Region in a Circle

The final paragraph discusses a specific problem of finding the area of a shaded region in a circle, where the area is the difference between the area of the circle and the area of an inscribed right triangle. The area of the circle is given by πr², and the area of the triangle is calculated using the formula (1/2 × base × height), where the base and height are equal to the radius of the circle. An example with a radius of 8 units is provided, resulting in a shaded area of 32π - 64 square units.

Keywords

💡Area

Area is a fundamental geometric concept referring to the amount of space enclosed within a shape or a surface. In the context of the video, area is calculated for various shapes such as rectangles, triangles, and circles to demonstrate the different formulas used in geometry. For instance, the area of a rectangle is found by multiplying its length by its width, as shown with an 8 by 5 rectangle resulting in an area of 40 square units.

💡Rectangle

A rectangle is a quadrilateral with four right angles. It is one of the primary shapes discussed in the video for calculating area. The script explains that the area of a rectangle is determined by the product of its length and width, emphasizing the importance of units, such as square feet in the example given.

💡Triangle

A triangle is a polygon with three edges and three vertices. The video covers different types of triangles, including right triangles and equilateral triangles, each having distinct formulas for calculating their areas. For a right triangle, the area is one-half the product of its base and height, as illustrated with a base of 10 and a height of 8 yielding an area of 40 square units.

💡Right Triangle

A right triangle is a specific type of triangle that has one 90-degree angle. The script uses the right triangle to introduce the concept of calculating area with the formula one-half times base times height. It also demonstrates how to find the area of a triangle when given the lengths of two sides and the included angle, using the sine function.

💡Equilateral Triangle

An equilateral triangle is a special type of triangle where all three sides are of equal length. The video explains that the area of an equilateral triangle can be found using the formula (sqrt(3)/4) * s^2, where s is the length of a side. This formula is applied in the script with a triangle that has sides of length 10.

💡Square

A square is a special type of rectangle where all four sides are equal in length. The video mentions the square when discussing the area calculation, which is simply the side length squared. An example is given with a square with a side of 9, resulting in an area of 81 square units.

💡Circle

A circle is a shape where all points are equidistant from a central point, known as the center. The area of a circle is a key topic in the video, with the formula being pi * r^2, where r is the radius of the circle. The script also discusses calculating the area of a sector of a circle, which involves the central angle and the area of the entire circle.

💡Sector

A sector of a circle is the region enclosed by two radii and an arc. The video explains how to calculate the area of a sector by taking the fraction of the circle represented by the sector's central angle and multiplying it by the area of the entire circle, using the formula (theta/360) * pi * r^2, where theta is the angle in degrees.

💡Parallelogram

A parallelogram is a four-sided figure with opposite sides parallel. The area of a parallelogram is calculated as the product of its base and height. The video script provides examples of finding the area of a parallelogram, including situations where the height is found using the Pythagorean theorem.

💡Trapezoid

A trapezoid, also known as a trapezium in some regions, is a four-sided figure with at least one pair of parallel sides. The video discusses the area of a trapezoid, which is calculated as the average of the lengths of the two parallel sides (the bases) multiplied by the height. The script includes an example where the area is found by dividing the sum of the bases by two and then multiplying by the height.

💡Rhombus

A rhombus is a type of parallelogram where all four sides are of equal length. The area of a rhombus can be found by the formula one-half times the product of its diagonals. The video script explains this concept and provides an example where the diagonals are 10 and 12, resulting in an area calculation.

💡Heron's Formula

Heron's formula is a method for calculating the area of a triangle when all three side lengths are known. The video script introduces Heron's formula as sqrt(s*(s-a)*(s-b)*(s-c)), where s is the semi-perimeter of the triangle, and a, b, and c are the side lengths. An example is given with a triangle having sides of 9, 10, and 11, demonstrating the application of Heron's formula.

Highlights

Introduction to calculating the area of different shapes in geometry.

Explanation of the formula for the area of a rectangle: length times width.

Example calculation of a rectangle's area with dimensions 8 by 5.

Understanding the units of area, such as square feet.

Formula for the area of a right triangle: one half times base times height.

Calculation of a right triangle's area with base 10 and height 8.

Method for finding the area of a non-right triangle using base and height.

Example of calculating a non-right triangle's area with sides 9, 5, and 6.

Area calculation for an equilateral triangle using the formula involving the square root of three.

Formula for the area of a square: side squared.

Example of a square's area calculation with a side length of 9.

Area of a circle formula: pi times radius squared.

Calculation of a circle's area with a diameter of 10 centimeters.

Method for finding the area of a circle's sector given the angle and radius.

Example calculation of a sector's area with a 60-degree angle and radius of 10.

Area calculation for a semi-circle using half of pi times radius squared.

Explanation of the area formula for a parallelogram: base times height.

Calculation of a parallelogram's area with base 8 and height 12.

Method for finding the area of a parallelogram when given slant height and other dimensions.

Area calculation for a trapezoid using the formula involving the sum of bases and height.

Example of a trapezoid's area calculation with bases 10 and 20, and height 8.

Strategy for calculating the area of a trapezoid when given irregular dimensions.

Area formula for a rhombus: one half times the product of the diagonals.

Calculation of a rhombus's area with diagonals of lengths 10 and 12.

Method for finding the area of a rhombus when given one side and half of a diagonal.

Area calculation using Heron's formula for a triangle with sides 9, 10, and 11.

Strategy for finding the area of a square given the length of its diagonal.

Calculation of the area of a square with a diagonal of length 10 square root of 2.

Method for calculating the area of a shaded region in a circle with a right triangle.

Example calculation of the shaded area in a circle with a triangle side of length 8.

Transcripts

in this video we're going to focus on

finding the area

of different shapes that you encounter

in a typical geometry course

the first shape that we need to talk

about is the rectangle

let's say the rectangle has a length of

8

and a width of five

what is the area of the rectangle

the area is simply length times width

so in this example it's just eight times

five

which is

forty now you may need to

keep in mind the units

of the area let's say this is 8 feet by

5 feet

then the area is 40 square feet

now the next shape that we need to talk

about is the triangle

let's start with

a right triangle

so let's say if the base of the triangle

is 10

and the height is 8

what is the area of the triangle

the area

is one half

base times height

so we said ten is the base and the

height is eight

so this is going to be one half

ten times eight half of 10 is 5 and 5

times 8 is 40.

so it's 40 square units

now what if you have a triangle that

looks like this

let's say this is nine

and this is five and this is six

what's the area of the triangle

so for any right triangle you could use

the same formula it's one half base

times height in this example the height

is nine but the length of the base is

five plus six

the base of the triangle is this entire

length

which is eleven so it's going to be one

half

nine times eleven

nine times eleven is ninety-nine

and half of a hundred is fifty so half

of ninety-nine

is forty nine point five

now what if we have an equilateral

triangle

let's say all sides are 10.

what is the area of this triangle

for an equilateral triangle the equation

that you need

is the square root of three over four

times s squared

so it's root three over four

times ten squared

ten squared that's a hundred

and 100 divided by 4 is 25

so it's 25 root 3.

that's how you could find the area of an

equilateral triangle

now let's say if we have a square

and the left of the square is nine

what is the area of this figure

now for a square

all sides are the same

so the length and the width of the

square is nine

the area of a square is simply s squared

or side squared so it's going to be 9

squared

which is just 81.

now let's talk about the area of a

circle

so let's say if you're given the

diameter of the circle

and let's say the diameter of the circle

is 10 centimeters what is the area of

the circle

to find the area

it's equal to pi r squared

the radius r is one half of the diameter

so this is the diameter d

and this portion

is the radius of the circle

so the radius is half of 10

which is five so it's five centimeters

so the area is going to be pi

times five squared or simply 25 pi

square centimeters

now consider this problem

let's say we have a circle

and we want to find the area

of a sector of the circle

and let's say

the angle here is 60.

so we want to find the area

of this shaded region

and let's say the circle has a radius of

10.

what is the area

of the green region

just the sector

to find the area of a portion

of

a circle it's going to be

the angle in degrees divided by 360 so

that's the fraction of the circle

times the area of the entire circle

which is pi r squared

so the angle theta is 60.

so it's going to be 60 divided by 360

multiplied by pi times the radius

squared which the radius is 10.

now 60 divided by 360 what we can do is

cancel a zero

so we have six

divided by 36

and 10 squared is a hundred

now

36 is basically six times six

so we can get rid of one of the

six values

so we're left with a hundred pi

divided by six

and we could reduce the fraction

if we divide both numbers by two half of

a hundred is fifty

half of six is three

so it's fifty pi divided by three

so that's the area of the sector

and let's say if you have a semi-circle

and

let's say the radius of that circle

is eight

if you need to find the area of a

semi-circle

is one half

pi r squared because we have half a

circle

so it's one half pi

times eight squared

and eight squared that's 64. and half of

64 is 32. so in this example the area of

the semicircle is 32 pi square units

next up we have the area

of a parallelogram

let's say if the base is 8

and the height

is 12. what's the area of the

parallelogram

the area

is very similar to a rectangle it's base

times height

so it's 8 times 12.

and 8 times 12 is 96 so that's the area

of this particular

parallelogram

now let's see if we have another one

that

looks like this

let's say if

you're given

the slay height instead let's say the

slant height is five

and this section is

nine

but this part

let's say is three and this part is six

what is the area

of this parallelogram

in order to find the area you gotta find

the height first

and notice that we have a right triangle

the hypotenuse of the right triangle is

five

and one of the legs is three so we gotta

find the missing side

so we could use a squared plus b squared

is equal to c squared

so a is three

we're looking for the missing side b

and hypotenuse c is five

three squared is nine five squared is

twenty five

twenty five minus 9 is 16

and the square root of 16 is 4.

so the missing side or the height

is 4. so now we could use the formula

area as base times height

we have a base of 9 and a height of 4 so

nine times four is thirty six

next up we have the area of a trapezoid

so let's say the first base has a length

of ten

and the second one

20 and let's say the height of the

triangle i mean the trapezoid rather is

eight

find the area of this trapezoid

the area is one half

the sum of the two bases b one plus b

two

times the height

b1 is 10

and b2 the second base is 20 and the

height is 8.

so 10 plus 20 is 30.

and half of eight if we just multiply

these two

that's four so this becomes four times

thirty

which is

one twenty

so that's the area of

this particular trapezoid

now let me give you another problem

that's similar to this one

so let's say that

these two sides are 10

and let's say

this is 12

and this is 24.

find the area of the trapezoid

in order for us to do

that need to break it up into parts

so we need to make two right triangles

now keep in mind this entire length is

24.

which means

that

this is 12.

so this section has to be 6

and that has to be 6

so that it adds up to 24.

so now we can find the height of the

right triangle

so this is 10 this is 6. we got to find

the missing side

so using the same equation a squared

plus b squared is equal to c squared

let's say a is six

b is the height that we're looking for

and c is ten

six squared is thirty six

ten squared is one hundred

and a hundred minus thirty six is sixty

and the square root of 64 is eight

so the height is eight

now that we have the height

we can now find the area

so the area is going to be one half

b1 which is 12 plus b2 which is 24

times the height of eight

so 12 plus 24 that's 36

and half of 36 is 18.

so 18 times 8 let's break 18 into 10 and

8

and distribute 8.

8 times 10 is 80

8 times 8 is 64.

and 80 plus 64

is 144 so that's the area of this

particular trapezoid

now the next shape that we need to talk

about

is

the rhombus

so let's say the length of the first

diagonal

is

10

and the length of the second diagonal

is 12.

what is the area of this rhombus

by the way for a rhombus

all sides are congruent like a square

and the diagonals bisect each other at

90 degrees so those are some things to

keep in mind

the area of a diagonal i mean not a

diagonal but a rhombus is one half d1

times d2

so it's the product of the two diagonals

times one half so it's one half times

ten

times twelve

half of ten is five five times twelve is

sixteen

so that's the area of this particular

rhombus

now here's another problem

so let's say

the left of one of the sides

is 13

and this side is 5

where 5 is only half

of one of the diagonals

find the area of this particular rhombus

so let's focus on the right triangle

this is 5 this is 13.

it helps to know the special right

triangles for example

there's a 3 4 5 triangle

also if you multiply these numbers by 2

you can get the 6 8 10 triangle which we

covered

there is the 7 24 25 triangle

the 8 15 17 right triangle and also the

5 12 13 triangle

so we have two of these numbers 5 and

13. therefore the missing side must be

12.

now that we know what the missing side

is

you can also use the pythagorean theorem

to get 12 if you want to you can use a

squared plus b squared equals c squared

to get the same answer

but once we have the missing side

we now have the length of the two

diagonals

so diagonal one

is five and five these two sides are

equivalent

so

diagonal one has a length of ten

diagonal two

has a length of twelve plus twelve which

is twenty-four

so a is equal to one half d1 d2

so it's one half of 10

times 12

i mean not 12 but 24.

now half

of 24 is 12

and 12 times 10

is 120.

so the area of this particular rhombus

is 120 square units

now let's say if you have a triangle

where you're given two sides

and the included angle

what is the area of this particular

triangle

the area is one half a b

sine

c

so let's call this

angle a angle b

angle c

this is side c

side a

and side

b so in this case

a lowercase a is 12

and lowercase b or side b is 10.

and the angle between them that's angle

c that's 30.

half of twelve is six

and sine thirty is one half

half of ten is five and six times five

is thirty

so that's the area of this particular

triangle

so if you have two sides of a triangle

and the included angle

you can use this formula so this works

if you do not have a right triangle

how can we find the area

of a scalene triangle

let's say this is 9

10 and this is 11.

so this is not a right triangle and we

don't have any angles

so what can we do

to find the area of a triangle if we're

given all three sides

the first thing we need to do is find s

which is one half

of the perimeter of

the triangle

so it's going to be 9 plus 10 plus 11

divided by 2.

nine plus ten is nineteen nineteen plus

eleven is thirty

and thirty divided by two is fifteen

so that's the value of s

so now that we have the value of s

we can use huron's formula to find the

area

so it's going to be the square root

of s

times s minus a

times s minus b

times s minus c

s is 15

and then it's going to be 15 minus 9

times 15 minus 10

times 15 minus 11.

fifteen minus nine is six

fifteen minus ten is five

fifteen minus eleven is four

now fifteen

well let's see if we could simplify this

an easier way 15 can be broken into five

and three

and six

is three times two

i'm not going to change the five and

four i'm going to leave it alone

so basically

these two

can come out as a single five

these two can come out as a single three

and

the square root of four

is two so that comes out as a two so

left over with a two inside

five times three is thirty i mean not

thirty but fifteen

and fifteen times two is thirty

so the final answer is 30 square root 2.

so that's the area of the triangle

used in a heron's formula

let's say if we have a square

and we're given the length of the

diagonal

and let's say the length of that

diagonal is

10

square root 2.

what is the area of the square

now we know that the area is basically

side squared

all sides of the square are congruent

so therefore we could find this side

by using the pythagorean theorem if this

is s s and that's 10 root 2 we can solve

it

so using equation a squared plus b

squared is equal to c squared

a and b are both s in this example

and c is 10 root 2.

s squared plus s squared is 2 2s squared

10 squared is 100 and the square root of

2

squared which is like the square root of

2 times the square root of 2 that's the

square root of 4 which is 2.

so we can divide both sides by 2. so

therefore s squared is a hundred

and the square root of a hundred is ten

now the area is s squared and s squared

is a hundred so that is the area

so that's the answer one hundred

now let's say if we have a circle

and there's a right triangle in the

circle

let's call this a

b

and c

and c is the center of the circle

and you're given the length of one side

of the triangle let's say it's eight

your task is to find the area

of

the shaded region

to find the area of the shaded region

it's going to be

the area of the large

object the object on the outside which

is the area of the circle

minus the area

of the object on the inside which is the

area of the triangle

so the area of the shaded region is

going to be pi r squared

minus one half base times height

now notice that eight is the radius of

the triangle the radius is the distance

between the center of the circle and any

point on a triangle

so ac is also the radius of the circle

which means this section is eight

and that's the base and the height of

the triangle

so the radius which is 8 is the same as

the base of the triangle and it's the

same as the height of the triangle

so this is going to be 64 pi

minus

8 times 8 is 64 and half of 64 is 32.

so the area of the shaded region is 64

pi

minus 32.

you