REPRESENTING REAL-LIFE SITUATIONS USING EXPONENTIAL FUNCTIONS || GRADE 11 GENERAL MATHEMATICS Q1
Summary
TLDRThis educational video explores the concept of exponential functions, demonstrating their form and applications in real-life scenarios. It covers the definition of exponential functions, examples with bases greater than zero and not equal to one, and their use in modeling population growth, exponential decay, and compound interest. The script also explains how to construct a table of values for exponential functions and provides step-by-step calculations for various examples, including bacteria growth, radioactive decay, and investment growth. The video concludes with an introduction to the natural exponential function with base e, illustrating its application in temperature change.
Takeaways
- 📚 Exponential functions are mathematical functions of the form \( f(x) = b^x \) where \( b > 0 \) and \( b \neq 1 \).
- 🚫 The base of an exponential function cannot be one because it would result in a constant function regardless of the exponent.
- 📈 Examples of exponential functions include \( f(x) = 6^x \), \( f(x) = 16^x \), and \( f(x) = 3^x + 1 \).
- 🔢 For exponential functions with negative bases, such as \( -4 \) raised to \( 1/2 \), the result is the square root of the base's reciprocal.
- 📊 A table of values for different functions like \( y = (1/3)^x \), \( y = 10^x \), and \( y = 0.8^x \) can be constructed by substituting various values of \( x \).
- 🌱 Applications of exponential functions include modeling population growth, where quantities can double or triple over certain time periods.
- 📉 Exponential decay, such as the half-life of radioactive substances, can also be modeled using exponential functions.
- 💰 Compound interest is another real-life application where the amount of money grows exponentially over time.
- 🔢 The natural exponential function uses the base \( e \) (approximately 2.71828), which is a fundamental constant in mathematics.
- 📉 A table of values for a function involving the natural base \( e \), like the temperature of a meat slab in an oven, can show how the temperature changes over time.
- 🛠️ Using a scientific calculator is recommended to evaluate and check the results of exponential functions and their applications.
Q & A
What is an exponential function?
-An exponential function is a mathematical function of the form f(x) = b^x, where b is the base and x is the exponent. The base b must be greater than 0 and not equal to 1.
Why can't the base of an exponential function be 1?
-If the base b is equal to 1, then for any value of x, the function will always yield a result of 1, making it a constant function rather than an exponential one.
What are some examples of exponential functions mentioned in the script?
-Examples include f(x) = 6^x, f(x) = 16^x, and f(x) = (3^x) + 1.
Why is the function f(x) = x^3 not considered an exponential function?
-The function f(x) = x^3 is not an exponential function because the base is x, which is a variable, and not a constant greater than 0 and not equal to 1.
How do you construct a table of values for an exponential function?
-You substitute given values of x into the function and calculate the corresponding y values. This process is repeated for each x value to create the table.
What is the significance of the base being greater than zero in an exponential function?
-The base being greater than zero ensures that the function will yield positive values for any real number x, which is a requirement for an exponential function.
What is the formula for an exponential growth model in terms of population?
-The formula for an exponential growth model is y = y0 * (growth rate)^(t/time period), where y0 is the initial population, t is the time elapsed, and the growth rate is typically a factor greater than 1.
How is the concept of half-life used in exponential decay?
-In exponential decay, the half-life is the time it takes for half of the substance to decay. The remaining amount of substance after time t is given by y = y0 * (1/2)^(t/half-life period).
What is the formula for compound interest using an exponential function?
-The formula for compound interest is A = P * (1 + r)^t, where A is the amount of money accumulated after n years, including interest, P is the principal amount, r is the annual interest rate, and t is the time the money is invested for in years.
What is the natural base e used for in exponential functions?
-The natural base e, approximately equal to 2.71828, is a fundamental mathematical constant used as the base for the natural exponential function. It is commonly used in calculus and various scientific fields.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Fungsi Eksponen Matematika Peminatan Kelas X - Apersepsi Masalah COVID-19
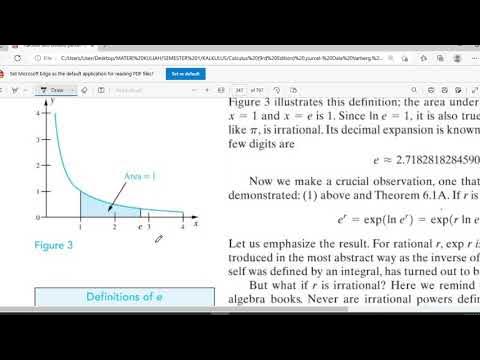
Fungsi Transenden 3 (Fungsi Eksponensial Natural)

Representing Real Life Situations Using Exponential Functions (General Mathematics)

FÁCIL e RÁPIDO | FUNÇÃO EXPONENCIAL

Fungsi Eksponensial Matematika Lanjut Kelas XI
Logarithmic Functions | Transforming Logarithmic Equation to Exponential Form and Vice Versa
5.0 / 5 (0 votes)