Polinomial (Bagian 3) - Pembagian Polinomial Cara Bersusun, Horner dan Horner - Kino
Summary
TLDRThis video script discusses polynomial division, focusing on three methods: synthetic division, Horner's method, and Horner-Kino method. It provides step-by-step examples for each technique, explaining how to find the quotient and remainder in polynomial division, emphasizing the importance of understanding these methods for future mathematical applications.
Takeaways
- 📚 The video discusses the division of polynomials, focusing on three methods: synthetic division, Horner's method, and Horner's method for non-factorable quadratics.
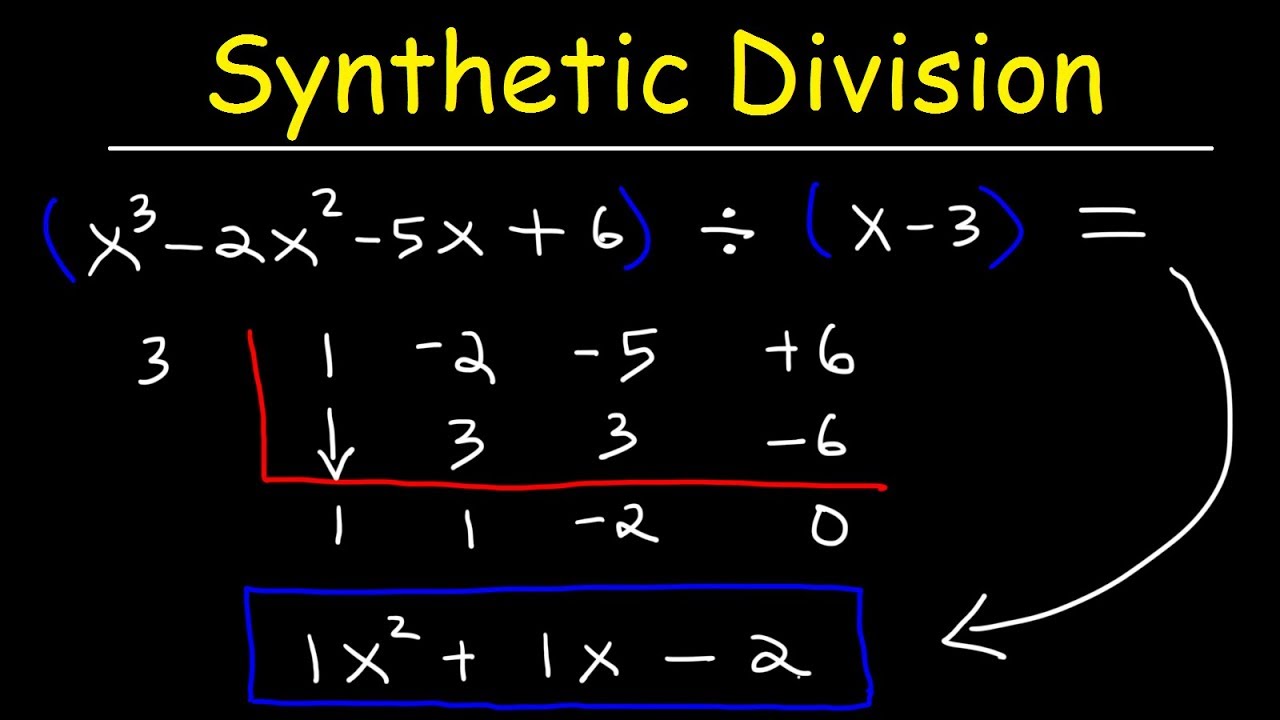
- 🔢 Synthetic division is compared to basic arithmetic division, starting with the highest degree term and working downwards.
- 📉 The example of dividing the polynomial \(2x^3 + 7x^2 - 5x + 1\) by \(x + 3\) is used to illustrate synthetic division, resulting in a quotient of \(2x^2 + x - 8\) and a remainder of 25.
- 🔗 The relationship between the polynomial, divisor, quotient, and remainder is highlighted, emphasizing that the polynomial can be expressed as the product of the divisor and the quotient plus the remainder.
- 📈 The degree of the quotient in polynomial division is explained, stating that it is the degree of the polynomial minus the degree of the divisor.
- 🔍 Synthetic division is applied to another example, dividing \(x^4 - 5x^2 + 6x - 10\) by \(x^2 - 3x + 1\), resulting in a quotient of \(x^2 + 3x + 3\) and a remainder of \(12x - 13\).
- 🌐 Horner's method is introduced as a way to simplify polynomial division, especially when the divisor is a linear term.
- 🔄 The process of applying Horner's method is demonstrated using the same polynomial \(2x^3 + 7x^2 - 5x + 1\) divided by \(x + 3\), yielding the same quotient and remainder as synthetic division.
- 📊 Horner's method for non-factorable quadratic divisors is introduced, showing how to handle cases where the divisor cannot be factored, such as dividing \(2x^4 - 3x^3 + 4x^2 - 2x + 1\) by \(x^2 - 5x + 6\).
- 🔧 The video concludes by summarizing the key points and reminding viewers of the importance of understanding polynomial division for future topics.
Q & A
What is the main topic discussed in the video?
-The main topic discussed in the video is polynomial division, specifically focusing on three methods: synthetic division, Horner's method, and Horner's method with a quadratic divisor.
What is synthetic division?
-Synthetic division is a method of dividing polynomials that is similar to long division, but it is more efficient and involves less writing.
How is the first example of polynomial division in the video solved?
-The first example is solved using synthetic division, where the polynomial 2x^3 + 7x^2 - 5x + 1 is divided by x + 3, resulting in a quotient and a remainder.
What is Horner's method?
-Horner's method is a technique for evaluating polynomials that can also be used for polynomial division. It simplifies the process by reducing the number of multiplications needed.
What is the difference between Horner's method and synthetic division?
-Horner's method is generally used for evaluating polynomials at a specific value and can also be used for division, whereas synthetic division is specifically tailored for polynomial division and is more efficient when dividing by linear factors.
What is the purpose of finding the zero of the divisor in Horner's method?
-Finding the zero of the divisor in Horner's method is used to simplify the process by substituting the zero into the polynomial to evaluate its value, which is then used in the division process.
What is the degree of the polynomial that results from the division of a polynomial of degree 'n' by a polynomial of degree 'm'?
-The degree of the resulting polynomial from such a division is n - m, assuming m < n.
How is the remainder of a polynomial division defined in terms of its degree?
-The degree of the remainder is at most one less than the degree of the divisor. If the divisor is of degree m, the remainder's degree is at most m - 1.
What is the general form of the result of polynomial division?
-The general form of the result of polynomial division is F(x) = P(x) * Q(x) + R(x), where P(x) is the polynomial being divided, Q(x) is the quotient, and R(x) is the remainder.
How is the division of a polynomial by a quadratic divisor approached in the video?
-The division of a polynomial by a quadratic divisor is approached using a modified version of Horner's method, known as Horner's method with a quadratic divisor, which involves finding zeros of the quadratic divisor and using them in the division process.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Lesson 33 Long Division to Find Roots

Pembagian suku banyak dengan cara bersusun - Menentukan hasil dan sisa pembagian

MENENTUKAN NILAI POLINOMIAL (CARA BERSUSUN DAN SKEMA HORNER) - POLINOMIAL (2) - MATEMATIKA KELAS XI

Polinomial (Bagian 2) - Menentukan Nilai Polinomial dengan Substitusi dan Skema Horner

Suku Banyak (teorema sisa dan teorema faktor) #matematika #sma #polinom #sukubanyak #teoremasisa
Synthetic Division of Polynomials
5.0 / 5 (0 votes)