POLINÔMIOS #01 | DEFINIÇÃO FORMA REDUZIDA E GRAU DE UM POLINÔMIO | \Prof. Gis/

Summary

In this lesson, Gis introduces polynomials by explaining their components, including monomials, coefficients, and independent terms. The video covers how polynomials are formed by adding or subtracting monomials and provides insights into their classification, such as monomials, binomials, and trinomials. Gis also explains the process of reducing polynomials by combining like terms and demonstrates how to calculate the degree of a polynomial. Whether dealing with one or multiple variables, the degree of the polynomial is determined by the highest exponent or the sum of exponents in each monomial. The lesson sets the foundation for learning polynomial operations in future classes.

Takeaways

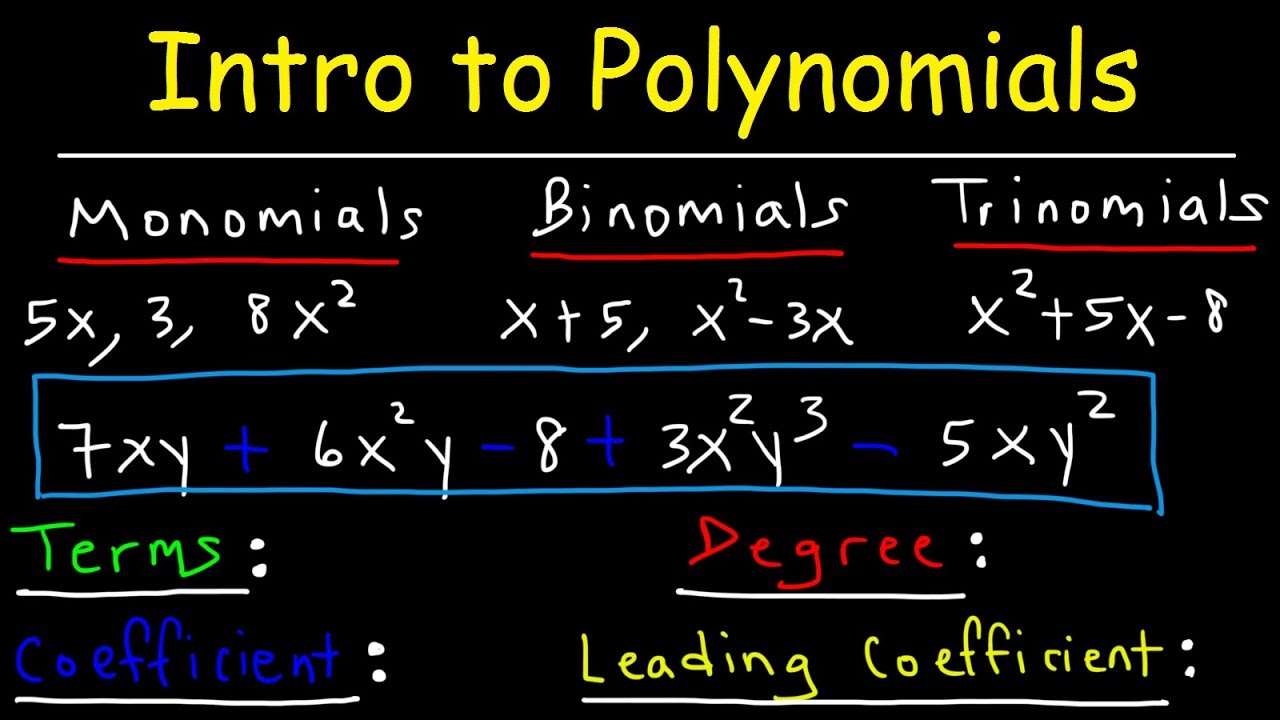
• 😀 Monomials are algebraic expressions with only one term, and polynomials are formed by adding or subtracting monomials.
• 😀 Polynomials are classified by the number of terms they contain: monomials (1 term), binomials (2 terms), trinomials (3 terms), and polynomials (4 or more terms).
• 😀 The coefficient of a monomial is the numerical factor, and the literal part refers to the variable and its exponent.
• 😀 A null monomial has a coefficient of zero, and a polynomial containing it is called a null polynomial.
• 😀 The independent term of a polynomial is the term without any variables, like the 12 in a polynomial.
• 😀 A polynomial is in its reduced form when no like terms remain, meaning terms with identical variables and exponents have been combined.
• 😀 The degree of a polynomial is the highest exponent of its variable(s), and this can be determined by identifying the highest degree among its monomials.
• 😀 To calculate the degree of a polynomial with multiple variables, you must add the exponents of the variables in each monomial, and the highest sum determines the degree of the polynomial.
• 😀 In reduced form, polynomials with more than three terms do not receive special names and are simply referred to as polynomials.
• 😀 Understanding the degree and classification of polynomials is essential for performing operations like addition, subtraction, multiplication, and division of polynomials.

Q & A

What is a monomial?

A monomial is an algebraic expression that consists of only one term. It can include a coefficient, a variable, and an exponent, such as 15x³ or -9x⁴y.

What is the definition of a polynomial?

A polynomial is an algebraic expression that is formed by adding or subtracting finite monomials. For example, 15x³ + 6x² - 9x - 12 is a polynomial with four terms.

What is a null monomial?

A null monomial is a monomial where the coefficient is zero, such as 0x³. It does not contribute to the polynomial and is often omitted.

What is the independent term in a polynomial?

The independent term in a polynomial is the term that does not have a variable. For example, in the polynomial 15x³ + 6x² - 9x - 12, the independent term is -12.

What does it mean for a polynomial to be in reduced form?

A polynomial is in reduced form when it has no like terms left to combine. This means that all monomials with the same literal part have been simplified by combining their coefficients.

What are the special names given to polynomials based on the number of terms?

Polynomials are classified based on their number of terms. A monomial has one term, a binomial has two terms, a trinomial has three terms, and any polynomial with four or more terms is simply called a polynomial.

How do you calculate the degree of a polynomial?

The degree of a polynomial is determined by the highest exponent of its variable(s). If the polynomial contains more than one variable, the degree of each monomial is the sum of the exponents of its variables, and the highest degree among the monomials defines the degree of the polynomial.

What is the degree of the polynomial 3x⁴ + 2x³ - 5x²?

The degree of the polynomial 3x⁴ + 2x³ - 5x² is 4 because the highest exponent of the variable x is 4.

How do you determine the degree of a polynomial with multiple variables?

To determine the degree of a polynomial with multiple variables, you need to calculate the degree of each monomial separately by adding the exponents of its variables. The highest sum of exponents among the monomials gives the degree of the polynomial.

Can you provide an example of a polynomial with more than three terms? What is its classification?

An example of a polynomial with more than three terms is 2A¹B⁷ + 3A³B² - 5A⁷B² + 7AB. Since it has four terms, it is simply called a polynomial and does not have a special name like monomial, binomial, or trinomial.