MENGHITUNG NILAI PELUANG | MATEMATIKA | KELAS 8 #BelajarBarengMrJS

Summary

In this video, the presenter explains the process of creating sample spaces and understanding probability using familiar examples like coins and dice. Starting with the basic concept of sample points for a single coin, the script expands to multiple coins and dice, showing how to systematically build sample spaces with tables. It also touches on empirical probability through examples of repeated coin flips and dice throws. By the end, viewers will understand how to calculate theoretical and empirical probabilities and apply them to real-life scenarios, enhancing their grasp of probability theory.

Takeaways

• 😀 Sample space represents all possible outcomes in an experiment, like heads or tails for a coin toss.
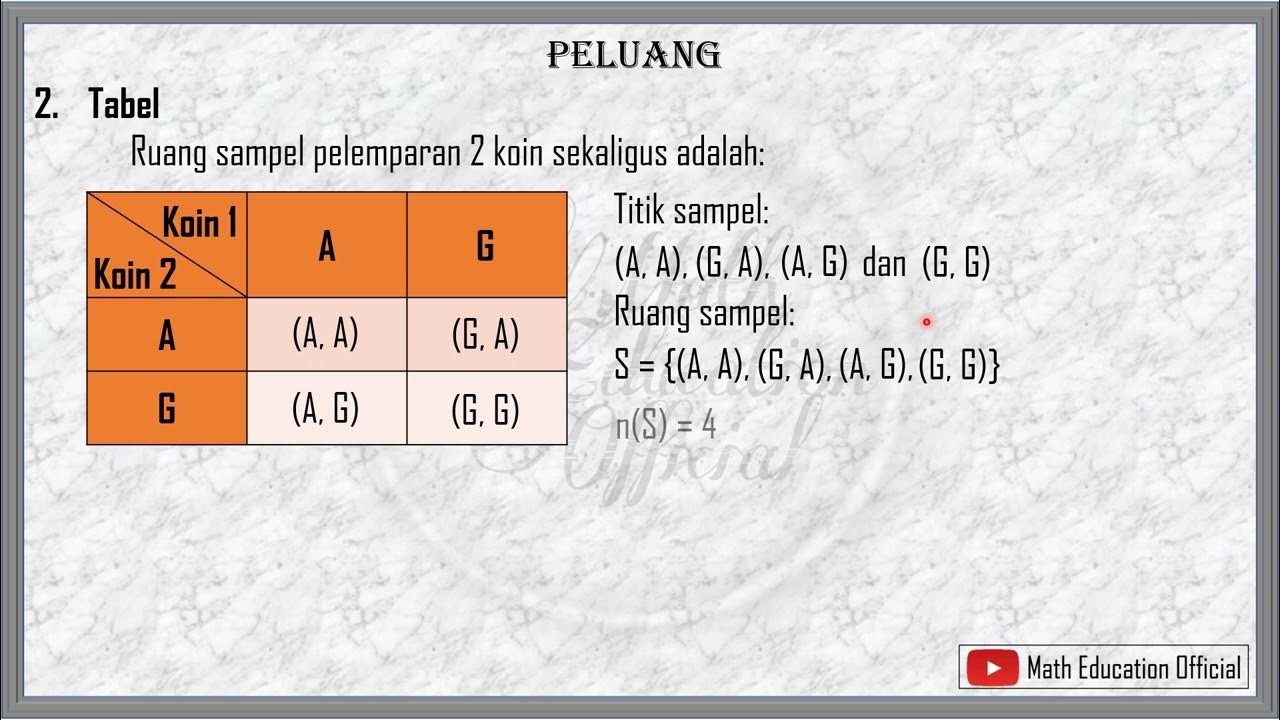
• 😀 A sample space can be created using a table or matrix to organize all possible outcomes for combined events.
• 😀 For multiple coin tosses, the sample space expands as the number of coins increases, e.g., 2 coins have 4 possible outcomes.
• 😀 Theoretical probability is based on expected outcomes, while empirical probability relies on observed data from experiments.
• 😀 To calculate theoretical probability, divide the number of favorable outcomes by the total number of outcomes in the sample space.
• 😀 Empirical probability is calculated by dividing the number of occurrences of an event by the total trials.
• 😀 For dice rolls, a sample space consists of 6 outcomes (1-6), and a matrix can be used for multiple dice.
• 😀 When combining multiple events, like tossing coins and rolling dice, the sample space grows, requiring the use of tables to visualize combinations.
• 😀 In probability problems, first construct the sample space, then identify favorable outcomes to apply the probability formula.
• 😀 Practice with various examples, such as coin tosses, dice rolls, and marbles, to understand how to calculate probabilities in different scenarios.

Q & A

What is the concept of a sample space in probability?

A sample space refers to all possible outcomes of an experiment. For example, with a coin, the sample space consists of two outcomes: heads and tails.

How can we construct the sample space for two coins?

For two coins, you can use a table where each coin's outcome is listed. For example, the outcomes for two coins would be: HH, HT, TH, TT.

What is the significance of sample points in probability?

Sample points are the individual outcomes within the sample space. For example, when tossing a coin, heads or tails are sample points.

How can we find the sample space for three coins?

To find the sample space for three coins, list all possible combinations of heads and tails. This would give us 8 outcomes: HHH, HHT, HTH, HTT, THH, THT, TTH, TTT.

What is the process for constructing a sample space for a die?

For a die, the sample space consists of six outcomes: 1, 2, 3, 4, 5, and 6. When rolling two dice, you list all the possible pairs of numbers from each die.

How do we calculate the sample space for two dice?

For two dice, create a table where each die is labeled with its possible outcomes (1-6). By combining each outcome of the first die with each of the second die, you get 36 possible outcomes.

What happens when you combine a coin and a die in a probability experiment?

When combining a coin and a die, you multiply the number of outcomes for the coin (2 outcomes: heads, tails) by the number of outcomes for the die (6 outcomes), resulting in 12 total outcomes.

What is the formula for calculating theoretical probability?

The formula for theoretical probability is P = (Number of favorable outcomes) / (Total number of possible outcomes). This helps calculate the likelihood of an event occurring.

How do you find the empirical probability of an event?

Empirical probability is calculated by dividing the number of times an event occurs by the total number of trials. For example, if a coin lands heads 96 times in 200 trials, the empirical probability of heads is 96/200.

How do you handle a situation where an event involves conditional probability, like drawing marbles from a bag without replacement?

In conditional probability, when an event affects the outcome of another, like drawing marbles without replacement, you adjust the sample space by removing the item already drawn. For example, if two green marbles are drawn from a bag of 25 blue, 12 red, and 15 green marbles, the new sample space would reflect the remaining items.