Menentukan Peluang Empirik Frekuensi Relatif dari Suatu Kejadian

Summary

In this educational video, the presenter introduces the concept of empirical probability, also known as relative frequency, through practical examples. The explanation begins with basic definitions, contrasting empirical probability with theoretical probability. The video walks viewers through various examples, such as tossing a coin, drawing candies from a jar, and rolling a die, to calculate the relative frequency of different outcomes. Each example is clearly explained step by step, making complex ideas accessible and easy to follow. The lesson is concluded with encouragement for viewers to ask questions in the comments and engage further with the material.

Takeaways

• 😀 Understanding empirical probability is essential in mathematics as it helps determine the likelihood of an event based on experiment results.
• 😀 Empirical probability, also called relative frequency, is the ratio of the number of successful outcomes to the total number of trials in an experiment.
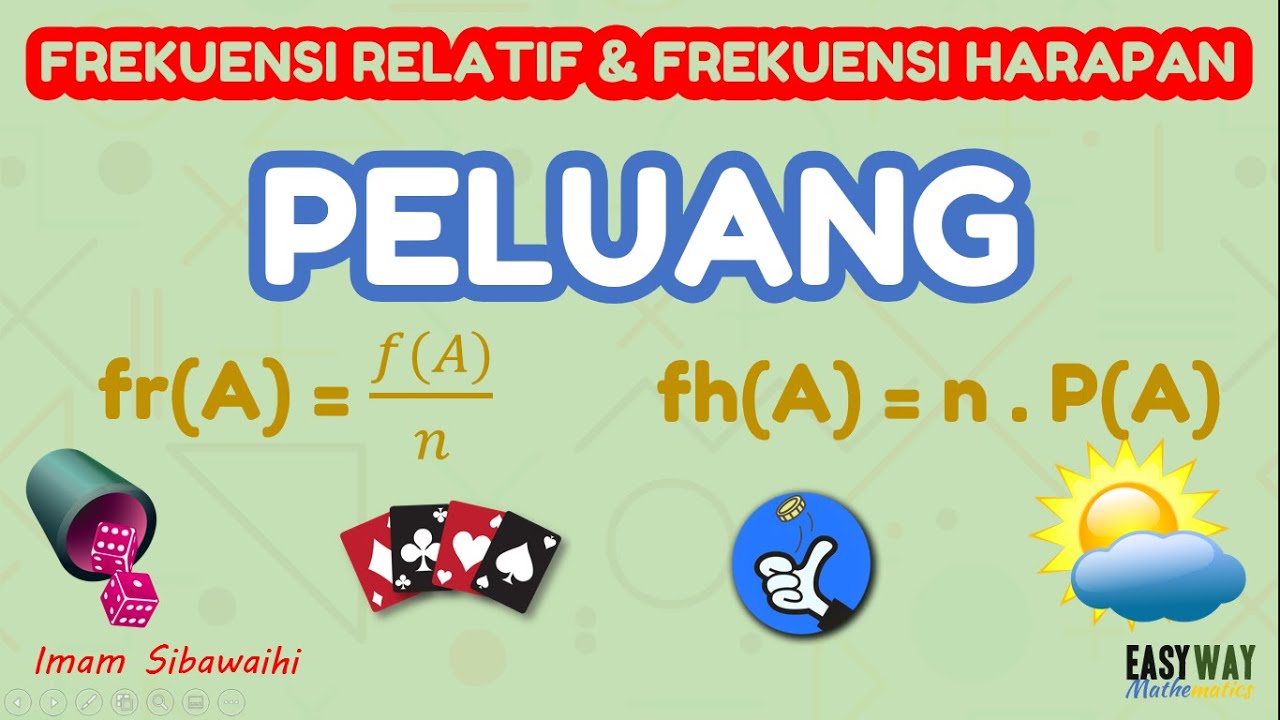
• 😀 Empirical probability can be calculated using the formula: FR(A) = n(A) / n, where FR(A) is the relative frequency of event A, n(A) is the number of occurrences of event A, and n is the total number of trials.
• 😀 The empirical probability of getting heads in a coin toss can be calculated by dividing the number of heads by the total number of tosses.
• 😀 In a coin toss experiment where heads appears 20 times out of 50 tosses, the empirical probability of heads is 2/5.
• 😀 Similarly, the empirical probability of tails (or other outcomes) can be calculated using the same formula, adjusting for the number of occurrences.
• 😀 The script uses multiple examples, such as the coin toss and the candy experiment, to demonstrate how to calculate empirical probabilities for different scenarios.
• 😀 When conducting experiments with multiple outcomes (like choosing colored candies), the probability of each outcome can be found by dividing the occurrences of each event by the total number of trials.
• 😀 The script also discusses calculating the relative frequency of events in cases where outcomes are more complex, such as when there are multiple categories (e.g., red, green, and yellow candies).
• 😀 It emphasizes simplifying fractions when calculating empirical probabilities to make them easier to interpret, as seen in examples where fractions like 4/15 and 1/3 were simplified.

Q & A

What is empirical probability?

Empirical probability is the probability calculated from experimental or observed data. It is determined by the ratio of the number of times an event occurs to the total number of trials.

What is the formula for calculating relative frequency (empirical probability)?

The formula for calculating relative frequency is: FR(A) = n(A) / n, where n(A) is the number of occurrences of event A, and n is the total number of trials.

How do you calculate the empirical probability of getting 'Heads' when tossing a coin 50 times and getting 'Heads' 20 times?

To calculate the empirical probability of getting 'Heads', use the formula: FR(Heads) = n(Heads) / n = 20 / 50 = 2/5.

How do you calculate the empirical probability of getting 'Tails' when tossing a coin 50 times?

For 'Tails', the probability is calculated as: FR(Tails) = n(Tails) / n = 30 / 50 = 3/5.

What is the difference between empirical probability and theoretical probability?

Empirical probability is based on the results of actual experiments, while theoretical probability is based on mathematical calculations assuming ideal conditions (e.g., the ratio of favorable outcomes to total possible outcomes).

How do you calculate the relative frequency of selecting a red candy from a jar where 8 out of 30 candies selected were red?

The relative frequency for selecting a red candy is: FR(Red) = n(Red) / n = 8 / 30 = 4/15.

If you select 30 candies and get 12 green, what is the relative frequency of selecting a green candy?

The relative frequency of selecting a green candy is: FR(Green) = n(Green) / n = 12 / 30 = 2/5.

In a dice roll experiment where you roll a die 100 times, how do you calculate the relative frequency of rolling a '1'?

The relative frequency for rolling a '1' is calculated as: FR(1) = n(1) / n = 20 / 100 = 1/5.

How do you calculate the probability of rolling a number less than 4 in a dice roll experiment where 20 rolls were '1', 14 rolls were '2', and 16 rolls were '3'?

To find the relative frequency of rolling a number less than 4, sum the occurrences of '1', '2', and '3' (20 + 14 + 16 = 50), then calculate: FR(Less than 4) = 50 / 100 = 1/2.

What is the empirical probability of rolling a number greater than 3 when rolling a die 100 times, given 20 rolls were '1', 14 were '2', and 16 were '3'?

The probability of rolling a number greater than 3 is the remaining 50 rolls (i.e., '4', '5', and '6'). The relative frequency is: FR(Greater than 3) = 50 / 100 = 1/2.