sta301 lec 19 | STA301 short lec 19 | Statistics and probability in urdu /Hindi
Summary
TLDRThis video lecture covers Lecture 19 of the State 301 course, focusing on the definition of probability through relative frequency and the posterior approach. It delves into empirical, statistical, and classical types of probability, explaining how probability can be derived from repeated experiments. The lecture also explains key formulas, examples, and concepts like the complement of an event and addition laws in probability. Viewers are encouraged to join the community for more learning and stay updated with upcoming lectures. The content is designed to help students grasp the various approaches and types of probability in practical scenarios.
Takeaways
- 😀 The lecture focuses on understanding the concept of probability and its different definitions, such as relative frequency and posterior probability.
- 😀 Relative frequency, also known as posterior probability, is based on past experiences or experiments, making it an objective approach to calculating probability.
- 😀 Probability can be calculated using relative frequency, which is the ratio of favorable outcomes to the total number of trials, especially in large-scale experiments.
- 😀 The probability formula for relative frequency is defined as the limit of the ratio (M / N) as N approaches infinity, where M is the number of successful outcomes and N is the total number of trials.
- 😀 The script discusses empirical or statistical probability, where outcomes are based on experiments rather than theoretical assumptions.
- 😀 A key example in the lecture involves tossing a coin 10,000 times and calculating the probability of getting heads and tails based on relative frequencies.
- 😀 The concept of complement probability is explained, where the probability of an event occurring is related to its complement (1 - P(A)).
- 😀 Classical or theoretical probability, where outcomes are equally likely, is also discussed as part of understanding the different approaches to calculating probability.
- 😀 The addition rule in probability is introduced, explaining how to find the probability of the union of two events using the formula P(A ∪ B) = P(A) + P(B) - P(A ∩ B).
- 😀 The importance of understanding probability in real-world scenarios is emphasized, with practical examples such as calculating the likelihood of births or deaths in a population.
Q & A
What is the main topic of lecture number 19 in the transcript?
-The main topic of lecture number 19 focuses on the definition of probability, relative frequency, and different approaches to probability, such as the Posterior Definition of Probability.
What does 'Relative Frequency' refer to in the context of probability?
-'Relative Frequency' in probability refers to how often an event occurs relative to the total number of trials or observations. It is also known as the Posterior Definition of Probability, based on past experiences or experiments.
How is 'Relative Frequency' different from 'Classical Probability'?
-'Relative Frequency' is based on actual experiments and past data, whereas 'Classical Probability' is based on equally likely outcomes, often used in idealized scenarios like tossing a fair coin or rolling a fair die.
What is the formula for calculating the probability using relative frequency?
-The formula for calculating probability using relative frequency is: Probability of event A = M / N, where M is the number of times event A occurs, and N is the total number of trials or experiments.
What does the term 'Posterior Probability' mean?
-'Posterior Probability' refers to the probability of an event occurring, updated based on new data or evidence from past experiences or repeated experiments.
What is the relationship between relative frequency and probability as the number of trials increases?
-As the number of trials (N) increases, the relative frequency (M/N) tends to stabilize and approach a constant value, making the probability more reliable and accurate.
How do we calculate the probability of heads or tails in a coin toss using relative frequency?
-To calculate the probability of heads or tails in a coin toss, we divide the number of occurrences of heads (or tails) by the total number of tosses. For example, if heads appears 5017 times out of 10,000 tosses, the probability is 5017 / 10,000.
What does the term 'Empirical Probability' refer to?
-'Empirical Probability' refers to the probability calculated from observed data or experiments, also called 'Relative Frequency Probability' or 'Statistical Probability'.
What is the Complementary Probability formula?
-The Complementary Probability formula is: Probability of event A = 1 - Probability of the complement of A. This means that if the probability of an event A is known, the probability of not A can be found by subtracting the probability of A from 1.
What is the role of the Addition Rule in probability?
-The Addition Rule in probability helps calculate the probability of the union of two events. It is given by: P(A or B) = P(A) + P(B) - P(A and B). This formula accounts for the overlap of events A and B to avoid double counting.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

TI Sesi #2 - Review teori probabilitas
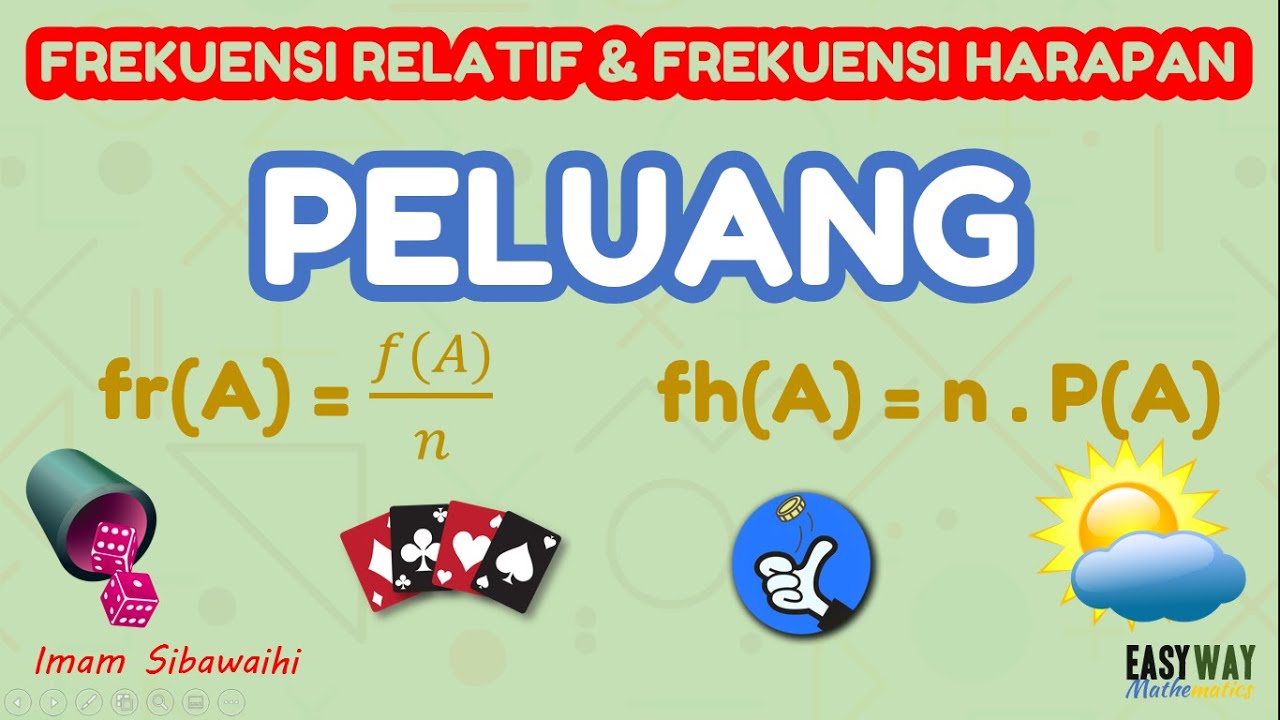
Frekuensi Relatif dan Frekuensi Harapan

Peluang ~ Menentukan Nilai Peluang dan Frekuensi Harapan Suatu Kejadian (Materi PJJ Klas VIII/8 SMP)

Konsep Dasar Ilmu Negara

06. Termodinamika Metalurgi (Segmen 01: Konsep Aktivitas Termodinamika)

Introduction to Theory of Computation
5.0 / 5 (0 votes)