Teorema Sudut dalam Lingkaran

Summary

This video tutorial covers essential theorems related to angles in circles. It explains key definitions such as circles, arcs, and angles formed by radii and chords. The first major theorem demonstrates how the central angle is twice the size of the inscribed angle facing the same arc. The video also covers several derived theorems, including the relationship between opposite angles in cyclic quadrilaterals. The tutorial provides step-by-step proofs and examples, ensuring students grasp the mathematical principles. It concludes with exercises to help viewers practice and reinforce their understanding.

Takeaways

• 😀 A circle is defined as a set of points equidistant from a central point.
• 😀 An arc is a portion of the circle defined by two points on the circle.
• 😀 A central angle is an angle with its vertex at the center of the circle.
• 😀 An inscribed angle is an angle formed by two chords meeting at a point on the circle.
• 😀 The diameter is a chord passing through the center of the circle.
• 😀 The first theorem: The inscribed angle is always half the measure of the central angle subtended by the same arc.
• 😀 To prove the first theorem, congruent isosceles triangles are used to establish the relationship between central and inscribed angles.
• 😀 The second theorem: Opposite angles in a cyclic quadrilateral (a quadrilateral inscribed in a circle) add up to 180 degrees.
• 😀 For cyclic quadrilaterals, each pair of opposite angles sums to 180 degrees due to the inscribed angle theorem.
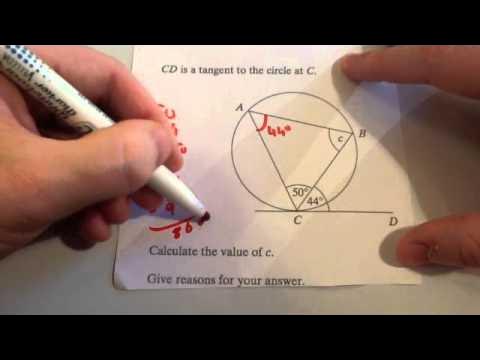
• 😀 Practice problems in the video demonstrate how to calculate angles in circles using the above theorems, such as finding missing angles and verifying angle sums.

Q & A

What is the definition of a circle?

A circle is defined as the set of all points that are equidistant from a fixed point called the center.

What is a 'busur' (arc) in a circle?

A 'busur' (arc) is a part of the circle bounded by two points on the circle.

What is the difference between a minor arc and a major arc?

A minor arc is the smaller arc between two points on the circle, while a major arc is the larger arc between the same two points.

What is the definition of a central angle in a circle?

A central angle is an angle with its vertex at the center of the circle, and its sides are radii that intersect the circle.

What is a chord in a circle?

A chord is a straight line segment whose endpoints lie on the circle.

What is the relationship between a central angle and the arc it subtends?

The measure of a central angle is equal to the measure of the arc it subtends.

What does the first theorem state about an inscribed angle and a central angle?

The first theorem states that an inscribed angle is half the size of the central angle that subtends the same arc.

How do we prove that the measure of the central angle is twice the measure of the inscribed angle?

We prove it by showing that two isosceles triangles are formed, and by using the properties of the angles in these triangles, we find that the central angle is twice the inscribed angle.

What is the second theorem related to angles in cyclic quadrilaterals?

The second theorem states that the opposite angles in a cyclic quadrilateral (a quadrilateral inscribed in a circle) are supplementary, meaning their sum is 180 degrees.

What is the method to find the measure of an inscribed angle given a specific arc?

To find the measure of an inscribed angle, we calculate half of the measure of the arc it subtends.