PERBEDAAN FUNGSI INJEKTIF, SURJEKTIF DAN BIJEKTIF LENGKAP DENGAN CONTOH SOAL

Summary

In this video, the host explains the differences between three types of functions: injective, surjective, and bijective. An injective function maps each element of the domain to a unique element in the codomain, while a surjective function ensures that every element of the codomain has at least one element in the domain mapped to it. A bijective function combines both properties, where each element in the domain is paired with a unique element in the codomain and vice versa. The video includes visual aids and examples to clarify these concepts and their properties.

Takeaways

• 😀 Functions are classified into three types: injective, surjective, and bijective, based on their properties.
• 😀 An injective function (also called a one-to-one function) maps each element of the domain to a unique element in the codomain.
• 😀 In an injective function, no element in the codomain has more than one corresponding element in the domain.
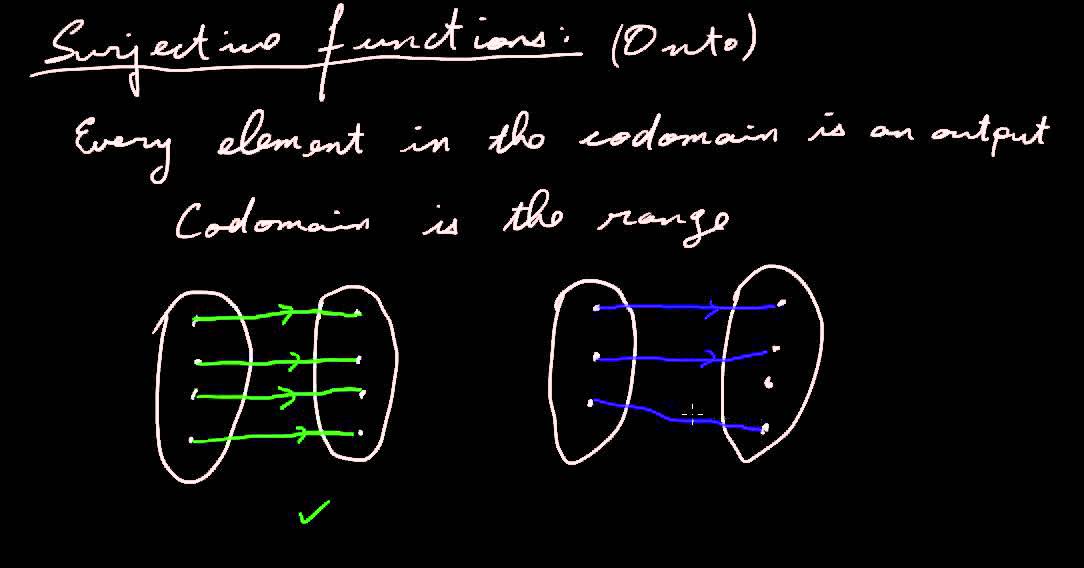
• 😀 A surjective function (onto function) allows one or more elements in the codomain to be paired with elements from the domain, but every element of the codomain must be paired.
• 😀 For a surjective function, the number of elements in the codomain must be equal to or fewer than the number of elements in the domain.
• 😀 A bijective function combines both injectivity and surjectivity, meaning each element in the domain has a unique and corresponding element in the codomain, and all codomain elements are paired.
• 😀 In a bijective function, the inverse of the function is also a valid function, which is not necessarily true for injective or surjective functions.
• 😀 Example 1: f(x) = x + 3. The function is injective but not surjective because one element of the codomain has no pairing in the domain.
• 😀 Example 2: f(x) = x². This function is neither injective nor surjective because multiple elements in the domain can map to the same element in the codomain, and some elements in the codomain have no corresponding pair.
• 😀 Understanding these properties of functions is essential for identifying whether a function is injective, surjective, or bijective, which in turn helps in various mathematical analyses and applications.

Q & A

What is an injective function?

An injective function, also known as a one-to-one function, is a function where each element of the codomain is paired with at most one element of the domain. No element in the codomain has more than one pre-image in the domain.

What is the key property of a surjective function?

A surjective function, also known as an onto function, ensures that every element in the codomain has at least one element in the domain mapped to it. In other words, there are no unpaired elements in the codomain.

What defines a bijective function?

A bijective function is a function that is both injective and surjective. This means that each element of the domain is paired with exactly one element of the codomain, and every element of the codomain has a pre-image in the domain.

Can a surjective function have more than one element in the codomain mapped to a single element in the domain?

Yes, a surjective function allows multiple elements in the codomain to be mapped to a single element in the domain. However, all elements in the codomain must still have at least one pre-image.

What is the relationship between the number of elements in the domain and codomain in a surjective function?

In a surjective function, the number of elements in the codomain should be the same as or less than the number of elements in the domain. This ensures that all elements in the codomain are paired with an element in the domain.

Why is the function f(x) = x + 3 not surjective in the provided example?

The function f(x) = x + 3 is not surjective because one element in the codomain (set B) does not have a corresponding element in the domain, making it impossible to cover the entire codomain.

How do you determine whether a function is injective from a diagram?

A function is injective if each element in the codomain is paired with at most one element in the domain, as shown by the diagram. If an element of the codomain has more than one pre-image in the domain, the function is not injective.

What is the primary difference between an injective and surjective function?

An injective function ensures that no element in the codomain has more than one pre-image in the domain, whereas a surjective function guarantees that every element in the codomain has at least one pre-image in the domain.

Why is the function f(x) = x^2 not injective in the provided example?

The function f(x) = x^2 is not injective because elements in the domain (such as -2 and 2) have the same output in the codomain (both map to 4), meaning multiple domain elements map to the same codomain element.

What happens if a function is neither injective nor surjective?

If a function is neither injective nor surjective, it does not meet the criteria for being bijective. In such a case, the function may have both unpaired elements in the codomain and multiple elements in the codomain paired with the same element in the domain.