TITIK BERAT BENDA

Summary

In this educational video, Bu Nur explains the concept of the center of mass (also known as the centroid) for various shapes. She covers three types of objects: one-dimensional, two-dimensional, and three-dimensional. The lesson includes examples and calculations for each type, detailing how to determine the center of mass for lines, areas, and volumes. Bu Nur also emphasizes the use of formulas to compute the coordinates of the centroid, while discussing different geometric shapes like rectangles, circles, and cylinders. The session concludes with a practical application of these concepts in solving problems related to the center of mass.

Takeaways

• 😀 One-dimensional objects have a center of mass calculated using the object's length and its position along the x or y-axis.
• 😀 Two-dimensional objects, like triangles and squares, have a center of mass determined by their area and coordinates.
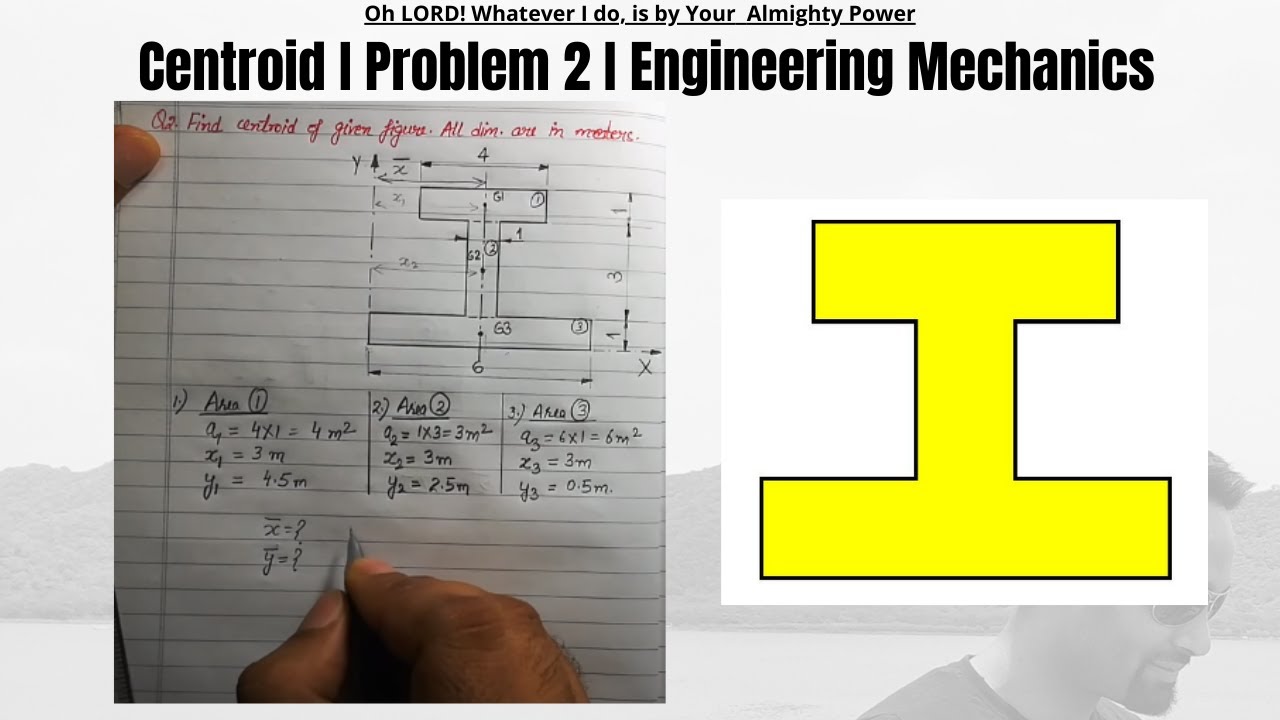
• 😀 For two-dimensional objects, you may need to divide the object into simpler parts to calculate the center of mass for each part separately.
• 😀 Three-dimensional objects, like cylinders or spheres, have a center of mass calculated using their volume and coordinates in three-dimensional space.
• 😀 The general formula for the center of mass is: X0 = (sum of (L * X)) / sum of L, where L represents length, area, or volume, and X is the coordinate.
• 😀 For two-dimensional shapes, the center of mass formula includes both x and y coordinates, as well as the area of each part of the object.
• 😀 The center of mass for a one-dimensional object can be found by multiplying the length of each section by its position and dividing by the total length.
• 😀 In the case of three-dimensional objects, the center of mass depends on whether the object is standing (on the y-axis) or lying down (on the x-axis).
• 😀 For objects that are not simple in shape, decomposition is necessary to break them into simpler shapes to calculate the center of mass.
• 😀 Bu Nur explains that for problems involving multiple objects, the center of mass of each object is calculated and then averaged based on their total lengths, areas, or volumes.

Q & A

What is the topic being discussed in the transcript?

The topic discussed is the concept of the center of gravity (titik berat) in various types of objects, categorized into one-dimensional, two-dimensional, and three-dimensional objects.

How many types of objects are discussed in terms of their center of gravity?

There are three types of objects discussed: one-dimensional, two-dimensional, and three-dimensional objects.

What is the formula for calculating the center of gravity for a one-dimensional object?

For a one-dimensional object, the center of gravity (x0, y0) can be calculated using the formula: (L1 * X1 + L2 * X2 + L3 * X3) / (L1 + L2 + L3) for x0, and a similar approach for y0.

How is the center of gravity calculated for a two-dimensional object?

For a two-dimensional object, the center of gravity is calculated using the formula: (A1 * X1 + A2 * X2 + ... + An * Xn) / (A1 + A2 + ... + An) for x0, and a similar formula for y0, where A represents the area of the sections of the object.

How is the center of gravity calculated for a three-dimensional object?

For a three-dimensional object, the center of gravity is calculated by taking into account the volume of the object, and it is determined based on the axis of rotation (either x, y, or z).

What does a one-dimensional object typically look like?

A one-dimensional object typically appears as a simple line, which can be either horizontal or vertical.

What kinds of shapes are described as two-dimensional objects in the script?

Two-dimensional objects in the script include shapes like triangles, circles, and squares, which have length and width but no depth.

What is an example of a three-dimensional object mentioned in the transcript?

Examples of three-dimensional objects discussed include a cylinder and a hemisphere, which have volume and occupy space in all three dimensions.

What happens when the three-dimensional object is in a standing position versus lying down?

When a three-dimensional object is standing, the center of gravity is calculated along the y-axis, while if it is lying down, the center of gravity is calculated along the x-axis.

What is the significance of dividing the object into sections when calculating the center of gravity?

Dividing the object into sections helps in calculating the center of gravity of irregular shapes, especially for two-dimensional objects, as it simplifies the calculation of the weighted average position of each section.