dy/dx, d/dx, and dy/dt - Derivative Notations in Calculus
Summary
TLDRThis video explains the difference between 'dydx' and 'd/dx' in the context of derivatives. The speaker outlines how 'd/dx' is a command telling you to differentiate a function with respect to x, while 'dydx' is a statement indicating the derivative of y with respect to x. Examples are provided, including how to differentiate expressions like x³ + 4x² and y³ using the power rule. The video also touches on implicit differentiation, related rates problems, and how to differentiate with respect to different variables such as s and t. A pop quiz is included to reinforce the concept.
Takeaways
- 😀 The notation 'd/dx' is a command that tells you to differentiate a function with respect to x.
- 😀 'dydx' simply makes a statement about the derivative of y with respect to x.
- 😀 Differentiating a function like x³ + 4x using 'd/dx' yields 3x² + 4.
- 😀 The difference between 'd/dx' and 'dydx' is that one tells you to differentiate, while the other gives the result of the derivative.
- 😀 Implicit differentiation involves functions where the dependent variable (y) is itself a function of x, requiring an extra 'dy/dx' term.
- 😀 When differentiating a function like y³ with respect to x, you'll use the chain rule and multiply by 'dy/dx'.
- 😀 In related rates problems, you differentiate with respect to time (t), often yielding expressions like dx/dt or dy/dt.
- 😀 For example, differentiating x⁵ with respect to t gives 5x⁴ * dx/dt.
- 😀 Related rates problems are common in physics and calculus, where you analyze how multiple variables change with respect to time.
- 😀 A practical example involves differentiating r = x³ + t⁵ with respect to y, which involves the chain rule and understanding how each variable relates to y.
Q & A
What is the difference between dydx and d/dx?
-d/dx is a command that tells you to differentiate a function with respect to x, while dydx is a statement that indicates the derivative of y with respect to x.
When you see dydx, what does it represent?
-dydx represents the derivative of y with respect to x, meaning it shows how y changes as x changes.
What does the notation d/dx tell you to do?
-The notation d/dx tells you to differentiate the function inside the parentheses with respect to x.
How do you differentiate the function x³ + 4x² with respect to x?
-Using the power rule, the derivative of x³ + 4x² with respect to x is 3x² + 8x.
What is implicit differentiation?
-Implicit differentiation is when you differentiate equations where the variables are not explicitly isolated, such as when both x and y appear together in an equation.
How do you differentiate y³ with respect to x?
-To differentiate y³ with respect to x, you use the chain rule. The result is 3y² times dydx because y depends on x.
What happens when you differentiate with respect to a different variable, like s or t?
-When you differentiate with respect to a different variable, like s or t, you modify the notation to reflect that, such as dx/ds or dx/dt, and apply the corresponding derivative rules.
What does dx/dt represent?
-dx/dt represents the derivative of x with respect to time, often used in related rates problems where the rate of change of one variable depends on time.
How do you differentiate x⁴ + y³ with respect to time?
-To differentiate x⁴ + y³ with respect to time, you apply the chain rule: the derivative is 4x³ times dx/dt plus 3y² times dydt.
How do you find the derivative of r = x³ + t⁵ with respect to y?
-To differentiate r = x³ + t⁵ with respect to y, you use the chain rule. The result is 3x² times dx/dy + 5t⁴ times dt/dy.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Complete Integration and Derivative Formulae List | Easy Trick to Learn| Engineering Mathematics 2

ĐẠO HÀM và ý nghĩa hình học (Derivative Intro) | Vật Lý Chill

dYdX on Arbitrum: How To Start Trading in Under 5 Minutes

CFA Level I Derivatives - Forward Contracts vs Futures Contracts
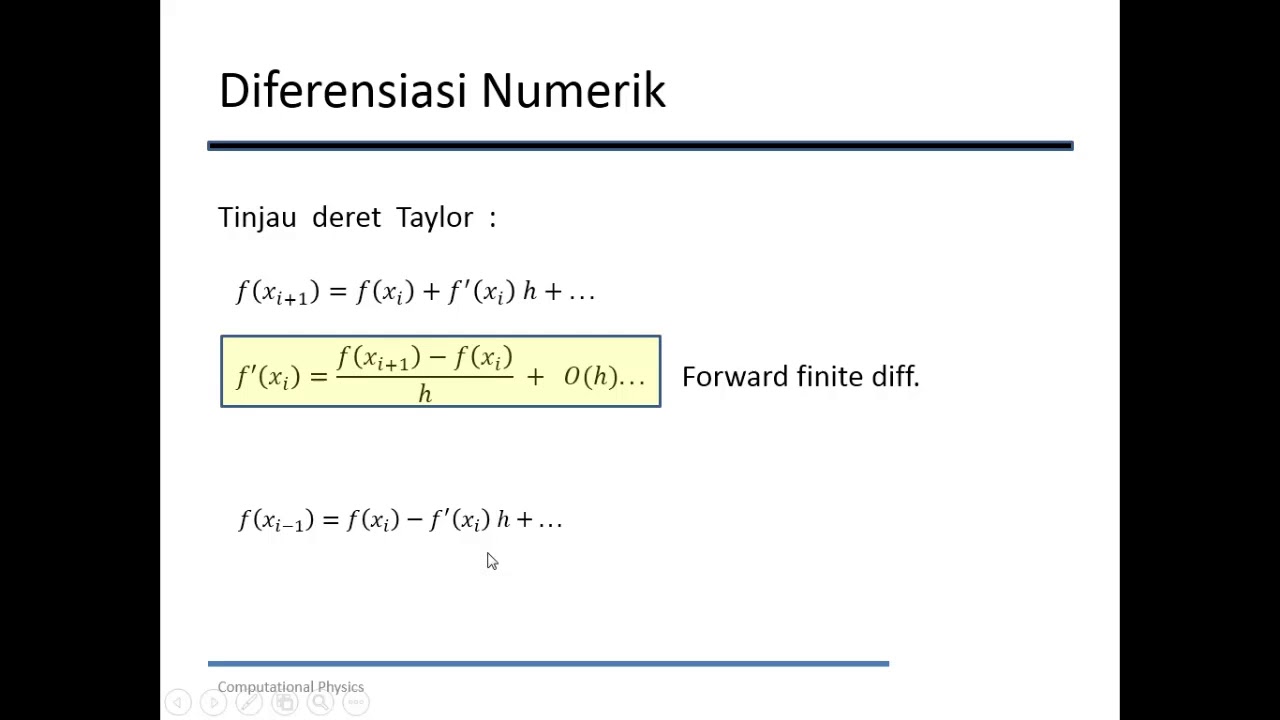
Diferensiasi Numerik
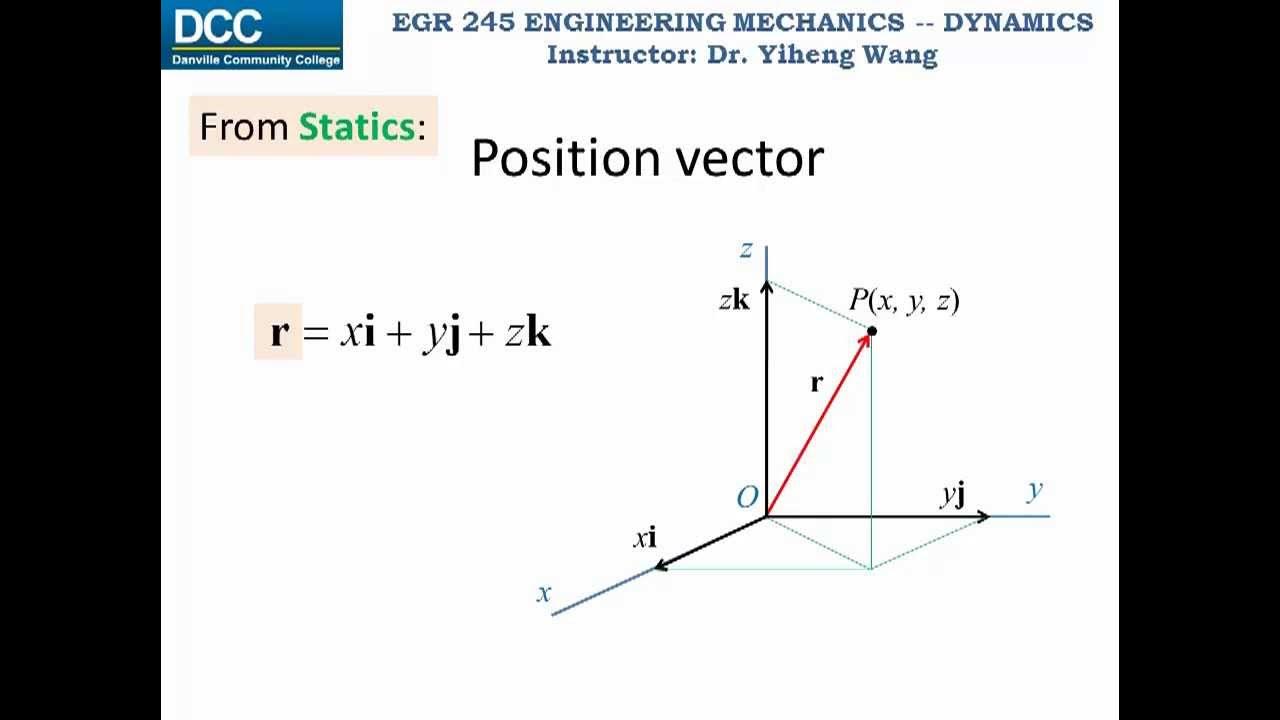
Dynamics Lecture 02: Particle kinematics, Rectilinear continuous motion part 1
5.0 / 5 (0 votes)