Solving Quadratic Equations
Summary
TLDRThis educational video script focuses on solving quadratic equations and inequalities. It introduces methods like factorization for equations with 'a' coefficients equal to 1, and for those not equal to 1, it suggests extracting common factors. The script also covers completing the square and using the quadratic formula for solutions. It concludes with solving quadratic inequalities by factorization and analyzing regions on a number line, providing a comprehensive guide to quadratic equations and their applications.
Takeaways
- The session focuses on solving quadratic equations and inequalities, with an emphasis on factorization, completing the square, and the quadratic formula.
- Three types of quadratic equations are discussed based on the coefficients of x², x, and the constant term: b=0, c=0, and when neither b nor c is zero.
- For equations with b=0, the solution involves isolating x² and taking the square root of the constant term.
- When c=0, the highest common factor is extracted, and the equation is solved by setting each factor equal to zero.
- In cases where b≠0 and c=0, two numbers are identified whose product is the constant term and whose sum is b, then the equation is factored accordingly.
- For equations where the coefficient of x² is not 1, common factors are first extracted, and the equation is rearranged for easier solution.
- Completing the square involves creating a perfect square trinomial and isolating it to solve for x.
- The quadratic formula x = (-b ± √(b² - 4ac)) / 2a is used for solving equations that don't factor easily, with a, b, and c being coefficients from the equation.
- Solving quadratic inequalities involves factoring and testing intervals on a number line to determine where the inequality holds true.
- The solution to a quadratic inequality is represented on a number line, showing the intervals where the inequality is satisfied.
Q & A
What are the three types of quadratic equations with 'a' or the coefficient of x² equal to 1?
-The three types are: 1) When b equals 0, such as x² - c = 0. 2) When c equals 0, such as x² + bx = 0. 3) When b does not equal zero and c equals zero, such as x² + bx = 0.
How do you solve a quadratic equation of the form x² - c = 0?
-You move the constant term c to the right side to get x² = c, then take the square root of both sides to find x = ±√c.
What is the process for solving a quadratic equation when c equals zero?
-You factorize the equation to the form x(x + b) = 0 and then solve for x = 0 or x = -b.
How do you approach solving a quadratic equation when b is not zero and c is zero?
-You find two numbers that multiply to c and add to b, then factorize the equation in the form x + p(x + q) = 0 and solve for x = -p or x = -q.
What should you do if the coefficient of x² is not 1 when solving a quadratic equation?
-First, take out any common factor, then rearrange the equation into the form ax² + bx + c = 0, and solve it accordingly.
Can you provide an example of solving a quadratic equation by taking out a common factor?
-Yes, for the equation 3x² + 6x = 0, the common factor is 3x. After factoring out 3x, the equation becomes x(x + 2) = 0, leading to solutions x = 0 or x = -2.
How do you expand x in a quadratic equation to make it factorizable?
-You find two numbers that multiply to ac (where a is the coefficient of x² and c is the constant) and add to b (the coefficient of x), then rewrite the middle term using these numbers.
What is the formula for completing the square for a quadratic equation?
-The formula is to first add and subtract (b/2)² to the equation, then rewrite it in the form (x + b/2)² = (b/2)² + c.
How do you use the quadratic formula to solve a quadratic equation?
-You use the formula x = (-b ± √(b² - 4ac)) / (2a), where a, b, and c are the coefficients of the quadratic equation ax² + bx + c = 0.
Can you give an example of solving a quadratic inequality using factorization?
-Yes, for the inequality x² + 7x + 10 ≥ 0, you factorize it to (x + 2)(x + 5) ≥ 0. By testing intervals, you find the solution is x ≤ -5 or x ≥ -2.
What is the significance of drawing a number line when solving quadratic inequalities?
-Drawing a number line helps you visualize and test intervals to determine where the inequality holds true, based on the roots of the corresponding quadratic equation.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

SOLVING QUADRATIC EQUATIONS BY COMPLETING THE SQUARE || GRADE 9 MATHEMATICS Q1

Solving Equations Transformable into Quadratic Equations

MATH 9 - Solving Equations Transformable to Quadratic Equation Including Rational Algebraic Equation
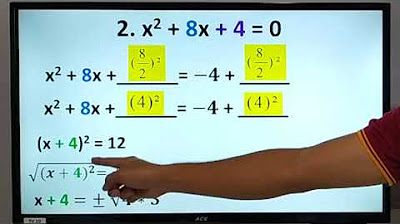
Math8 1G LV4 - Completing the Square and Quadratic Formula

EQUATIONS IN QUADRATIC FORM || GRADE 9 MATHEMATICS Q1

Persamaan Kuadrat Kelas 10 Kurikulum Merdeka
5.0 / 5 (0 votes)