UAD - Kuliah Online 1475530 Karakterisasi Material Lanjut (Lecture 1c)
Summary
TLDRThis transcript delves into the intricacies of X-ray diffraction (XRD) analysis, particularly in relation to materials like iron (α-Fe) and sodium chloride (NaCl). The discussion covers key factors that influence diffraction patterns, such as Miller indices (hkl), structure factors, and intensity variations. It explains the role of structural, temperature, Lorentz, and polarization factors in shaping diffraction results. The conversation also touches on the complexities of different crystal structures, such as FCC and BCC, and how these structures influence observed diffraction intensities. The content is deeply technical, offering insight into the mathematical and physical principles behind X-ray crystallography.
Takeaways
- 😀 The diffraction intensities are influenced by structural factors such as the atomic arrangement and symmetry of the crystal lattice.
- 😀 The Lorentz polarization factor plays a key role in X-ray diffraction, with lower angles resulting in higher intensity diffraction patterns.
- 😀 The structure factor (F_hkl) is determined by the arrangement of atoms in the unit cell and their positions relative to each other.
- 😀 Different crystal structures, such as FCC (Face-Centered Cubic) and BCC (Body-Centered Cubic), result in different diffraction patterns and intensity distributions.
- 😀 In X-ray diffraction, the interaction between the X-rays and the atoms depends on the number of electrons in the atom, affecting the diffraction intensity.
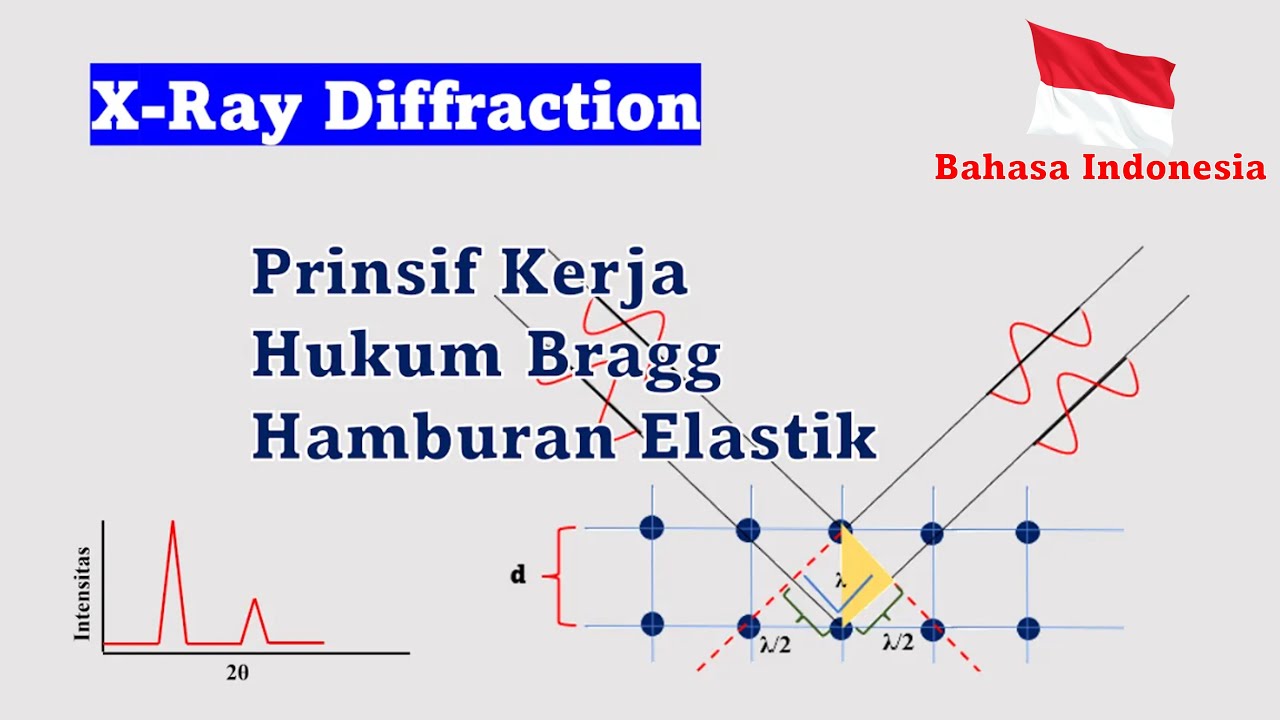
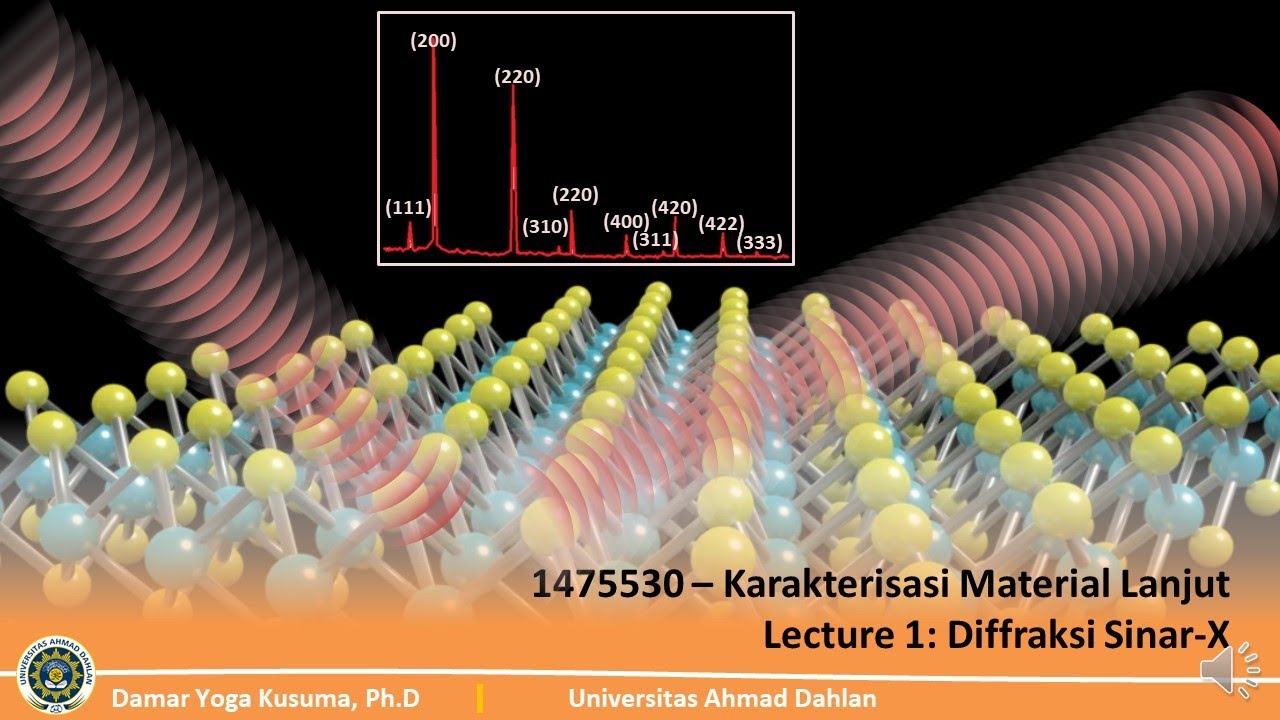
- 😀 FCC crystals, like NaCl, often show diffraction peaks that correspond to specific (hkl) values, such as 200, 111, and 220, depending on whether the indices are odd or even.
- 😀 In the BCC structure, diffraction peaks will only appear for certain (hkl) values, with certain combinations leading to zero intensity, due to phase cancellations.
- 😀 The structure factor can be calculated using the atomic positions within the unit cell, and the sum of exponential terms for each atom in the structure.
- 😀 The diffraction peaks in NaCl (FCC structure) are determined by the arrangement of sodium and chloride atoms, with different diffraction intensities for odd/even (hkl) combinations.
- 😀 The concept of 'multiplicity' explains that some crystallographic planes have more symmetry, leading to higher diffraction intensities. For example, the (100) plane in FCC structures can have multiple equivalent planes, amplifying intensity.
Q & A
What is the structure factor (f_hkl) in X-ray diffraction?
-The structure factor (f_hkl) determines the intensity of diffraction peaks and is based on the arrangement of atoms in the unit cell. It is calculated by summing the scattering factors of atoms in the unit cell, with each atom's position weighted by the exponential of its Miller indices and fractional coordinates.
What is the role of the Lorentz factor in X-ray diffraction?
-The Lorentz factor compensates for the geometrical effects during diffraction, specifically how diffraction intensity varies with the diffraction angle (θ). It accounts for the fact that diffraction is more intense at lower angles, with intensity decreasing as the angle increases.
How does the polarization factor affect diffraction?
-The polarization factor describes how the polarization of X-rays impacts the diffraction pattern. It is a function of the diffraction angle and affects the distribution of intensity across different diffraction peaks.
Why do certain diffraction peaks not appear in the X-ray diffraction patterns of materials?
-Certain diffraction peaks may not appear due to destructive interference, atomic displacement, or the intrinsic structure factor of the material. Additionally, factors like temperature, absorption, and the nature of the atoms involved can influence whether a peak is visible.
What is the significance of Miller indices in diffraction patterns?
-Miller indices (h, k, l) represent the orientation of atomic planes in a crystal lattice. These indices help identify which planes of atoms will diffract X-rays and influence the resulting diffraction pattern, determining the positions of diffraction peaks.
Why do some diffraction peaks appear only for specific combinations of Miller indices?
-The appearance of diffraction peaks is determined by the symmetry and structure of the crystal. Peaks typically appear only when the sum of the Miller indices (h+k+l) is either all odd or all even, which reflects the symmetry and atomic arrangement of the crystal.
What factors influence the intensity of diffraction peaks in X-ray diffraction?
-The intensity of diffraction peaks is influenced by several factors, including the structure factor (which depends on atomic arrangement), the Lorentz and polarization factors, the absorption of X-rays by the material, and the temperature, which affects atomic vibrations.
How do temperature and atomic displacement affect diffraction intensities?
-Temperature causes atoms to vibrate more, which can lead to broader diffraction peaks and reduced intensity. Displacement of atoms from their ideal positions also affects the diffraction pattern by altering the structure factor and leading to lower or absent peaks.
How does the atomic scattering factor (f_n) vary between different elements?
-The atomic scattering factor (f_n) depends on the number of electrons in an atom. Elements with more electrons, such as copper, scatter X-rays more strongly, leading to higher diffraction intensities. In contrast, elements with fewer electrons, such as hydrogen, have smaller scattering factors.
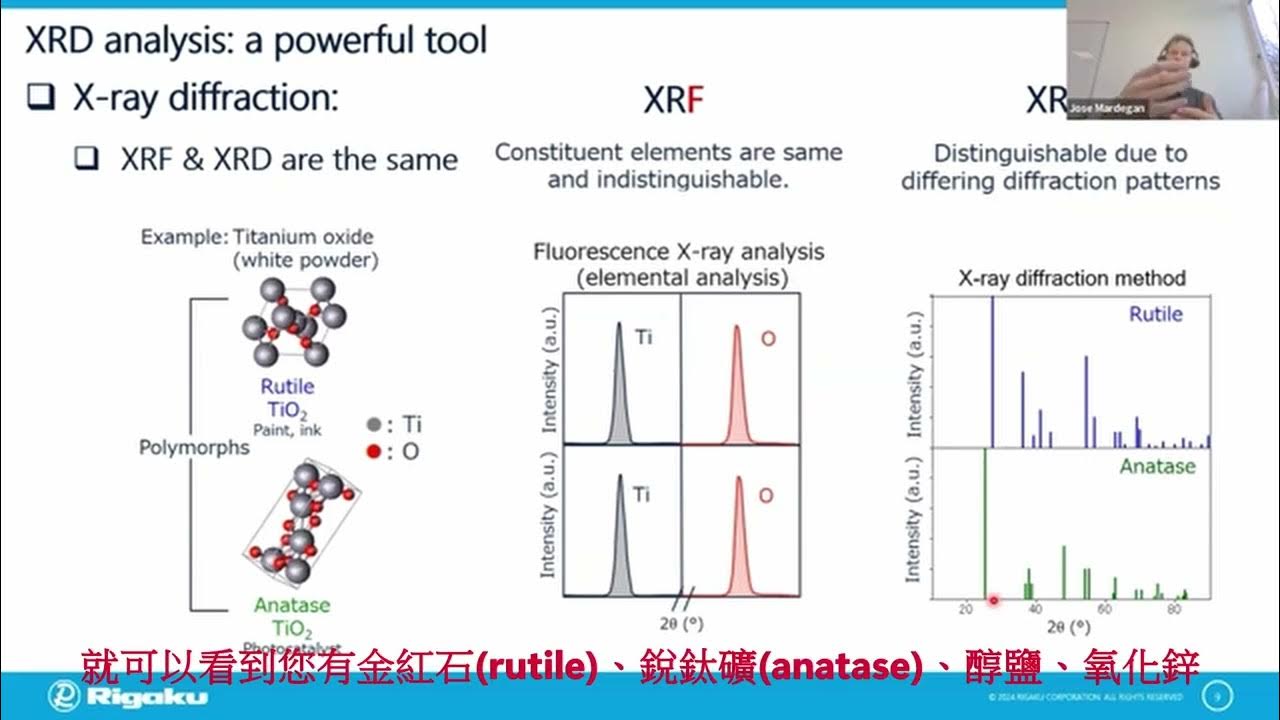
Why do different crystal structures (FCC, BCC, NaCl) produce different diffraction patterns?
-Different crystal structures (FCC, BCC, NaCl) have different atomic arrangements, leading to variations in diffraction patterns. For instance, FCC crystals typically show peaks for Miller indices like (200) and (220), while BCC crystals exhibit different patterns due to their unique atomic coordination and symmetry.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
5.0 / 5 (0 votes)