Introducing Weird Differential Equations: Delay, Fractional, Integro, Stochastic!

Summary

This educational video introduces viewers to four unusual types of differential equations: delay, integral, stochastic, and fractional. Delay differential equations (DDEs) are notable for their dependence on past values of the function. Integral differential equations combine derivatives and integrals of the function. Stochastic differential equations incorporate random fluctuations, while fractional differential equations involve non-integer order derivatives. The video briefly explains these concepts and mentions methods for solving them, such as the method of steps for DDEs and Laplace transforms for integral equations, without going into complex mathematical details.

Takeaways

• 📚 The lesson introduces four types of unusual differential equations not typically covered in early undergraduate courses.
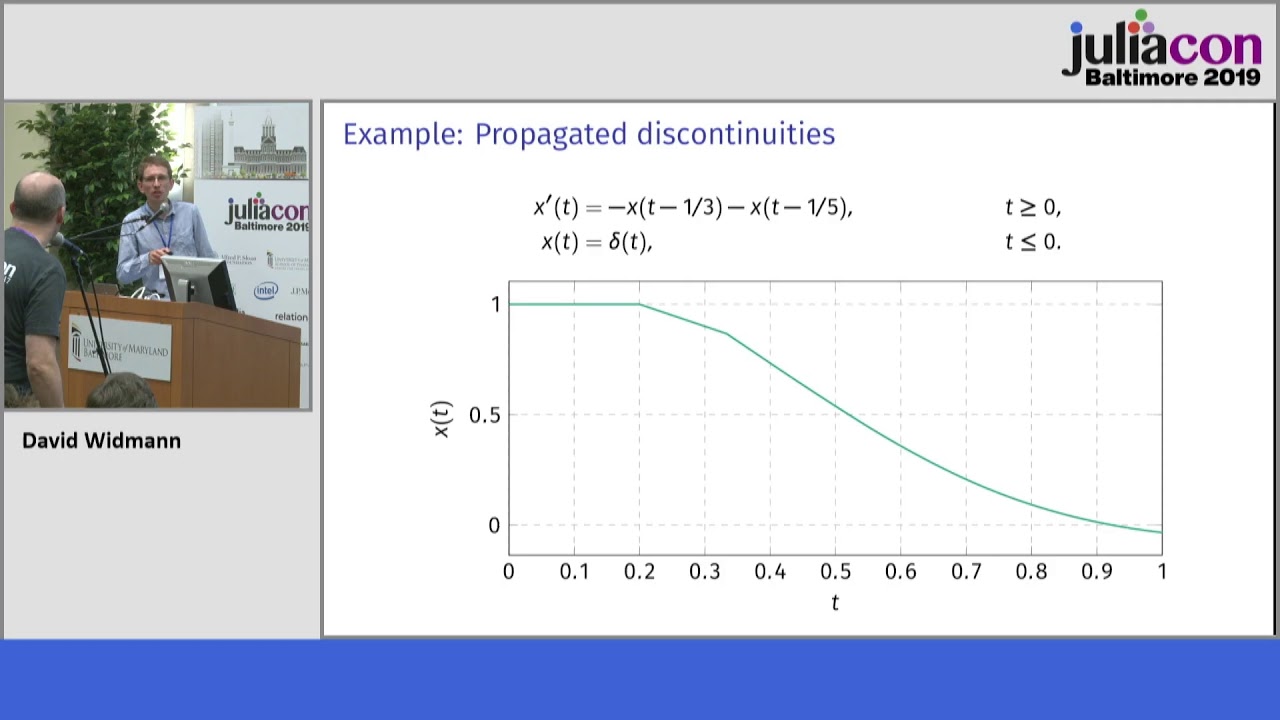
• 🕒 Delay Differential Equations (DDEs) depend on the value of the dependent variable at a previous time, not just the present.
• 🔍 There are three types of DDEs: continuous delay, fixed and discrete delay, and scaled delay, with the latter including the pantograph equation as a special case.
• 📉 Solving DDEs requires specifying an initial function over a time interval, rather than a single initial condition, due to their dependence on past values.
• 👣 The method of steps is a special technique used to solve DDEs with discrete delays by iteratively solving over intervals.
• 🧩 Integral Differential Equations include both derivatives and integrals of the function of interest, and can be solved using integral transforms for simple cases.
• 🔄 Stochastic Differential Equations incorporate random fluctuations or 'white noise' terms, requiring techniques from stochastic calculus and numerical methods for solutions.
• 🎲 Fractional Differential Equations involve fractional derivatives, which are derived from fractional calculus allowing for non-integer orders of differentiation.
• 🎯 Fractional calculus extends the concept of differentiation to allow for taking derivatives of real or even complex numbers, not just integers.
• 👨‍🏫 The video is educational, aiming to introduce and explain these advanced types of differential equations to a presumably upper-level audience.
• 🌟 The video concludes with acknowledgments to patrons and an invitation for viewers to like and subscribe for more content.

Q & A

What are the four types of 'weird' differential equations mentioned in the lesson?

The four types of 'weird' differential equations discussed are delay differential equations (DDEs), integral differential equations, fractional differential equations, and stochastic differential equations.

What is a delay differential equation (DDE)?

A delay differential equation (DDE) is a differential equation that depends on the value of the dependent variable x at a previous time, denoted as x(t-tau), where tau represents the time delay.

Can you explain the three types of delay differential equations?

The three types of DDEs are: 1) Continuous delay DDE, which depends on the sum of all possible past values of x. 2) Fixed and discrete delay DDE, where the delay is a fixed amount of time. 3) Scale delay DDE, which depends on a previous value of x corresponding to lambda times t, where lambda is a constant between 0 and 1.

What is the method of steps used for solving delay differential equations?

The method of steps involves specifying an initial function from negative tau to 0, and then iteratively substituting the function into the DDE to solve for x(t) over successive intervals of tau units of time.

How does an integral differential equation differ from a regular differential equation?

An integral differential equation contains both derivatives and integrals of the function of interest, unlike regular differential equations which only involve derivatives.

What is a stochastic differential equation?

A stochastic differential equation is a differential equation that includes a random fluctuating or stochastic process, often represented by a term involving sigma(x, t) times epsilon(t), where epsilon is a white noise term.

What is fractional calculus and how does it relate to fractional differential equations?

Fractional calculus is a field of study that allows for the taking of non-integer order derivatives, such as half or quarter derivatives. Fractional differential equations involve these fractional derivatives of an unknown function.

Why is it necessary to specify an initial function for a delay differential equation with a discrete delay?

Specifying an initial function is necessary because to determine the value of dx/dt at a future time, one needs the value of x at previous times, which is not provided by just an initial condition at t=0.

How can integral transforms be used to solve integral differential equations?

Integral transforms, such as the Laplace transform, can be used to convert integral differential equations into a form that is easier to solve analytically, especially for simple linear cases.

What techniques are used to solve stochastic differential equations?

Solving stochastic differential equations typically involves using techniques from stochastic calculus in combination with numerical methods.

Outlines

00:00

📚 Introduction to Unusual Differential Equations

This paragraph introduces the topic of the video, which is about four unusual types of differential equations that are typically encountered in research and advanced applied mathematics courses. The instructor explains that these equations are not commonly studied in early undergraduate courses. The four types discussed are delay differential equations (DDEs), integral differential equations, stochastic differential equations, and fractional differential equations. The explanation begins with delay differential equations, emphasizing how they differ from standard differential equations by depending on the value of the dependent variable at a previous time, denoted as 'tau' seconds before the present time 't'. The paragraph also outlines the three types of DDEs: continuous delay, fixed and discrete delay, and scaled delay, with an example of the pantograph equation provided for the scaled delay type.

05:02

🔍 Exploring Advanced Types of Differential Equations

The second paragraph delves into the specifics of solving delay differential equations, emphasizing the necessity of an initial function rather than a single initial condition due to the nature of delays in the equations. The method of steps is introduced as a technique for solving DDEs with discrete delays. The paragraph then transitions to integral differential equations, which involve both derivatives and integrals of the function of interest. It mentions the use of integral transforms like the Laplace transform for solving simpler cases. The discussion moves on to stochastic differential equations, which incorporate a random process represented by white noise. Solving these requires stochastic calculus and numerical methods. Lastly, the paragraph touches on fractional differential equations, providing a brief introduction to fractional calculus, which allows for the calculation of non-integer order derivatives. The video concludes with acknowledgments and a sign-off from the instructor.

Keywords

💡Differential Equations

Differential equations are mathematical equations that involve a function and its derivatives. They are used to describe the behavior of changing phenomena and are central to the field of calculus. In the video, differential equations are the main theme, with a focus on 'weird' types that are not commonly studied in early undergraduate courses but are important in research and advanced applied mathematics.

💡Delay Differential Equations (DDEs)

Delay differential equations are a type of differential equation where the rate of change of the function depends not only on the current value but also on past values. The video introduces DDEs as equations that include a delay term, such as x(t-τ), where τ represents a time in the past. This concept is illustrated with examples like the pantograph equation, which is a special case of a DDE.

💡Integral Differential Equations

Integral differential equations are equations that contain both derivatives and integrals of the function of interest. They are highlighted in the video as a 'weird' type that combines the rate of change (differentiation) and the accumulation (integration) of a quantity. An example given in the script is an equation where the derivative of x with respect to t is influenced by an integral involving another function g.

💡Fractional Differential Equations

Fractional differential equations involve derivatives of non-integer orders, which is a concept from fractional calculus. The video explains that these equations allow for taking 'fractions' of derivatives, such as a half or a quarter derivative, using techniques that generalize the standard differentiation process. This type of equation is used to model phenomena that exhibit memory or hereditary properties.

💡Stochastic Differential Equations

Stochastic differential equations are differential equations that include a random component, often represented by white noise. The video describes these equations as ones that depend on a stochastic process, which introduces randomness into the model. Solving them typically requires techniques from stochastic calculus and numerical methods.

💡Dynamical Systems

Dynamical systems are a framework in mathematics for understanding how systems evolve over time. In the context of the video, dynamical systems are mentioned in relation to differential equations, which are used to model the behavior of such systems. The script discusses how traditional differential equations depend on current values, while delay differential equations also consider past values.

💡Initial Function

In the context of solving delay differential equations, an initial function is necessary to provide the values of the dependent variable over a certain interval in the past, rather than just a single initial condition. The video explains that specifying an initial function is crucial because it allows the solution of the DDE to be determined over time, by providing the past values required by the delay.

💡Method of Steps

The method of steps is a technique for solving differential equations, particularly those with initial conditions that are given over an interval rather than at a single point. The video describes this method in the context of solving DDEs, where the initial function is used to iteratively solve for the function values over successive intervals.

💡Fractional Calculus

Fractional calculus is a field of mathematical analysis that generalizes the concept of differentiation and integration to arbitrary (non-integer) orders. The video provides a brief introduction to fractional calculus as a way to understand fractional differential equations, where derivatives of real or even complex orders are considered.

💡White Noise

White noise, in the context of stochastic differential equations, represents a random fluctuating process that is often modeled as having constant power spectral density. The video mentions white noise in the form of ε(t), which is multiplied by a function of x and t to create the stochastic component of the equation.

Highlights

Introduction to four unusual types of differential equations: delay, integral, fractional, and stochastic differential equations.

Delay differential equations (DDEs) depend on the value of the dependent variable at a previous time, not just the present.

Three types of DDEs: continuous delay, fixed and discrete delay, and scaled delay.

The method of steps is used to solve DDEs with discrete delays by specifying an initial function.

Integral differential equations involve both derivatives and integrals of the function of interest.

Integral transforms like the Laplace transform can be used to solve simple integral differential equations.

Stochastic differential equations incorporate random fluctuations or stochastic processes.

Solving stochastic differential equations requires techniques from stochastic calculus and numerical methods.

Fractional differential equations involve fractional derivatives, which are not limited to rational orders.

Fractional calculus allows for the computation of derivatives that are not whole numbers, such as half or quarter derivatives.

The video aims to introduce the concepts of these unusual differential equations without going into detailed solutions.

The importance of specifying an initial function for DDEs due to their dependence on past values.

The Pantograph equation as a special type of scaled delay differential equation.

Higher order integral and partial integral differential equations are also possible beyond first order.

The inclusion of white noise terms in stochastic differential equations to represent randomness.

The use of the gamma function in fractional calculus to compute fractional derivatives.

An acknowledgment of patrons supporting the educational content creation.

Transcripts

greetings students and welcome to my

lesson on weird types of differential

equations

you don't usually study these types of

differential equations in early or even

intermediate undergraduate courses

they tend to come up in areas of

research and upper level courses on

applied math

now by weird differential equations i'm

really going to talk about

four weird types of differential

equations delay differential equations

integral differential equations

fractional differential equations and

stochastic differential equations

we'll start by discussing delay

differential equations

when you look at dynamical systems

consisting of a single differential

equation

you conventionally think of an equation

like dx by dt

equals f of x comma t the differential

equation depends on the value of t

and the value of x at the present time

at the current time

however what if in addition to my

differential equation depending on

x at the present time it also depended

on the value of x

at a previous time let's say tau seconds

before the present time t

in that case we could write our

differential equation as dx by dt

equals a function f of x

at the time t x at the time t minus tau

and t where x of t minus tau represents

the value of x that occurred tau

seconds earlier this differential

equation which depends on the value of

the dependent variable x

occurring earlier is called a delay

differential equation

or dde a delay differential equation dx

by dt

is a differential equation that depends

on the value of x

sometime in the past now what i've

written up here and called the delay

differential equation

isn't the most general way to describe a

delay differential equation

in fact there's actually three types of

ddes the first type is a dde with

continuous delay

this dde depends on x t and the sum of

all possible past values of x and if you

want to sum so many values then in

general you can write that as an

integral

the integral from negative infinity to

zero of x of t

plus tau times d mu by d tau with

respect to tau

where mu is some function of the delay

tau

the second type of dde is the type we

wrote above where the delay is

fixed and discrete of course you're not

restricted to a single delay you can

have multiple delays in your system

tau 1 tau 2 all the way to tau n

the third type is one in which you have

a scale delay now what do i mean by this

basically your dde depends on x of t

the value of x at time t as well as a

previous

value of x corresponding to lambda times

t where lambda is a constant between 0

and 1.

a special type of this equation is

called the pantograph equation given by

dx by dt equals a times x of t

plus b times x of lambda times t where a

and b are some real constants now how do

you solve

a delay differential equation i won't go

through a complete example here but i'll

briefly illustrate what the process

looks like

say i have a dde with a discrete delay

tau like the simple dde i described

earlier on in the video

i'll call this equation 1. to start

solving this dde

i need to first specify an initial

function from negative tau to 0

as opposed to an initial condition at t

equals 0 for instance

and why do i need to specify this

initial function let me explain this on

the side

say i had to solve this dde and i only

had the value of x

at time 0 as my initial condition

suppose also that my delay tau was

5. then in order to determine the value

of dx by dt and by extension

x of t at the time 1 i would need the

value of x

at time negative four according to this

dde

however i only have the value of x at

zero i don't have the value

at negative four so specifying x at zero

is simply

not enough to solve this dde i need to

specify what the entire function looks

like from negative 5 to 0

which is why i need an initial function

as my initial condition

instead of an initial point so given

this dde

an initial function what we can do is

substitute phi of t minus tau for

x of t minus tau into equation one with

t minus tau varying from negative tau to

zero

then with this function plugged in we

can solve for x of t

from t equals zero to t equals tau and

once we obtain

x of t from zero to tau we can then plug

that in as x

of t minus tau and then solve for x of t

from tau to two tau

then we can keep repeating these steps

plugging in our previous

x in place of the x of t minus tau and

using that to solve the differential

equation and obtain a full value of x

that's tau

units of time in the future this full

method by the way is given a special

name it's called

the method of steps another type of

weird differential equation we'll go

over is the integral differential

equation

as the name implies this type of

differential equation contains both the

derivatives of the function of interest

and the integrals of the function of

interest

for instance if i have a first order

differential equation in terms of dx by

dt

an integral differential equation could

look something like this where dx by dt

is f of x comma t plus an integral term

from t

naught to t of another function g of s

comma

x d s except for really simple cases

it's not that easy to solve integral

differential equations by hand

but in cases when you can determine the

solution analytically like

simple linear differential equations you

can use integral transforms like the

laplace

transform to find the answer i should

also note that we don't necessarily need

to have a first order integral

differential equation

we can just as well have second or

higher order or even partial

integral differential equations the next

type of weird differential equation

we'll discuss

is the stochastic differential equation

consider our typical differential

equation

dx by dt equals some function of x and t

if i add a term sigma of x and t times

an epsilon of t

where epsilon represents a white noise

term then i get something called a

stochastic differential equation

which is a differential equation that

depends on some random fluctuating or

stochastic process

solving stochastic differential

equations involves using techniques

developed in stochastic calculus in

combination with numerical methods

we won't go over those here since our

goal with this video is just to

introduce these

weird types of differential equations

and the last type of differential

equation i'll discuss is a fractional

differential equation

as the name suggests this is a

differential equation which involves the

fractional derivatives of an unknown

function

to see what this means let me give a

very brief pep talk on fractional

calculus

now in typical calculus you have a

function f of t

and you can take its derivative df by dt

or even a higher order derivative

like the d2f by dt squared which is the

second derivative

but what if i didn't want to fully

commit to taking a derivative

for instance what if i wanted to take a

fraction of a derivative

well i can do that using fractional

calculus

fractional calculus allows me to take a

half derivative or a quarter derivative

even if i wanted to

in general using a combination of clever

manipulations in the gamma function i

can compute

fractional derivatives like the alpha

derivative of f with respect to t

where alpha is some real number between

0 and 1.

in fact it could also be a complex

number so even though it says

fractional calculus i'm not necessarily

restricted to derivatives of a rational

order

so a fractional differential equation is

then a differential equation which

involves these fractional derivatives

anyway that should do it for this brief

video i'd like to thank the following

patrons for supporting me at the five

dollar level or higher and if you

enjoyed the video feel free to like and

subscribe

this is the faculty of khan signing out