Pre-Calculus 12 - Video #32: Trigonometric Equations

Summary

This precalculus lesson focuses on solving trigonometric equations, with a step-by-step approach to algebraic manipulation, reference angles, and periodic functions. The instructor covers various types of trigonometric equations involving sine, cosine, tangent, and secant. Key concepts include identifying reference angles, understanding quadrant placements, factoring, and solving for general solutions with periodicity. As the lesson progresses, it introduces more complex scenarios, including equations with multiple trig functions, and emphasizes the importance of considering rotations and factoring tricks. The video is designed to help students master these essential skills and build confidence in solving trigonometric equations.

Takeaways

• 😀 Understand the importance of algebraic manipulation in solving trigonometric equations, like isolating the sine, cosine, or tangent terms before finding solutions.
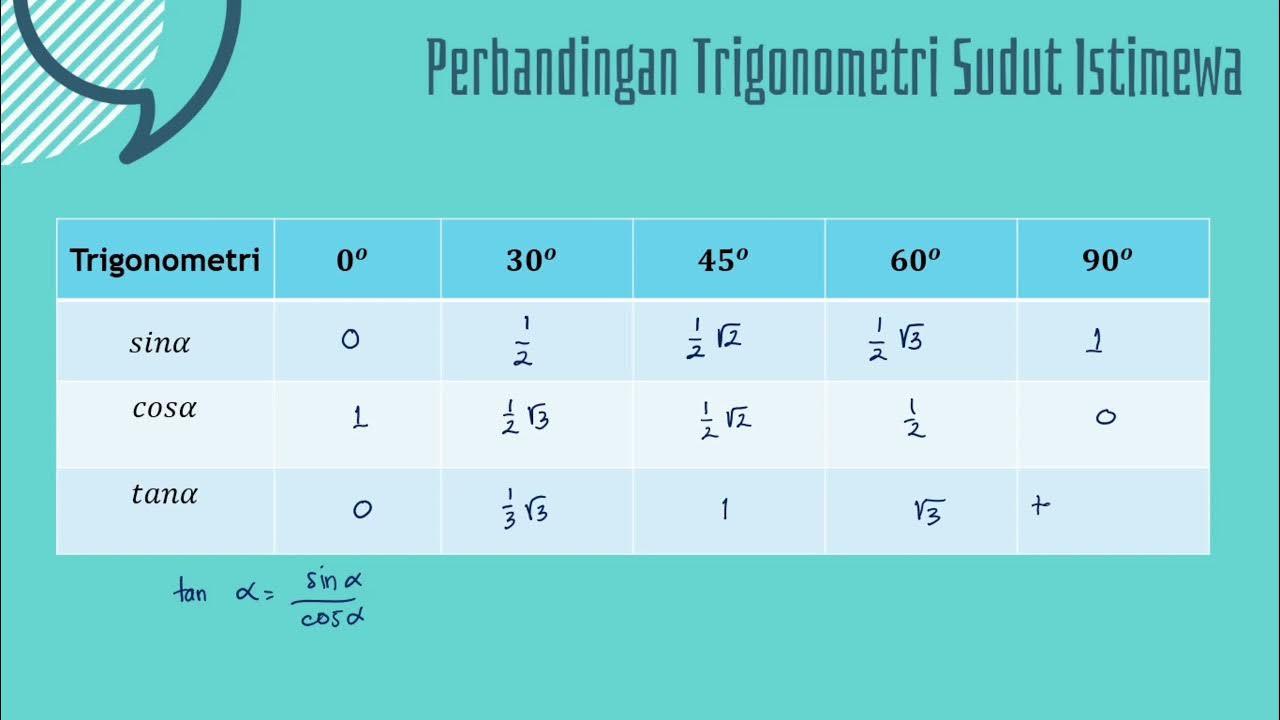
• 😀 Identifying reference angles is crucial to solving trigonometric equations—recognizing when sine, cosine, or tangent take on specific values (like 1/2 or -√3/2) helps determine solutions quickly.
• 😀 Solutions for trigonometric equations depend on the quadrant where the function is positive or negative, based on the unit circle and the nature of sine, cosine, and tangent.
• 😀 Trigonometric functions like sine, cosine, and tangent are periodic, so their solutions repeat after certain intervals. For sine and cosine, the period is 2π, while for tangent, it's π.
• 😀 General solutions include adding multiples of the period to each specific solution, allowing for all possible solutions over infinite rotations.
• 😀 When solving for cosine and sine, the value of the angle may result in multiple possible solutions, corresponding to different quadrants and reference angles.
• 😀 For tangent equations, solutions are simpler because the period is π, and this affects the general solution format, which does not require adding 2πn.
• 😀 For more complex equations involving multiple trigonometric functions (like sine and tangent together), start by factoring and isolating terms to simplify the equation.
• 😀 Special cases like equations with higher multiples of the angle (e.g., 2x or 3x) require adjusting the period of the function, which affects the number of rotations and, consequently, the number of solutions.
• 😀 Understanding and using trigonometric identities, such as sin²θ + cos²θ = 1, helps transform equations and makes solving easier, especially when mixed functions like secant and cosine appear.

Q & A

What is the first step in solving the equation sin(2x) - 1 = 0?

The first step is to isolate the sine function. To do this, add 1 to both sides of the equation, then divide both sides by 2 to get sin(x) = 1/2.

Which quadrants do the solutions for sin(x) = 1/2 exist in?

The solutions for sin(x) = 1/2 exist in quadrant I and quadrant II because sine is positive in these quadrants.

How do you find the reference angle for sin(x) = 1/2?

The reference angle is 30 degrees (or π/6 radians) because sin(30°) = 1/2.

How do you write the general solution for sin(x) = 1/2?

The general solution is written as x = π/6 + 2πn and x = 5π/6 + 2πn, where n is any integer, to account for multiple rotations.

How do you solve the equation cos(x) + √2 = -cos(x)?

First, add cos(x) to both sides and subtract √2 from both sides, resulting in 2cos(x) = -√2. Then divide both sides by 2 to get cos(x) = -√2/2.

Which quadrants do the solutions for cos(x) = -√2/2 exist in?

The solutions for cos(x) = -√2/2 exist in quadrant II and quadrant III, since cosine is negative in these quadrants.

What is the reference angle for cos(x) = -√2/2?

The reference angle is π/4 because cos(π/4) = √2/2, and cosine of -√2/2 corresponds to angles in quadrant II and quadrant III.

How do you express the general solution for cos(x) = -√2/2?

The general solution is x = 3π/4 + 2πn and x = 5π/4 + 2πn, where n is any integer.

In the equation tan(x) = -√3, why do the solutions exist in quadrants II and III?

The solutions for tan(x) = -√3 exist in quadrants II and III because tangent is negative in these quadrants, while √3 is positive.

What is the general solution for tan(x) = -√3?

The general solution is x = 2π/3 + πn and x = 4π/3 + πn, where n is any integer. This accounts for the periodic nature of the tangent function with a period of π.

How do you solve the equation sin(x)tan(x) = 2tan(x)?

First, subtract 2tan(x) from both sides to get sin(x)tan(x) - 2tan(x) = 0. Then factor out tan(x), resulting in tan(x)(sin(x) - 2) = 0. Solve each factor separately. tan(x) = 0 gives x = 0, π, and sin(x) - 2 = 0 is not possible since sine cannot be greater than 1.

What is the period of the secant function and how does it affect the general solution?

The period of the secant function is the same as cosine, 2π. For secant equations, the general solution involves adding multiples of 2π to each solution to account for all possible rotations.

How do you solve a quadratic secant equation like sec(x)² - sec(x) - 2 = 0?

Factor the equation into (sec(x) - 2)(sec(x) + 1) = 0. Then, solve for each factor. sec(x) = 2 corresponds to cos(x) = 1/2, and sec(x) = -1 corresponds to cos(x) = -1. Solve each for the respective quadrants and write the general solution using 2πn for each case.

Why do some trigonometric equations involve adjusting the period for multiple rotations (e.g., 3x)?

When the variable is multiplied by a factor (e.g., 2x, 3x), the period of the trigonometric function changes. For instance, when the equation involves 3x, the period is reduced to 2π/3. This means more rotations fit within one cycle, and additional solutions must be considered to account for multiple periods within a single cycle.