INTEGRACIÓN por PARTES | Integrales Cíclicas
Summary
TLDRIn this video, Professor Andalón explains the process of solving an integral involving the function e^x multiplied by sin(x), using integration by parts. He introduces the mnemonic method 'YAT' to help identify which part of the equation should be set as 'u' and which as 'dv'. Through step-by-step calculations, he demonstrates how to derive the solution by applying integration by parts twice. The professor highlights the importance of recognizing specific function types, such as trigonometric and algebraic, in simplifying the process. The video concludes with practical advice on mastering integration techniques, preparing viewers for more complex calculus methods.
Takeaways
- 😀 The integration technique of integration by parts is introduced, where a product of two functions is involved, such as e^x and sin(x).
- 😀 To apply integration by parts, select one function as 'u' and the other as 'dv', using a mnemonic strategy like 'LIATE' to choose the function to differentiate first.
- 😀 The mnemonic 'LIATE' suggests starting with the function that fits in the following order: Logarithmic, Inverse trigonometric, Algebraic, Trigonometric, and Exponential functions.
- 😀 In this example, sin(x) is identified as the function to differentiate (u), while e^x dx is the remaining part of the integrand (dv).
- 😀 After identifying the functions for u and dv, the integration by parts formula is used, which is ∫ u dv = u v - ∫ v du.
- 😀 The process involves differentiating and integrating the functions accordingly: sin(x) becomes cos(x) (with a positive sign), and e^x dx integrates to e^x.
- 😀 Once the values for 'u' and 'v' are known, they are substituted into the integration by parts formula, leading to a more manageable integral.
- 😀 In case the new integral is still complex, the method of integration by parts is applied again to further simplify the expression.
- 😀 Repeated application of integration by parts leads to a system where the integrals eventually cancel each other out, simplifying the original expression.
- 😀 The final result for the integral of e^x * sin(x) dx is derived as (e^x sin(x) - e^x cos(x)) / 2, showcasing a neat resolution of the integral through strategic steps.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Integration By Parts
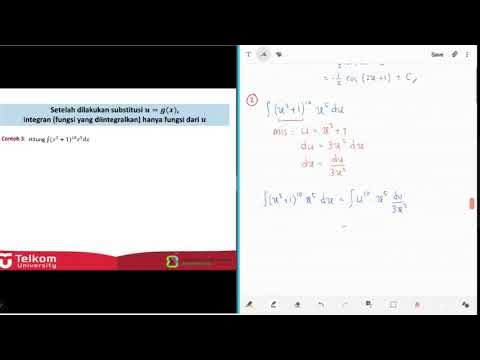
Kalkulus | Integral (Part 2) - Integral Substitusi [2]

Integración por partes | Ejemplo 3.2 Logaritmo natural

Integral de una división de polinomios (paso a paso)

UJAR KALKULUS 2 PERTEMUAN 9 INTEGRAL PARSIAL TAK TENTU
Inverse Functions
The Dirichlet Integral is destroyed by Feynman's Trick
5.0 / 5 (0 votes)