Inverse Functions
Summary
TLDRProfessor Dave explores the concept of inverse functions, illustrating how they reverse the output of a function to its original input. He explains the process of finding an inverse function by swapping 'x' and 'y' and solving for 'y'. Examples are used to demonstrate how to algebraically manipulate expressions to find inverses, emphasizing the need for the domain and range to switch places. The video also highlights the verification of inverse functions through the identity F(F^-1(x)) = x and F^-1(F(x)) = x. Finally, Dave discusses the geometric interpretation of inverse functions as reflections across the line y=x.
Takeaways
- 🔄 Inverse functions are designed to reverse the effect of the original function, allowing you to return to the initial input when applied.
- 📐 The process of finding an inverse involves algebraic manipulation, typically swapping F(x) and x and then solving for y.
- ↔️ The domain and range of the original function become interchanged in its inverse, reflecting the relationship between input and output.
- 🔍 To verify an inverse, apply both the original function and its inverse to a value and check if you retrieve the initial value.
- 📘 If a function does not allow for solving for y after swapping x and y, it does not have an inverse function.
- 🔢 For a function like y = x + 2, the inverse is found by subtracting 2 from both sides, resulting in y = x - 2.
- 🔄 Demonstrating the inverse relationship involves showing that F(F^{-1}(x)) = x and F^{-1}(F(x)) = x hold true.
- 📊 The graphical interpretation of finding an inverse is to reflect the graph of the function across the line y = x.
- 📈 For more complex functions, like y = 4x - 5, the inverse involves algebraic steps such as adding 5 and dividing by 4.
- 🧮 Even for non-linear functions like y = x^3 + 1, finding the inverse involves algebraic operations like subtracting 1 and taking the cube root.
Q & A
What is an inverse function?
-An inverse function is a function that, when applied to the output of the original function, returns the input value. In other words, if you apply the original function to a number and then apply the inverse function to the result, you should get the original number.
How do you find the inverse of a function?
-To find the inverse of a function, you swap the roles of the dependent and independent variables (usually X and Y) and then solve the resulting equation for Y. The new function you obtain is the inverse function.
Why is the inverse of a function not simply the reciprocal of the original function?
-The inverse of a function is not the reciprocal because it involves reversing the operations of the original function, rather than just taking the reciprocal. The inverse function undoes every operation performed by the original function.
How can you check if two functions are inverses of each other?
-To check if two functions are inverses, you can compose them in both possible orders (F of F inverse and F inverse of F). If both compositions return the original input (X), then the functions are inverses of each other.
What does it mean if a function does not have an inverse?
-If a function does not have an inverse, it means that after swapping the variables and attempting to solve for Y, you find that it's impossible to express Y as a function of X. This usually happens if the function is not one-to-one, meaning it does not have a unique output for every input.
How is the domain of a function related to the range of its inverse?
-The domain of the original function is equal to the range of its inverse, and the range of the original function is equal to the domain of its inverse. This is because the input-output pairs are swapped between the function and its inverse.
What is the geometric relationship between a function and its inverse on a graph?
-The graph of an inverse function is a reflection of the graph of the original function across the line Y equals X. This is because swapping X and Y in the function is equivalent to reflecting all points across this line.
What is the inverse of the function F(x) = x + 2?
-The inverse of the function F(x) = x + 2 is F inverse of X = X - 2. This is because the inverse must undo the operation of adding 2, which is achieved by subtracting 2.
How would you find the inverse of the function F(x) = 4x - 5?
-To find the inverse of F(x) = 4x - 5, you would swap X and Y, giving X = 4Y - 5. Then, you solve for Y by adding 5 to both sides and dividing by 4, resulting in Y = (X + 5) / 4. Therefore, the inverse function is F inverse of X = (X + 5) / 4.
What is a key characteristic of the inverse of the function F(x) = x^3 + 1?
-The inverse of the function F(x) = x^3 + 1 is found by swapping X and Y, leading to X = Y^3 + 1. Solving for Y involves subtracting 1 and then taking the cube root, resulting in F inverse of X = cube root of (X - 1). This inverse function reverses the cubic operation and the addition.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
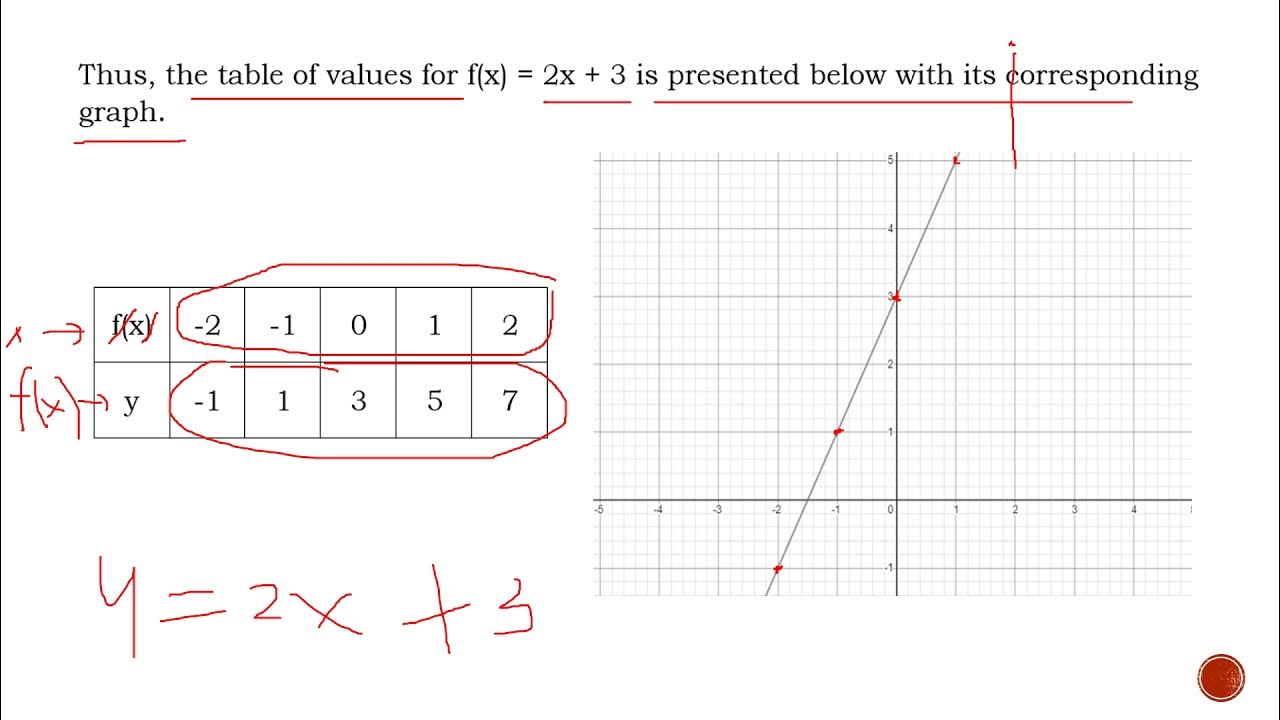
REPRESENTATIONS OF AN INVERSE FUNCTIONS | General Mathematics | Quarter 1 - Module 13

Verifying inverse functions by composition | Mathematics III | High School Math | Khan Academy
Back to Algebra: What are Functions?

FUNGSI INVERS KELAS 11, KONSEP DAN CONTOH SOAL mudah dipahami

FUNGSI | Bab Berpikir Komputasional | Materi Informatika SMP kelas 8 | Fase D

Fungsi Invers Part 1 ||Materi, Soal & Pembahasan||
5.0 / 5 (0 votes)