Penerapan Turunan dalam kehidupan sehari-hari
Summary
TLDRThis video introduces the concept of derivatives and their applications in everyday life. It explains that derivatives measure how a function changes as its input changes, and the process of finding derivatives is called differentiation. The video provides real-world examples, such as calculating the maximum height of a projectile or determining the speed of a firework at a given time. These examples demonstrate how derivatives help analyze motion and change. The video concludes with an example of a rocket's parabolic trajectory and finding its maximum height using differentiation.
Takeaways
- 👋 Introduction of Luqmanulhakim and Sriratu from the Computer Systems department.
- 📈 Explanation of derivatives as a measurement of how a function changes with respect to input values.
- 🔍 Derivatives show how one quantity changes as a result of changes in another quantity.
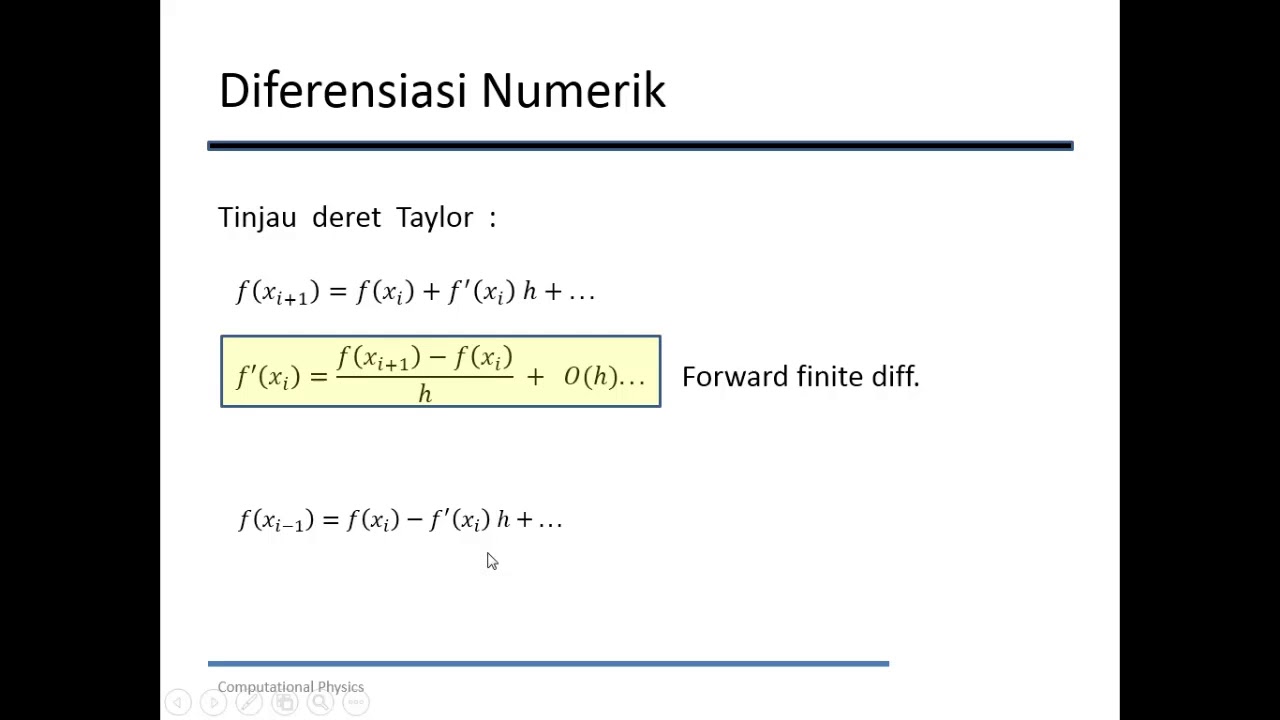
- 📝 The process of finding derivatives is called differentiation.
- 🚀 Example: A bullet is fired vertically, and its height over time is modeled to find the maximum height.
- ⏱ The time to reach the maximum height is calculated to be 25 seconds.
- 🎆 Another example involves the speed of fireworks at a specific time, modeled using a quadratic equation.
- 💥 The height of the firework is modeled as a quadratic function, and the velocity at t=5 is sought.
- 🛠 A third example discusses a rocket's parabolic path and how its maximum height is determined.
- 🏔 The rocket's maximum height is calculated to be 400 meters, reached at 4 seconds.
Q & A
What is the basic definition of a derivative as described in the video?
-A derivative is a measurement of how a function changes as the input values change. In general, it shows how one quantity changes as a result of changes in another quantity.
What is the process called to find the derivative of a function?
-The process of finding a derivative is called differentiation.
What is the given equation for the height of a projectile (peluru) in the first example?
-The equation for the height of the projectile (peluru) is h(t) = 200t - 25t², where 'h' represents height in meters and 't' represents time in seconds.
How do you determine the time to reach the maximum height of the projectile?
-To determine the time to reach maximum height, differentiate the height function and set the derivative equal to zero. The video provides the answer as 25 seconds.
In the second example involving fireworks, what is the equation modeling the height of the fireworks?
-The equation modeling the height of the fireworks is given as h(t) = 150t² + 150.
How would you find the velocity of the fireworks at t = 5 seconds?
-The velocity is the derivative of the height function. To find the velocity at t = 5 seconds, differentiate the height equation and substitute t = 5 into the derivative.
What is the maximum height reached by the rocket in the third example, and how is it calculated?
-The maximum height reached by the rocket is calculated using the equation h(t) = 200t - 25t². The maximum height occurs at t = 4 seconds, and the height is found to be 400 meters.
What kind of trajectory does the rocket follow in the third example?
-The rocket follows a parabolic trajectory, as indicated by the quadratic equation h(t) = 200t - 25t².
What is the significance of setting the derivative equal to zero in these examples?
-Setting the derivative equal to zero helps to find the critical points, which are the points where the maximum or minimum values occur, such as the maximum height of a projectile.
Why is differentiation useful in real-life applications such as those discussed in the video?
-Differentiation is useful because it allows us to calculate rates of change, optimize values (such as maximum height), and better understand how variables interact in physical scenarios like projectile motion or the launch of fireworks.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
5.0 / 5 (0 votes)