Rotasi Hal 34-36 Bab 1 TRANSFORMASI FUNGSI Kelas 12 SMA SMK Kurikulum Merdeka
Summary
TLDRThis educational video covers the concept of function transformation, specifically focusing on rotation. It introduces the rotation of a quadratic function graph, initially in blue, by 90 degrees to the right, resulting in a red graph. The video explains how points A (4,2) and B (9,3) on the original graph transform into A' (-2,4) and B' (-3,9) after rotation. It uses the rotation formula x'y' = cos(α) - sin(α) sin(α) cos(α) * xy, with α being the angle of rotation. The video also demonstrates how to apply this formula to rotate points and provides an example of rotating an exponential function graph by 90 degrees clockwise.
Takeaways
- 📚 The lesson focuses on function transformations, specifically rotation transformations as per the Merdeka curriculum for 12th-grade students in SMA and SMK.
- 📊 The script describes the rotation of a quadratic function graph to the right, transforming from a blue graph to a red one.
- 🔵 The original blue graph represents the function y = x^2, and the rotated red graph represents y = x.
- 🗺️ Points A (4,2) and B (9,3) are given on the original graph, and after a 90-degree rotation, they become A' (-2,4) and B' (-3,9) respectively.
- 🔄 The rotation formula is defined as x' = -y cos(α) - x sin(α) and y' = x cos(α) - y sin(α), where α is the angle of rotation.
- ⏲️ The direction of rotation is important; counterclockwise rotation results in a positive α, while clockwise rotation results in a negative α.
- 📐 The script provides a step-by-step calculation for rotating point A (4,2) by 90 degrees counterclockwise to get A' (-2,4).
- 📘 The lesson applies the rotation formula to a specific example involving the exponential function y = 2^x + 1.
- 🔢 For the exponential function, two points are considered: when x = -1, the point is (-1,1), and when x = 1, the point is (1,4).
- 🔄 After rotation, the point (-1,1) becomes (1,1) and the point (1,4) becomes (4,-1), showing the effect of a 90-degree clockwise rotation.
Q & A
What is the main topic of the video script?
-The main topic of the video script is the study of function transformations, specifically focusing on rotation.
What educational material is the script based on?
-The script is based on a curriculum package for students in grade 12 of SMA or SMK, specifically on pages 34 to 35.
What is the initial function represented by the blue graph in the script?
-The initial function represented by the blue graph is a quadratic function, y = x^2.
How is the blue graph transformed according to the script?
-The blue graph, which represents the function y = x^2, is rotated 90 degrees to the right.
What is the result of rotating the point A(4,2) by 90 degrees as described in the script?
-After rotating point A(4,2) by 90 degrees, it becomes A'(-2,4).
What is the general formula for rotating a point (x, y) by an angle α?
-The general formula for rotating a point (x, y) by an angle α is given by the transformation x' = x cos(α) - y sin(α) and y' = x sin(α) + y cos(α).
What is the significance of the direction of rotation in the context of the script?
-In the script, the direction of rotation is significant because it determines the sign of the angle α. A rotation in the clockwise direction (opposite to the direction of the clock's hands) results in a negative α, while a counterclockwise rotation results in a positive α.
How is the rotation of the point B(9,3) described in the script?
-The rotation of point B(9,3) is described by using the rotation matrix to find the new coordinates B'(-3,9) after a 90-degree rotation.
What is the example problem presented in the script?
-The example problem in the script involves rotating the exponential function y = 2^x + 1 by 90 degrees in the clockwise direction.
How are the coordinates of a point transformed when rotating by 90 degrees clockwise according to the script?
-When rotating a point by 90 degrees clockwise, the transformation involves using the rotation matrix where x' = -y and y' = x.
What is the final outcome of rotating the exponential function as described in the script?
-The final outcome of rotating the exponential function y = 2^x + 1 by 90 degrees clockwise is a new graph where the original point A(-1,1) becomes A'(1,1) and point B(1,4) becomes B'(4,-1).
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

ASYIK! Rumus ROTASI. TRANSFORMASI FUNGSI. Matematika Kelas 12 [SMA]

Transformasi Geometri Bagian 3 - Rotasi (Putaran) Matematika Wajib Kelas 11

Transformasi gabungan
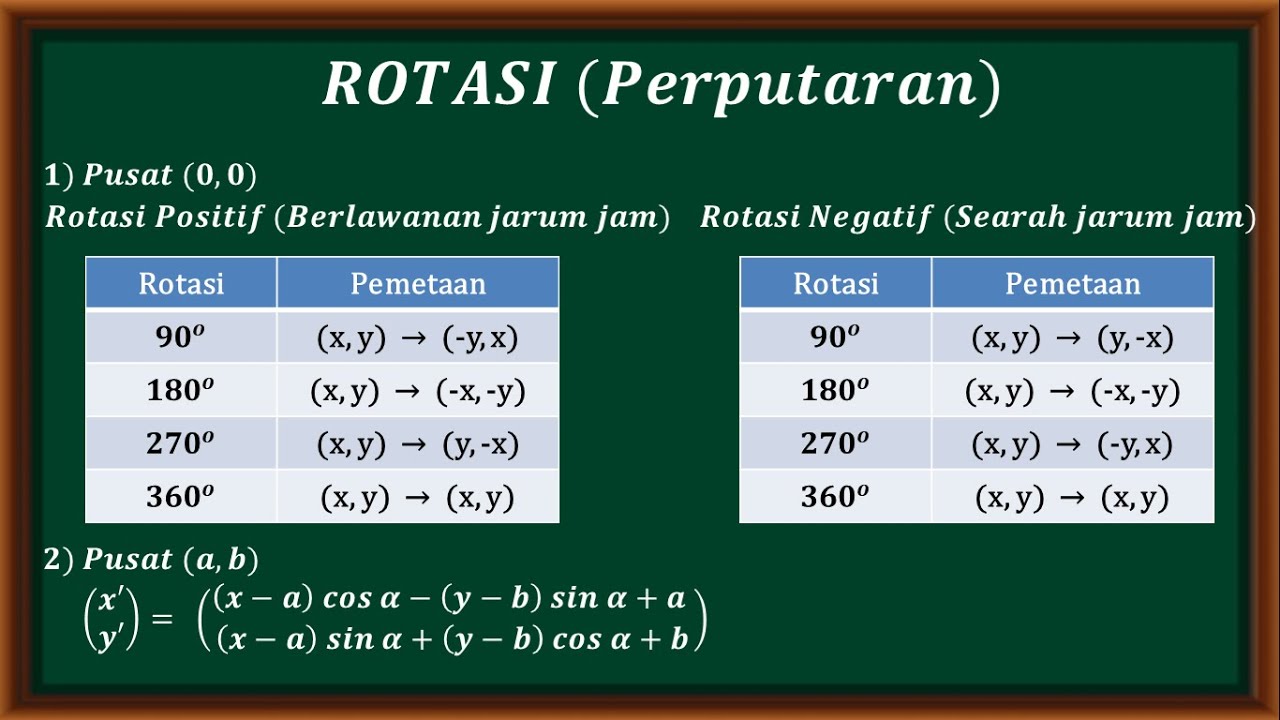
ROTASI (Perputaran) - Cara menentukan bayangan titik di pusat (0,0) dan (a,b)
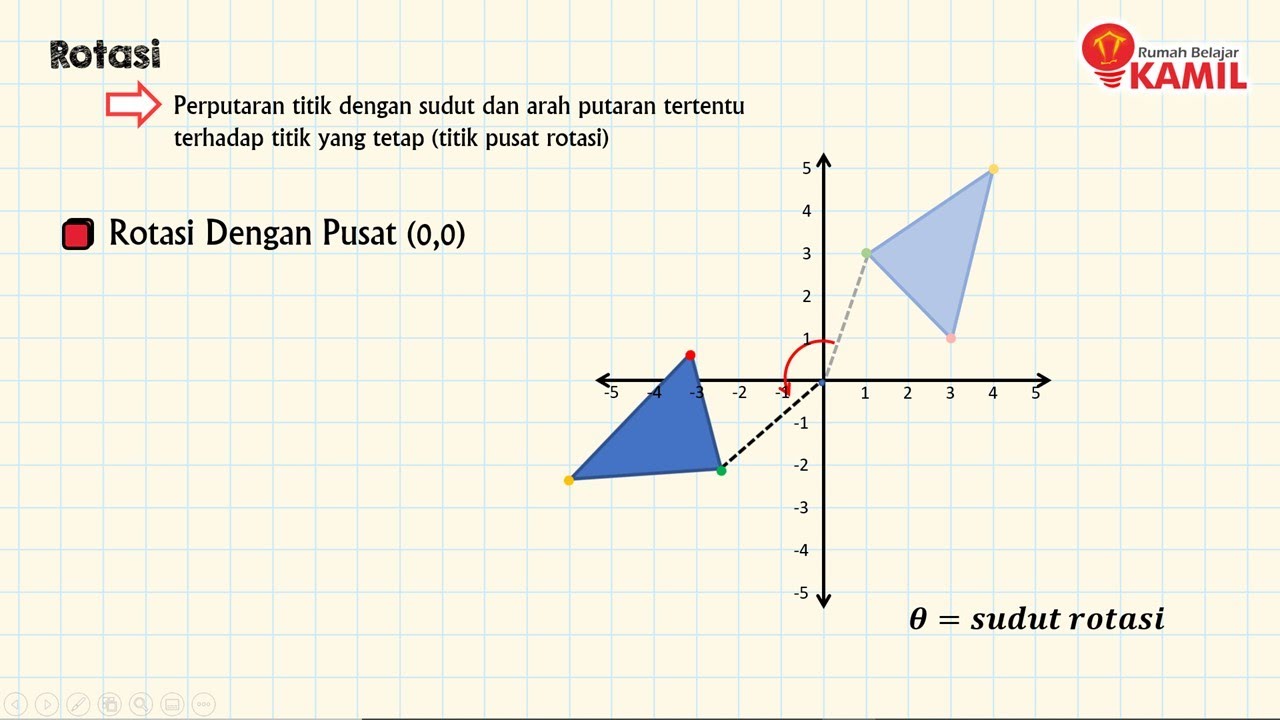
Matematika kelas 9 : Transformasi Geometri (part 3 : Rotasi I)

Transformasi Geometri [Part 2] - Translasi (Pergeseran)
5.0 / 5 (0 votes)