How Imaginary Numbers Were Invented
Summary
TLDRIn this video, the presenter explores the surprising importance of imaginary numbers in understanding the universe. Initially discovered as a solution to cubic equations, imaginary numbers became essential for describing reality in fields like physics and engineering. The video delves into the fascinating history and significance of these numbers, highlighting their unexpected connection to deeper truths about the natural world. The sponsor, Brilliant, offers an interactive platform for learning STEM concepts, including complex numbers and quantum mechanics, through hands-on experiences and visually engaging problems.
Takeaways
- 😀 Imaginary numbers were initially considered a quirky intermediate step but turned out to be fundamental to understanding the universe.
- 😀 The connection between mathematics and reality can be non-obvious, but abstract concepts like imaginary numbers have practical applications in physics and engineering.
- 😀 Even concepts considered purely theoretical, such as complex numbers, have real-world implications in fields like electrical engineering and quantum mechanics.
- 😀 Imaginary numbers are essential in solving cubic equations, which was one of their first uses, but their significance grew beyond that.
- 😀 The journey of discovering abstract mathematical ideas often involves letting go of the idea that math must directly mirror reality.
- 😀 Learning mathematics through interactive platforms, such as Brilliant, enhances understanding by allowing users to engage with the material directly.
- 😀 Brilliant's platform offers courses that cover topics from basic geometry to complex subjects like quantum mechanics and calculus.
- 😀 Interactive learning experiences, like manipulating mathematical concepts visually, can make difficult subjects more engaging and easier to understand.
- 😀 Complex topics such as calculus and imaginary numbers are taught step-by-step, with increasing difficulty and helpful hints provided when needed.
- 😀 For those interested in diving deeper into concepts like complex numbers, Brilliant offers specific courses on topics like Euler's formula and the Mandelbrot set.
Q & A
What is the significance of imaginary numbers in mathematics?
-Imaginary numbers, though initially considered odd or abstract, turned out to be fundamental in understanding the universe, particularly in areas like quantum mechanics and electrical engineering. Their discovery was an unexpected but crucial step in advancing mathematical and physical theories.
How did imaginary numbers arise in mathematics historically?
-Imaginary numbers arose when mathematicians were solving cubic equations. The process led to the introduction of numbers that were not 'real' in the traditional sense, as they involved square roots of negative numbers. This initially perplexed mathematicians but ultimately contributed to the broader understanding of complex numbers.
What role do imaginary numbers play in real-world physics?
-Imaginary numbers play a critical role in modern physics, particularly in the field of quantum mechanics. They help describe wave functions, resonance, and phenomena like quantum tunneling, which cannot be fully understood without using complex numbers.
Why were imaginary numbers initially considered controversial?
-Imaginary numbers were controversial because they represented numbers that did not have a direct, tangible connection to real-world quantities. They were seen as abstract and 'fictional,' which led to skepticism about their value in solving real-world problems.
What is Euler's formula, and how does it relate to imaginary numbers?
-Euler's formula, e^(ix) = cos(x) + i*sin(x), is a fundamental equation in mathematics that connects complex exponentials to trigonometric functions. It demonstrates how imaginary numbers are deeply connected to real numbers and can describe periodic phenomena such as wave motion.
How did mathematicians' views on imaginary numbers evolve over time?
-Mathematicians initially resisted the idea of imaginary numbers, but over time, they recognized their usefulness in solving complex problems, especially in physics and engineering. Their acceptance grew as their practical applications became evident, leading to the modern understanding of complex numbers.
What is the Mandelbrot set, and how does it relate to complex numbers?
-The Mandelbrot set is a set of complex numbers that produces a fractal when plotted. It demonstrates the beauty and complexity that can arise from the iteration of simple mathematical formulas, showcasing the profound and unexpected patterns that imaginary and complex numbers can generate.
How does Brilliant help in understanding complex topics like imaginary numbers?
-Brilliant uses interactive learning methods to teach complex topics, making them more accessible and engaging. The platform offers visual aids and practical exercises to help learners grasp abstract concepts like imaginary numbers, ensuring a deeper understanding of the material.
What is the connection between calculus and imaginary numbers?
-In calculus, imaginary numbers appear in areas like differential equations and integrals, particularly when dealing with complex waveforms, oscillations, or electromagnetic fields. They allow for a more complete and flexible mathematical framework for modeling real-world phenomena.
What is the 'Surface of Revolution' concept in calculus, and how does it relate to volume calculations?
-A Surface of Revolution is created by rotating a curve around an axis. In calculus, this concept is used to calculate the volume of 3D objects formed by rotating a 2D shape. It’s an example of how integrals are used in practical applications to solve real-world problems.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
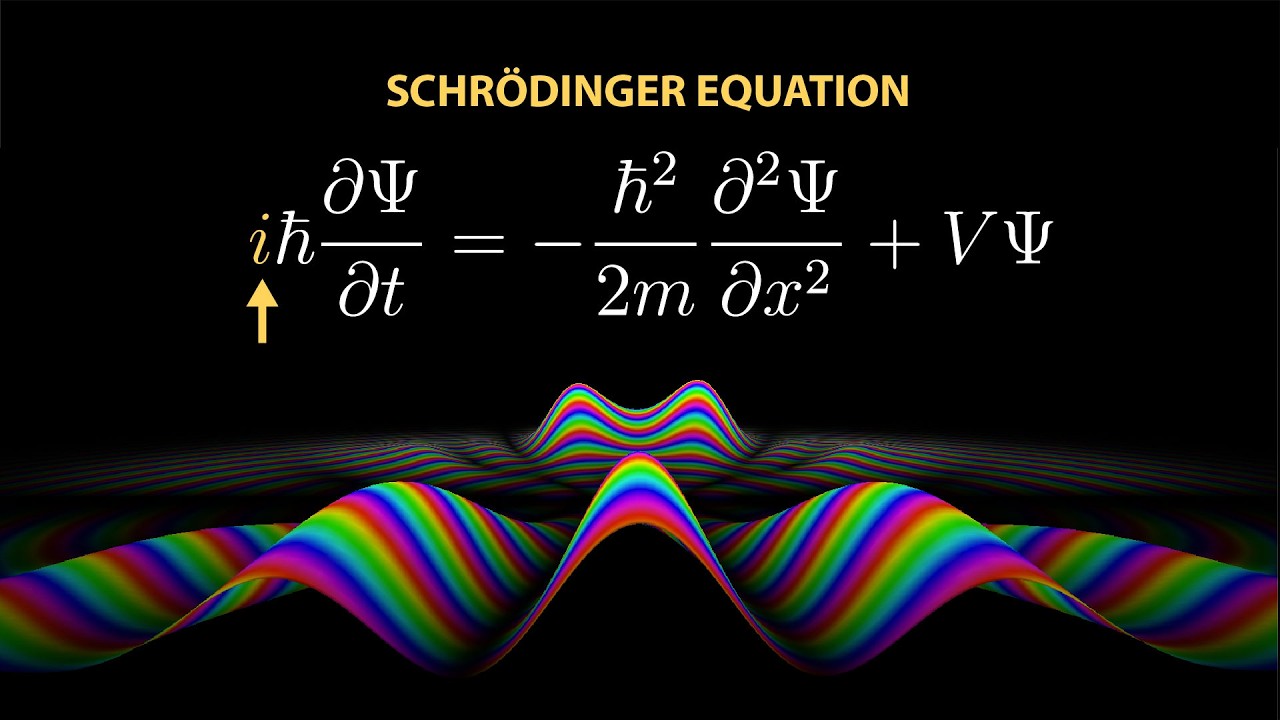
What is the i really doing in Schrödinger's equation?

Number System || Introduction (LESSON-1)
Números imaginarios | Introducción y potencias de "i"

FÁCIL e RÁPIDO | NÚMEROS COMPLEXOS

COMPLEXOS: OPERAÇÕES NA FORMA ALGÉBRICA (+, -, X) (AULA 4/14)

VLOG - Chapter 3: What Mathematics is About from Ian Stewart's Nature's Numbers
5.0 / 5 (0 votes)