[TAGALOG] Grade 10 Math Lesson: SOLVING GEOMETRIC SEQUENCE (Part III)- FINDING THE COMMON RATIO
Summary
TLDRIn this educational video, the host guides viewers through solving for the common ratio in geometric sequences. They use the formula \( a_n = a_1 \cdot r^{(n-1)} \) to find the ratio with examples: one with a first term of 7 and a fifth term of 112, and another with a first term of 5 and a fourth term of 320. The host demonstrates step-by-step calculations, including dividing terms and simplifying expressions to isolate the common ratio. A challenge problem is presented with a first term of 2 and a fourth term of 432, encouraging viewers to apply the learned method. The video concludes with an additional challenge and an invitation to join the next session.
Takeaways
- 📚 The video is a tutorial on finding the common ratio of a geometric sequence.
- 🎓 The formula used to find the common ratio is a_n = a_1 * r^(n-1) where a_n is the nth term, a_1 is the first term, and r is the common ratio.
- 🔢 The first example involves a sequence with the first term of 7 and the fifth term of 112.
- 🧮 To solve for the common ratio, the presenter divides the fifth term by the first term and then takes the fourth root of the result.
- 📈 The second example features a sequence with the first term of 5 and the fourth term of 320, leading to a common ratio of 4.
- 📉 The presenter emphasizes the importance of correctly substituting the values into the formula and solving for the common ratio.
- 🕵️♂️ The video includes a challenge problem for viewers to solve on their own, involving a sequence with the first term of 2 and the fourth term of 432.
- 🏁 The final common ratio for the challenge problem is found to be 6, using the same method as the examples.
- 🎉 The video concludes with an additional challenge question involving a sequence with the first term of 1 and a common ratio of 3, where the term in question is 243.
- 📢 The presenter encourages viewers to share the tutorial with friends, subscribe, and turn on notifications for more educational content.
Q & A
What is the formula for finding the nth term of a geometric sequence?
-The formula for the nth term of a geometric sequence is aₙ = a₁ × rⁿ⁻¹, where aₙ is the nth term, a₁ is the first term, r is the common ratio, and n is the term number.
How can you calculate the common ratio of a geometric sequence given the first and fifth terms?
-To find the common ratio, use the formula aₙ = a₁ × rⁿ⁻¹. Substitute the first term and fifth term into the equation, solve for r raised to the fourth power, and then take the fourth root to find r.
What is the common ratio if the first term of a geometric sequence is 7 and the fifth term is 112?
-The common ratio is 2. This is calculated by dividing the fifth term (112) by the first term (7) and then taking the fourth root of the result.
How do you solve for the common ratio when given the first and fourth terms of a geometric sequence?
-First, use the formula aₙ = a₁ × rⁿ⁻¹. Substitute the first and fourth terms, solve for r cubed, and then take the cube root of the result to find r.
What is the common ratio if the first term of a geometric sequence is 5 and the fourth term is 320?
-The common ratio is 4. This is calculated by dividing the fourth term (320) by the first term (5) and then taking the cube root of the result.
What is the process for solving a geometric sequence problem?
-The process involves writing down the geometric sequence formula, identifying the given terms, substituting them into the formula, solving for the unknowns, and simplifying the expression to find the common ratio or another term.
What is the common ratio if the first term of a geometric sequence is 2 and the fourth term is 432?
-The common ratio is 6. This is calculated by dividing the fourth term (432) by the first term (2), then taking the cube root of 216.
What is the next challenge question provided at the end of the video?
-The challenge question asks: 'The first term of a geometric sequence is 1, and its common ratio is 3. Which term is 243?'
What mathematical property is used when dividing both sides of an equation to isolate the common ratio?
-The multiplication property of equality is used when dividing both sides of an equation to isolate the common ratio, ensuring the equation remains balanced.
What is the purpose of the video lesson?
-The purpose of the video is to teach how to solve geometric sequence problems by finding the common ratio and using the geometric sequence formula effectively.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
How to Find the Geometric Means? Geometric Sequence - Grade 10 Math

GCSE Maths - Types of Number Sequences - Arithmetic vs Geometric

Grade 10 Math Q1 Ep6: Geometric Sequence VS Arithmetic Sequence

Sequences and Series (Arithmetic & Geometric) Quick Review
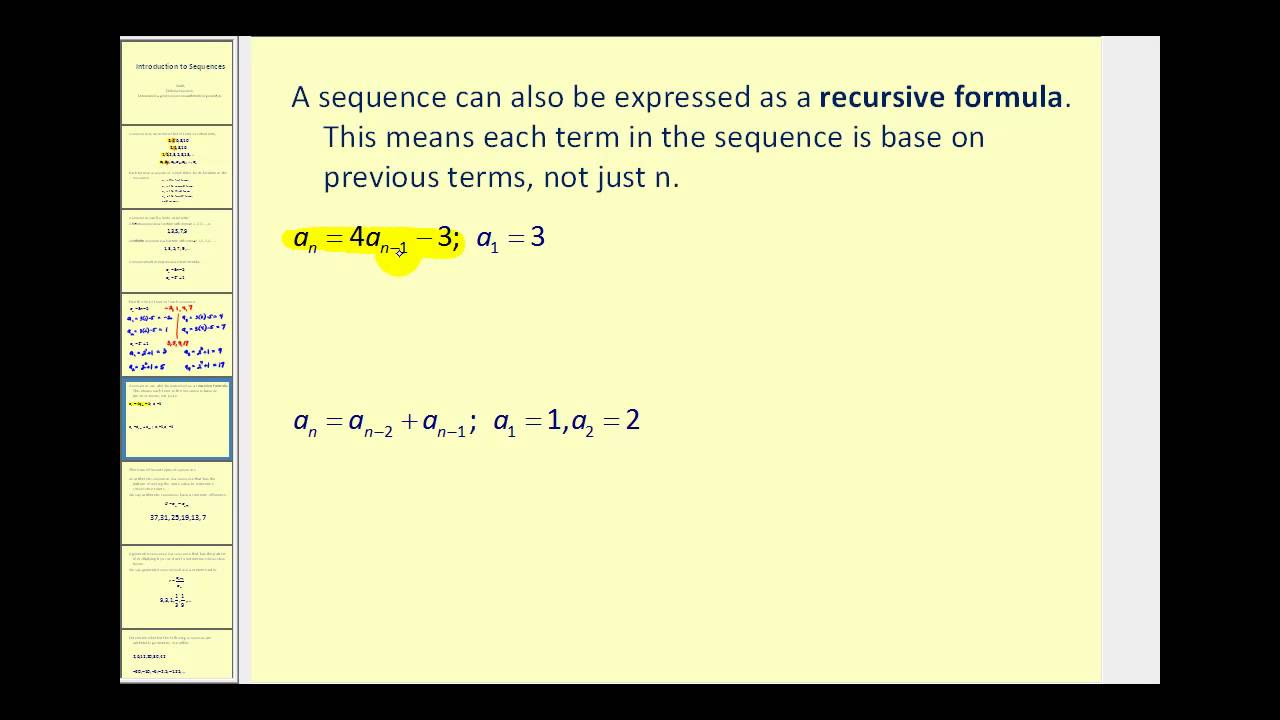
Introduction to Sequences
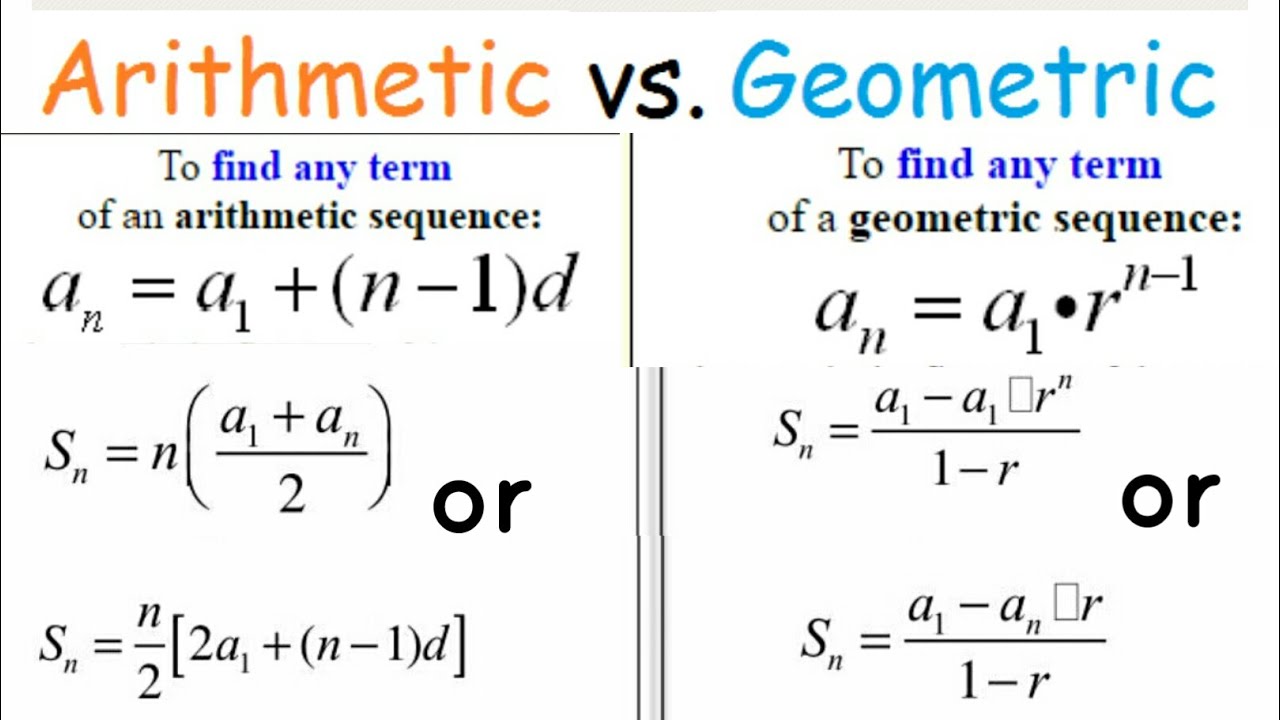
Arithmetic Sequence vs Geometric Sequence
5.0 / 5 (0 votes)