14 September BE 2567

Pawarisa [mint] Tanpoonkiat

14 Sept 202407:43

Summary

TLDRThe transcript discusses a control system lecture focusing on the Pi (Proportional-Integral) controller. It explains the need to determine the range of parameters for system stability using the R-array and R stability criteria. The lecture introduces disturbances and asks students to derive transfer functions, design proportional gains to minimize the effect of disturbances, and analyze system responses. It also touches on the physical concept of balancing, comparing the effectiveness of looking at the top versus the hand when balancing an object like an umbrella, and concludes with a bonus challenge related to a balancing pendulum.

Takeaways

🔍 The script discusses the concept of error in control systems and how it should ideally be zero.
📚 There's a mention of a PD (Proportional-Derivative) controller problem where the challenge is to compose the R array and find the parameter range for stability.
🧮 The Pi (Proportional-Integral) Controller is explained, emphasizing the need to satisfy R criteria for stability.
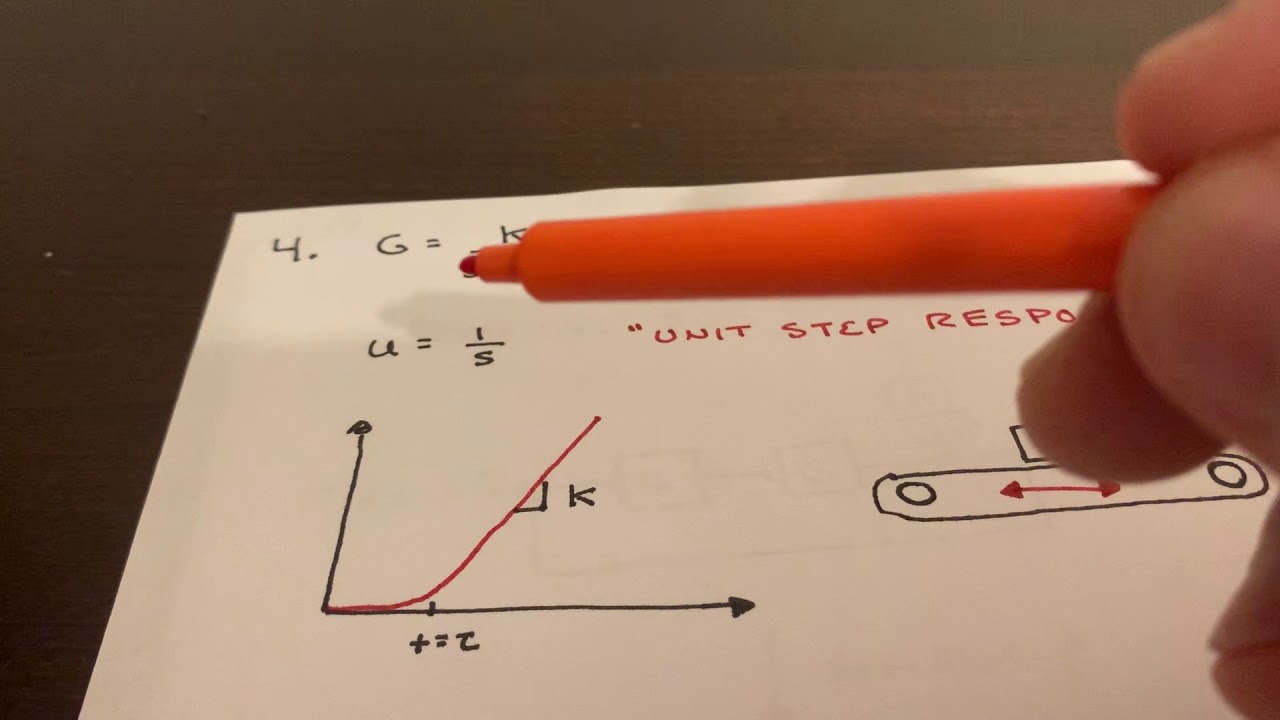
📉 The script simplifies the Pi Controller formula to an exam context, discussing the need to find conditions for system stability.
📘 Problem 5 introduces the concept of disturbance and asks to derive the transfer function of the output with respect to the disturbance.
🎓 The design of a proportional gain (KP) is discussed, with an emphasis on making the system's response to disturbance less than 10%.
📝 The script talks about the final value theorem and how it can be used to determine the steady-state response of a system.
📊 Question 6 involves obtaining a transfer function and analyzing its response in terms of peak time, setting time, and maximum overshoot.
🤔 The script ponders whether to keep certain information secret, suggesting a decision based on who should know the information.
🏋️‍♂️ A balancing challenge is introduced, comparing the strategy of looking at the top versus the hand when balancing a pendulum or an umbrella.

Q & A

What does the term 'PD controller' refer to in the context of the transcript?
-In the transcript, 'PD controller' likely refers to a Proportional-Derivative controller, which is a type of control system that uses the proportional and derivative terms to adjust the control input based on the error signal.
What is the significance of composing the 'R array' in the context of a PI controller?
-Composing the 'R array' in the context of a PI controller is significant for determining the range of the proportional gain (KP) that ensures the system remains stable according to the R stability criteria.
What is the general form of a PI controller as mentioned in the transcript?
-The general form of a PI controller mentioned in the transcript is a combination of a proportional term (KP * Error) and an integral term (KI * integral of Error), where KP and KI are constants.
What is the purpose of using the R stability criteria in the context of the transcript?
-The purpose of using the R stability criteria is to find the conditions, specifically the range of the proportional gain (KP), that keep the system stable when using a PI controller.
How does the introduction of disturbance affect the system as discussed in the transcript?
-The introduction of disturbance in the system, as discussed in the transcript, requires the derivation of the transfer function of the output with respect to the disturbance and the design of a proportional gain (KP) to minimize the effect of the disturbance on the system.
What is the ideal response of the system to disturbance as per the transcript?
-The ideal response of the system to disturbance, as per the transcript, is to have the output (x) divided by the disturbance approach zero, indicating minimal impact of the disturbance on the system's output.
What is the role of the proportional gain (KP) in minimizing the effect of disturbance on the system?
-The role of the proportional gain (KP) is to be designed in such a way that the ratio of the system's output (x) to the disturbance is less than a certain percentage (e.g., 10%), thus minimizing the effect of the disturbance.
What does 'x div by disturbance' refer to in the context of the transcript?
-In the context of the transcript, 'x div by disturbance' refers to the ratio of the system's output (x) to the disturbance, which should be minimized to ensure the system's stability and performance.
What is the significance of the final value theorem mentioned in the transcript?
-The final value theorem mentioned in the transcript is used to determine the steady-state value of the system's output (x) when the input is a step function, which is crucial for analyzing the system's long-term behavior.
What is the challenge presented in the transcript regarding balancing a pendulum?
-The challenge presented in the transcript is to balance a pendulum by looking at the top instead of the hand, which is suggested to be a more successful strategy due to the physical dynamics involved in balancing.

Outlines

00:00

🔍 Understanding Pi Controller Stability

The paragraph discusses the concept of a Pi controller in control systems. It mentions that if the input is zero, the error will also be zero. The speaker acknowledges a mistake in previously stating that the problem for the controller is a PD controller, when it is actually a Pi controller. The Pi controller is composed of a proportional term (KP) and an integral term (Ki), where KP is constant and proportional to the error, and Ki is a constant multiplied by the integral of the error. The goal is to find the range of the parameter 'a' that satisfies the stability criteria of the R array using the R stability criteria, as outlined in problem 5. The paragraph also introduces the concept of disturbance and asks the listener to derive the transfer function of the output with respect to the disturbance. The task is to design a proportional gain (KP) such that the steady-state error (x/ST) is less than 10% due to the disturbance. The speaker emphasizes the importance of understanding the loop transfer function and the steady-state response to a unit step force.

05:01

📚 Final Value Theorem and Transfer Function Analysis

This paragraph talks about using the final value theorem to determine the steady-state value of 'x'. It mentions that the transfer function has already been provided and asks for the calculation of the rise time, peak time, and maximum overshoot, as well as sketching the response. The speaker also poses a question about the physical meaning of balancing a pendulum, suggesting that looking at the top of the pendulum rather than the hand can be more effective. The paragraph ends with a teaser about a challenge involving balancing an umbrella, asking whether looking at the hand or the top is more successful and why. The speaker hints at a bonus point for those who attended the previous class but does not reveal what the bonus is.

Mindmap

Keywords

💡Error

In the context of the video, 'Error' refers to the difference between the desired output and the actual output in a control system. It is a fundamental concept in control theory, where minimizing error is a primary goal. The script mentions that the error should ideally be zero, indicating a perfect match between the desired and actual outcomes. This is crucial for the stability and performance of the system being controlled.

💡PD Controller

A 'PD Controller' is a type of controller in control systems that uses both proportional and derivative actions to adjust the control input based on the error. The 'P' stands for proportional, which is a response that is directly proportional to the current error, and 'D' stands for derivative, which is a response that is proportional to the rate of change of the error. The script discusses the need to compose the 'R' array and find the range of parameters to satisfy stability criteria, which is directly related to the tuning of a PD Controller.

💡R Array

The 'R Array' mentioned in the script is likely a matrix or array used in the design of control systems, particularly in the context of the 'R' stability criteria. It is used to determine the stability of the system by analyzing the range of parameters. The script suggests that students need to find the conditions that make the system stable, which involves understanding and manipulating the 'R' array.

💡Stability Criteria

Stability criteria are rules or conditions that a system must meet to be considered stable. In control systems, this often involves ensuring that the system does not oscillate uncontrollably or diverge from the set point. The script refers to using 'R' stability criteria to determine the range of a parameter that keeps the system stable, which is a critical part of designing a controller.

💡Proportional Gain (KP)

Proportional gain, often denoted as 'KP', is a key parameter in proportional control systems. It determines the extent to which the control system output is adjusted in response to the error. A higher KP value results in a more aggressive response to error, but it can also lead to instability if set too high. The script discusses designing the KP so that the system's response to disturbance is minimized, which is a common objective in control system design.

💡Disturbance

In the context of control systems, 'Disturbance' refers to any unwanted input or change that affects the system's output. The script introduces the concept of disturbance and asks to derive the transfer function of the output with respect to the disturbance. This is important for understanding how well the system can reject or accommodate external influences and maintain its desired performance.

💡Transfer Function

A 'Transfer Function' is a mathematical representation that describes the relationship between the input and output of a system. It is particularly used in control systems to analyze the system's dynamic behavior. The script asks to derive the transfer function with respect to the disturbance, which would help in understanding how changes in the disturbance affect the system's output.

💡Time Peak

The 'Time Peak' refers to the time it takes for a system's output to reach its peak value in response to a step input. It is an important parameter in evaluating the speed of response of a control system. The script mentions obtaining the transfer function and calculating the time peak, which is crucial for assessing the system's performance and making design improvements.

💡Maximum Overshoot

Maximum overshoot is the measure of how much the output of a system exceeds the desired value before settling down. It is a critical parameter in control system design as it affects the stability and accuracy of the system. The script asks to sketch the response and calculate the maximum overshoot, indicating its importance in evaluating the system's performance.

💡Balancing Pendulum

A 'Balancing Pendulum' is a classic example used in control systems to demonstrate the principles of feedback and control. The script asks a question about why it is preferred to look at the top of a pendulum rather than the hand when trying to balance it. This is likely to illustrate the concept of feedback and the importance of focusing on the system's output rather than the input.

Highlights

Introduction of the concept of 'Error' and its significance in control systems.

Explanation of the ideal system where error is zero.

Mistake correction regarding the PD problem for the controller.

Discussion on composing the R array and finding the range of parameters in PI control.

Definition and function of a PI Controller with constant KP and KI.

Simplification of PI controller form to exam form.

Use of R stability criteria to determine system stability.

Problem 5 introduction involving disturbance and distance.

Derivation of the transfer function of output with respect to the disturbance.

Design of proportional gain KP to minimize the effect of disturbance.

Ideal system preference for zero disturbance.

Practical approach to achieving less than 10% disturbance ratio.

Explanation of the final value theorem in control systems.

Introduction to question number 6 involving transfer function and system response.

Discussion on obtaining the right time peak, time setting, and maximum overshoot.

Sketching the response of a transfer function.

Bonus question on balancing a pendulum and its physical implications.

Challenge to balance an umbrella and its relevance to control systems.

Conclusion and summary of the second half of the challenge.