How to Find the LCM using Prime Factorization | Least Common Multiple | Math with Mr. J
Summary
TLDRIn the video 'Math with Mr J,' the presenter teaches viewers how to find the Least Common Multiple (LCM) using prime factorization, an efficient method for larger numbers. The tutorial begins with the prime factorization of 15 and 27, illustrating the process of breaking down numbers into their prime components. It then matches these factors vertically and multiplies them to find the LCM, which for 15 and 27 is 135. The video continues with another example using 28 and 52, demonstrating the same technique to determine their LCM as 364. This approach simplifies the LCM calculation, making it more manageable for complex numbers.
Takeaways
- 📚 The video teaches how to find the Least Common Multiple (LCM) using prime factorization.
- 🔢 Prime factorization is a strategy suitable for larger numbers that simplifies the LCM calculation.
- 🌰 The example of finding LCM for 15 and 27 is used to demonstrate the process.
- 📝 Prime factorization of 15 is 3 and 5, both of which are prime numbers.
- 📝 Prime factorization of 27 is 3 x 3 x 3, since 9 (3 x 3) is a factor of 27 and 3 is prime.
- 📋 The script instructs to list and match prime factors of the numbers vertically.
- 🔄 For 15 and 27, the matched prime factors are 3 (from 15) and 3 x 3 (from 27), and the unmatched factor is 5 (from 15).
- 🧮 The LCM is calculated by multiplying the highest powers of all prime factors involved, resulting in 135 for 15 and 27.
- 🌰 Another example is given with numbers 28 and 52, showing the step-by-step prime factorization.
- 📝 The prime factorization of 28 is 2 x 2 x 7, and for 52, it's 2 x 2 x 13.
- 📋 The unmatched prime factors for 28 and 52 are 7 and 13, respectively, used to calculate the LCM.
- 🧮 The LCM for 28 and 52 is found by multiplying 2 x 2 x 7 x 13, which equals 364.
Q & A
What is the primary focus of the video by Mr. J?
-The primary focus of the video is to teach how to find the least common multiple (LCM) using prime factorization, a method that is particularly useful for larger numbers.
Why is prime factorization a better approach for finding LCM compared to listing multiples?
-Prime factorization is a better approach for finding the LCM of larger numbers because listing out multiples can be difficult and time-consuming, whereas prime factorization provides a more systematic and efficient method.
What are the first two numbers Mr. J uses to demonstrate the prime factorization method for finding LCM?
-The first two numbers Mr. J uses to demonstrate the method are 15 and 27.
What is the prime factorization of 15 as presented in the video?
-The prime factorization of 15 is 3 and 5, as both numbers are prime and cannot be broken down further.
How is the prime factorization of 27 derived in the video?
-The prime factorization of 27 is derived by first recognizing that 3 and 9 are factors of 27. Then, since 9 can be broken down further into 3 times 3, the prime factorization is 3 times 3 times 3.
What is the least common multiple of 15 and 27 according to the video?
-The least common multiple of 15 and 27, found using prime factorization, is 135.
What is the next pair of numbers Mr. J uses to illustrate the process after 15 and 27?
-After demonstrating with 15 and 27, Mr. J uses the numbers 28 and 52 to further illustrate the process of finding LCM using prime factorization.
What is the prime factorization of 28 as explained in the video?
-The prime factorization of 28 is 2 times 2 times 7, as 14 (which is 2 times 7) is a factor of 28, and both 2 and 7 are prime numbers.
Can you explain the prime factorization of 52 as presented in the video?
-The prime factorization of 52 is 2 times 2 times 13, as 26 (which is 2 times 13) is a factor of 52, and both 2 and 13 are prime numbers.
What is the least common multiple of 28 and 52 as calculated in the video?
-The least common multiple of 28 and 52, calculated using prime factorization, is 364.
How does Mr. J suggest multiplying the prime factors to find the LCM in the video?
-Mr. J suggests multiplying the highest powers of all prime factors present in the factorization of the given numbers to find the LCM.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
How to Find the LCM (2 Different Ways) | Least Common Multiple | Math with Mr. J

MMC - MÍNIMO MÚLTIPLO COMUM
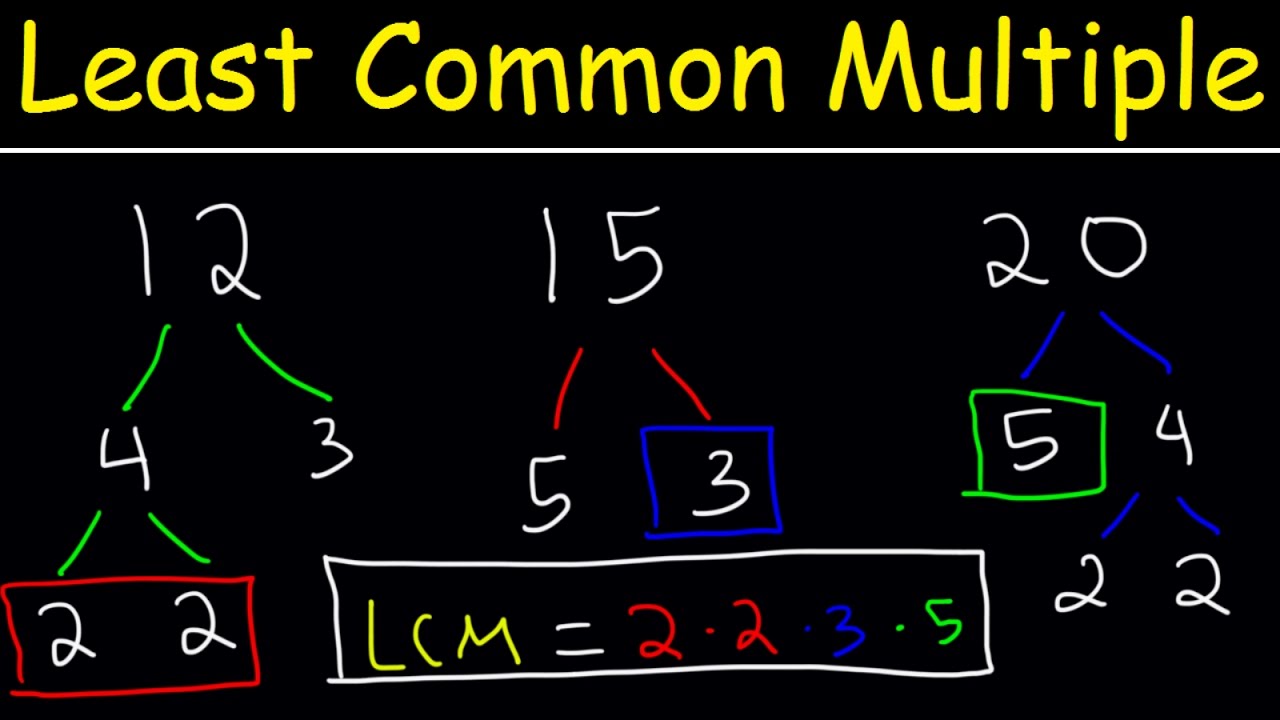
How To Find The LCM of 3 Numbers - Plenty of Examples!

SOAL CERITA KPK DAN FPB KELAS 5

Discrete Math - 4.3.2 Greatest Common Divisors and Least Common Multiples
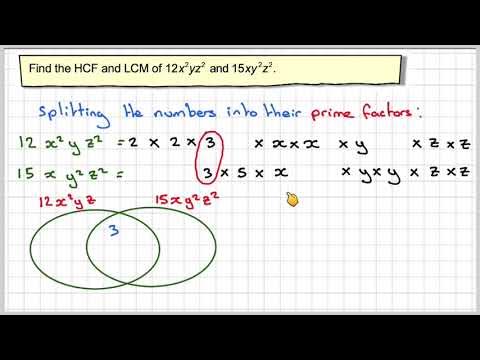
Finding the HCF and LCM of algebraic expressions
5.0 / 5 (0 votes)