#215 Problem on Nyquist plot in control systems || EC Academy

Summary

This transcript is a technical lecture on control systems, focusing on the transfer function and stability analysis. It covers critical points, range calculations, and various system configurations, explaining complex concepts with examples. The speaker dives into mathematical equations, stability conditions, and the use of software for plotting and analyzing system behavior. The lecture emphasizes the importance of system stability and includes real-world applications, such as determining critical points and adjusting parameters for optimal system performance. The use of graphical representation and step-by-step calculations is central to understanding the topics discussed.

Takeaways

• 😀 The script introduces a lecture on control systems and their transfer functions.
• 😀 The importance of determining the range of 'K' for which the system remains stable is emphasized.
• 😀 It is difficult to determine the number of poles in a system based solely on the given information.
• 😀 A critical point in the system should not be inserted, especially when working with complex systems like airplanes.
• 😀 There is a mention of a semi-circle representing a system with infinite range, where values approach infinity.
• 😀 The script highlights the use of plots to understand system stability and critical points.
• 😀 Various mathematical terms and calculations, such as omega and the square of values, are discussed in the context of system analysis.
• 😀 The importance of subscribing to the channel for further updates is frequently mentioned, blending technical content with engagement calls.
• 😀 The script briefly covers concepts like clock-wise and anti-clockwise rotation in the context of system modeling.
• 😀 There is an emphasis on using software tools to analyze and visualize the stability of systems in control theory.

Q & A

What is the main topic of the transcript?

The main topic of the transcript is related to control systems, particularly the transfer function, stability analysis, and calculating certain parameters such as range and poles in a control system.

What is the significance of the 'range of K' in control systems?

The 'range of K' refers to the range of values for the system's gain parameter, K, that ensures the system remains stable. It helps determine the stability criteria and the performance of the control system.

What is meant by a 'critical point' in the context of control systems?

A 'critical point' in control systems refers to a point where the system's behavior changes drastically, often leading to instability. These points are used to assess the stability and performance of the system.

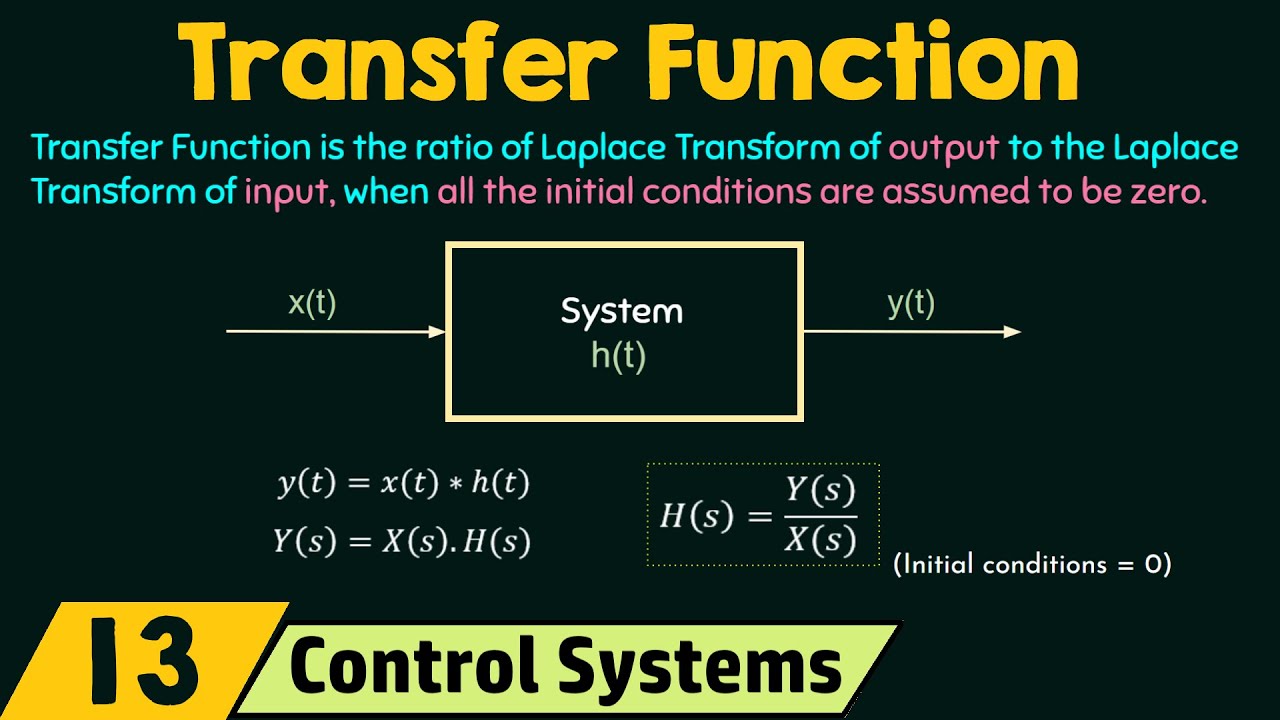
What role does the transfer function play in control systems?

The transfer function in a control system describes the relationship between the system's input and output in the frequency domain. It is crucial for analyzing system behavior, stability, and designing controllers.

What does the term 'stability' refer to in control systems?

Stability in control systems refers to the ability of a system to return to equilibrium after a disturbance. A stable system will not exhibit uncontrollable oscillations or diverging behavior over time.

Why is calculating poles important in control systems?

Calculating poles is important because the location of the poles on the complex plane determines the system's stability and transient response. If poles are in the right half-plane, the system is unstable.

What is the significance of a 'feedback system' in control theory?

A feedback system adjusts its output based on the input and the error signal. Feedback is used to control system behavior, improve accuracy, and maintain stability by reducing errors over time.

What does 'zero divided by K' imply in the system's equations?

In the context of the system's equations, 'zero divided by K' suggests a point where the output becomes zero, potentially indicating a boundary condition or a transition point for the system's behavior.

What is the importance of the term 'omega squared' in the transfer function?

Omega squared represents the frequency response of the system and plays a crucial role in determining the system's resonance and damping characteristics. It influences how the system responds to different frequencies.

How does the concept of 'rotation' relate to the system's stability?

Rotation in the context of control systems could refer to the system's phase shift or frequency response. Understanding the system's rotation helps in determining its stability, particularly in feedback systems with phase shifts.