Simple Harmonic Motion(SHM) - Position Equation Derivation
Summary
TLDRIn this lesson, Mr. P. explains the connection between circular motion and simple harmonic motion (SHM), focusing on deriving the position equation for an object in SHM. Using a motion-tracked dot, he shows how the position of an object can be described by a cosine function, with amplitude and frequency as key variables. The discussion also covers angular velocity, angular frequency, and the relationship between sine and cosine functions in SHM. Key concepts like phase shift, radians, and the importance of using radian mode on calculators are also highlighted. The lesson emphasizes understanding the derivation of equations for a deeper grasp of SHM.
Takeaways
- 😀 The motion of an object in circular motion can be related to simple harmonic motion (SHM) by analyzing its x-position.
- 😀 The x-position of an object in SHM can be described by the equation x = r * cos(θ), where r is the radius and θ is the angular displacement.
- 😀 To express the x-position as a function of time, we introduce angular velocity (ω), where θ = ω * t, which allows us to replace θ in the equation for x.
- 😀 Angular velocity (ω) is related to frequency (f) by ω = 2π * f, which is essential to convert circular motion into SHM terms.
- 😀 The position of an object in SHM can be written as x(t) = A * cos(2π * f * t), where A is the amplitude, f is the frequency, and t is time.
- 😀 The amplitude (A) represents the maximum displacement from the equilibrium position in SHM.
- 😀 The motion tracked dot on the yellow marker cap moves in SHM, and its path can be described by the equation for SHM: x(t) = A * cos(2π * f * t).
- 😀 A cosine graph of the object's motion shows the maximum displacements (amplitude) and the equilibrium position (zero displacement).
- 😀 The sine equation can also describe SHM, but it's used when the object starts at the equilibrium position, with a phase shift introduced if needed.
- 😀 Angular frequency (ω) and frequency (f) are related but distinct: ω = 2π * f, and they have different units (ω in radians per second, f in hertz).
Q & A
What is the relationship between circular motion and simple harmonic motion (SHM)?
-Circular motion, when viewed from the side, can be described as simple harmonic motion. Specifically, the horizontal projection of a point moving in a circle exhibits SHM.
What variables are involved in determining the position of the cap in SHM?
-The variables involved include the radius (r) of the circular motion, the position of the cap in the x direction (x), and the angular displacement (theta) from an initial position.
How is the x position of the yellow marker cap related to angular displacement?
-The x position of the cap is given by the equation x = r * cos(theta), where r is the radius of the circular motion and theta is the angular displacement.
How is time incorporated into the equation for the x position in SHM?
-To express the position as a function of time, the angular displacement (theta) is related to time through angular velocity (omega). The equation becomes x = r * cos(omega * t), where omega is the angular velocity and t is time.
What is angular velocity, and how is it related to frequency?
-Angular velocity (omega) is the rate of change of angular displacement and is given by omega = 2π * frequency. Frequency is the number of cycles per second, and angular velocity is in radians per second.
What does the equation x = r * cos(2π * frequency * t) describe?
-This equation describes the position of an object in simple harmonic motion as a function of time, where r is the amplitude, and frequency determines the speed of oscillation.
What role does amplitude play in simple harmonic motion?
-Amplitude (A) represents the maximum displacement of the object from the equilibrium position. It is the value of the x position when time equals zero, where cos(0) equals 1.
What is the difference between using sine and cosine in SHM equations?
-The sine and cosine functions both describe SHM but differ in the initial conditions. The cosine equation assumes the initial position is at the maximum displacement, while the sine equation assumes the object starts at the equilibrium position.
What is the significance of the phase constant (phi) in SHM?
-The phase constant (phi) is used to adjust the equation for SHM when the object starts at a position other than the maximum displacement or equilibrium. It represents a horizontal shift in the wave, modifying the initial condition of the motion.
Why is it important to use radians and not degrees when applying SHM equations?
-The equations for simple harmonic motion are derived using radians, and using degrees would lead to incorrect results. It is essential to ensure your calculator is set to radian mode when working with these equations.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео

English Expressions: three-word phrasal verbs

Penggunaan Simple Present Tense dan Contohnya | Kampung Inggris LC

ESL Writing - Summarizing and Paraphrasing

Mastering the Nominative Case in German: A Complete Guide! (Beginner / A1-A2) - 1080p/Full HD 🔥
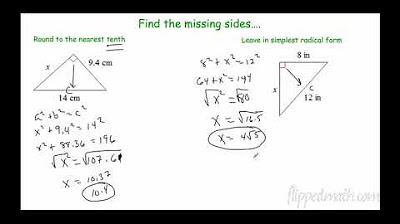
Geometry – 7.1 Pythagorean Theorem and Its Converse

Symmetrical Name Monsters with Mr. Snyder
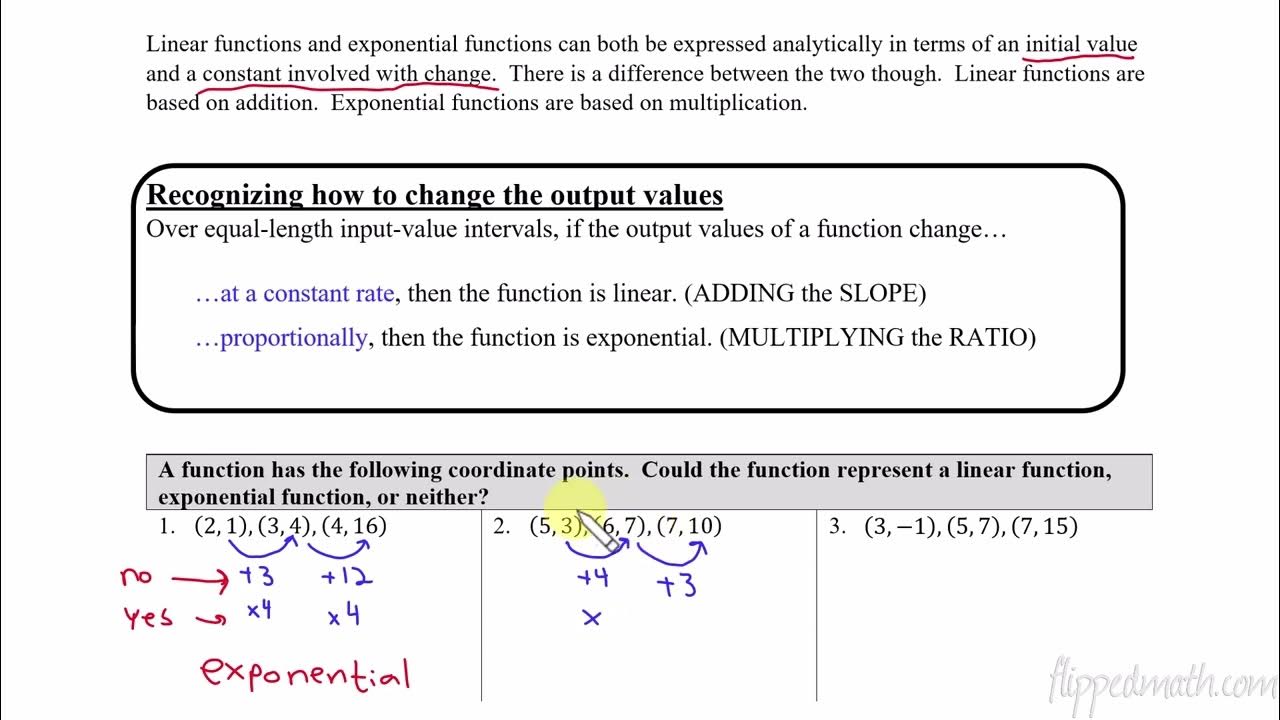
AP Precalculus – 2.2 Change in Linear and Exponential Functions
5.0 / 5 (0 votes)
