Understanding Failure Theories (Tresca, von Mises etc...)
Summary
TLDRThis video introduces the key concepts of material failure theories, exploring how we predict failure under different stress conditions. It explains the differences between ductile and brittle materials, focusing on how failure occurs through yielding or fracture. The video covers commonly used failure theories like Maximum Principal Stress, Tresca, and von Mises for ductile materials, and Coulomb-Mohr for brittle materials. It also emphasizes how hydrostatic stresses do not affect yielding in ductile materials but do influence failure in brittle materials. The content is backed by experimental data, showing how these theories are applied in real-world scenarios.
Takeaways
- 😀 Failure in materials occurs when stresses exceed a critical level, with ductile materials failing through plastic deformation and brittle materials through fracture.
- 😀 For uniaxial stress states, material failure is straightforward to predict based on yield or ultimate strength, but more complex stress states require advanced failure theories.
- 😀 Failure theories for ductile materials must recognize that hydrostatic stresses (volume-changing stresses) do not cause yielding, which is driven by deviatoric (shape-distorting) stresses.
- 😀 Maximum Principal Stress Theory (Rankine) is the simplest but not ideal for ductile materials, as it doesn't account for the independence of yielding from hydrostatic stresses.
- 😀 The Tresca failure theory (Maximum Shear Stress Theory) predicts yielding when the maximum shear stress reaches the material's yield strength, independent of hydrostatic stress.
- 😀 The von Mises failure theory (Maximum Distortion Energy Theory) predicts yielding based on the total distortion energy, providing a more accurate failure criterion for ductile materials.
- 😀 Mohr’s Circle helps visualize the stress state and differentiate between hydrostatic and deviatoric stresses in a tri-axial stress state.
- 😀 The Tresca and von Mises criteria for ductile materials generally provide similar results, but von Mises theory is more widely used due to its better agreement with experimental data.
- 😀 For brittle materials, failure is primarily due to fracture rather than yielding, and their failure theories need to account for differences in tensile and compressive strengths.
- 😀 Coulomb-Mohr theory is commonly used for brittle materials, incorporating both tensile and compressive strengths, and is sensitive to hydrostatic stresses.
- 😀 The Modified Mohr theory refines Coulomb-Mohr, better fitting experimental data and providing a more accurate failure prediction for brittle materials.
- 😀 Yield surfaces for failure theories in both ductile and brittle materials help visualize the conditions under which failure occurs, with different theories offering varying degrees of conservatism and accuracy.
Q & A
What is the primary focus of the video?
-The video focuses on explaining different failure theories for materials, particularly ductile and brittle materials, and how these theories help predict failure under various stress conditions.
How does static failure occur in materials?
-Static failure in materials occurs when the stresses within an object reach a critical level, causing the material to fail. For ductile materials, this is typically associated with the onset of plastic deformation, while for brittle materials, it results in fracture.
What is the role of failure theories in material science?
-Failure theories predict when a material will fail by comparing the stress state of an object with known material properties, such as yield or ultimate strength, obtained through uniaxial tests.
What is the difference between ductile and brittle materials in terms of failure?
-Ductile materials fail due to plastic deformation, which is typically linked to shear stresses, whereas brittle materials fail through fracture, which can occur under tensile or compressive stresses.
What is Maximum Principal Stress theory, and why is it not ideal for ductile materials?
-Maximum Principal Stress theory, or Rankine theory, predicts failure when the maximum or minimum principal stress reaches the material's yield or ultimate strength. However, it is not suitable for ductile materials because it doesn’t account for the fact that hydrostatic stresses do not cause yielding in these materials.
What are hydrostatic stresses and how do they affect ductile materials?
-Hydrostatic stresses are stresses that cause a change in volume, and they are the same in all directions (equal principal stresses). These stresses do not contribute to yielding in ductile materials because yielding is caused by shear deformation, not volumetric change.
What is the significance of deviatoric stresses in material failure?
-Deviatoric stresses are stresses that cause shape distortion without changing the volume. For ductile materials, yielding is caused by deviatoric stresses rather than hydrostatic stresses, which is why good failure theories for ductile materials focus on deviatoric components.
What are the Tresca and von Mises failure criteria, and how do they differ?
-The Tresca failure criterion (maximum shear stress theory) states that yielding occurs when the maximum shear stress equals the shear stress at yielding in a tensile test. The von Mises failure criterion (maximum distortion energy theory) states that yielding occurs when the distortion energy reaches that of yielding in a uniaxial tensile test. Von Mises theory is generally preferred as it aligns better with experimental data, although Tresca is more conservative.
How does Mohr's Circle help in understanding failure theories?
-Mohr's Circle is a graphical tool that helps visualize the stresses in a material at any given point. It allows for the identification of maximum shear stresses and the separation of hydrostatic and deviatoric stresses, aiding in the application of failure theories like Tresca and von Mises.
Why is von Mises theory preferred over Tresca in many engineering applications?
-Von Mises theory is preferred because it generally provides better alignment with experimental data and predicts failure more accurately. Although Tresca is simpler and more conservative, von Mises offers a more comprehensive understanding of material behavior under complex stress states.
What failure theory is typically used for brittle materials, and why?
-Coulomb-Mohr theory is commonly used for brittle materials because it accounts for the differences between tensile and compressive strengths, as well as the effects of hydrostatic stresses. This theory is more appropriate for materials that fail by fracture rather than yielding.
How does the Modified Mohr theory improve upon the Coulomb-Mohr theory?
-The Modified Mohr theory is a refinement of Coulomb-Mohr theory and provides better alignment with experimental data, particularly in scenarios where the Coulomb-Mohr theory does not accurately predict failure in certain quadrants of the stress state.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео

Sollecitazioni meccaniche (parte 1)
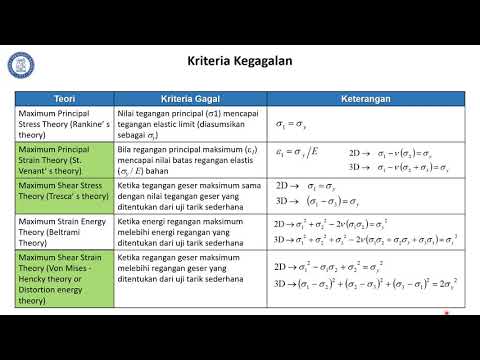
Analisis Kegagalan Logam: Modul 1 Segmen 2 (Kriteria Kegagalan)

Analisis Kegagalan Logam Modul 1 Segmen 3 (Kategori Kegagalan)

Mechanics of Materials: Lesson 16 - Fatigue and Creep Failures with S-N Diagram

Aprenda agora o que é tensão na resistência dos materiais (ResMat)

5 Criterio de resistencia Mohr Coulomb
5.0 / 5 (0 votes)
