AP Precalculus – 2.2 Change in Linear and Exponential Functions
Summary
TLDRIn this pre-calculus lesson, Mr. Bean explains the relationship between linear functions, exponential functions, and sequences. He compares arithmetic sequences with linear functions, showing how both involve an initial value and a constant rate of change, with linear functions using addition and exponential functions using multiplication. The lesson also delves into geometric sequences and exponential functions, highlighting the differences in domains and the behavior of each function. Through practical examples, Mr. Bean illustrates how to recognize and calculate linear and exponential functions, guiding students to differentiate between the two and apply them in various problems.
Takeaways
- 😀 Linear functions are related to arithmetic sequences, where the rate of change is constant and can be represented by the slope (m) in the slope-intercept form (y = mx + b).
- 😀 Exponential functions are related to geometric sequences, where the rate of change is proportional, represented by a constant ratio (r) in the form y = a * r^x.
- 😀 In both linear and exponential functions, the initial value plays a key role: the initial value of a linear function corresponds to the y-intercept, and for exponential functions, it's the starting value multiplied by the ratio.
- 😀 The domain of a sequence consists of discrete points, whereas the domain of a function is continuous, representing all real numbers.
- 😀 Arithmetic sequences follow a pattern of constant addition, while geometric sequences involve constant multiplication, which is the basis for exponential functions.
- 😀 To determine if a function is linear, check if the output values change by a constant amount (addition). If the output values change proportionally, the function is exponential (multiplication).
- 😀 In practice problems, identifying whether the change in output values is additive or multiplicative helps classify the function as linear or exponential.
- 😀 For linear functions, the equation can be derived from two points using the slope formula: m = (y2 - y1) / (x2 - x1), and then applying the point-slope form to find the equation.
- 😀 Exponential functions require finding the common ratio (r) by analyzing two points. Once r is determined, the equation can be written using the form y = a * r^x.
- 😀 Justification for classifying a function as linear or exponential should be based on identifying the pattern of change (constant addition for linear, proportional change for exponential).
- 😀 The ratio (r) in exponential functions is always positive because it represents the multiplicative change and never negative.
Q & A
What is the main topic of the lesson?
-The main topic of the lesson is understanding linear functions and exponential functions and comparing them with sequences, specifically arithmetic and geometric sequences.
How are linear functions similar to arithmetic sequences?
-Linear functions and arithmetic sequences are similar because both have an initial value and a constant rate of change. In linear functions, this is represented by the y-intercept and the slope, while in arithmetic sequences, it’s represented by the initial term and the common difference.
What is the key difference between the domains of sequences and functions?
-The domain of a sequence is discrete, consisting of individual points, while the domain of a function, such as a linear or exponential function, is continuous and includes all real numbers.
How do you identify if a function is linear or exponential?
-If the output values change at a constant rate for equal-length input intervals, the function is linear. If the output values change proportionally, where each change is by a consistent ratio, the function is exponential.
What is an example of a linear function equation using the given points?
-An example of a linear function equation using the points (3, 7) and (8, 1) would be f(x) = (-6/5)(x - 3) + 7, derived from the slope formula and point-slope form.
What is the process for finding the ratio in an exponential function?
-To find the ratio in an exponential function, you use the formula where the output at one point divided by the output at another point gives the ratio raised to the power of the difference in the x-values.
What is the domain of an exponential function?
-The domain of an exponential function is all real numbers, which means it extends from negative infinity to positive infinity.
Why is it important to justify whether a function is linear or exponential?
-Justifying whether a function is linear or exponential helps demonstrate an understanding of the underlying patterns in the data and ensures the correct application of mathematical principles.
What does it mean if the function changes proportionally?
-If a function changes proportionally, it means that for equal changes in the input, the output changes by a consistent ratio, characteristic of an exponential function.
What type of justification is required to prove a function is linear?
-To prove a function is linear, you must show that for each equal change in the input, the output has a constant rate of change, indicated by the same difference in output values.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео

Prereq 13: Complex Numbers

SHS Pre-calculus Q1 Ep1: Introduction to Pre-calculus and Conic Sections
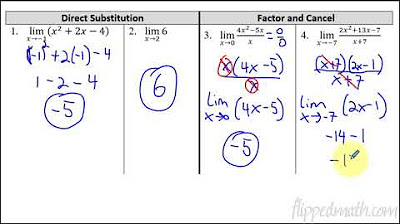
Calculus AB/BC – 1.6 Determining Limits Using Algebraic Manipulation
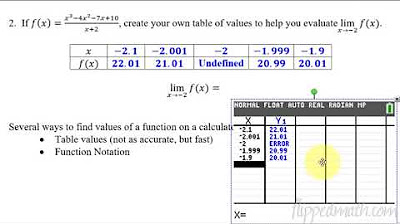
Calculus AB/BC – 1.4 Estimating Limit Values from Tables

Calculus AB/BC – 1.10 Exploring Types of Discontinuities

Calculus AB/BC – 2.1 Defining Average and Instantaneous Rate of Change at a Point
5.0 / 5 (0 votes)
