MENENTUKAN MODEL MATEMATIKA DARI SOAL CERITA SPtLDV
Summary
TLDRIn this educational video, the presenter explains how to create mathematical models from real-world problems involving linear inequalities with two variables. Using examples such as buying two types of oranges and transporting cars, the video guides viewers through the process of identifying variables, writing constraints, and formulating the system of inequalities. The goal is to teach viewers how to translate word problems into mathematical expressions, which can then be solved using methods like graphing or linear programming. The video emphasizes the importance of understanding constraints such as budget, weight, and capacity in these models.
Takeaways
- 😀 Mathematical models are representations of real-world problems using equations, inequalities, or functions.
- 😀 The first step in creating a mathematical model is identifying the variables that represent different aspects of the problem.
- 😀 Translating word problems into mathematical equations or inequalities is crucial in solving them effectively.
- 😀 In problems with two variables, such as buying oranges, variables can represent quantities like weight or cost.
- 😀 In the example of buying oranges, constraints like the budget and maximum weight are key to formulating inequalities.
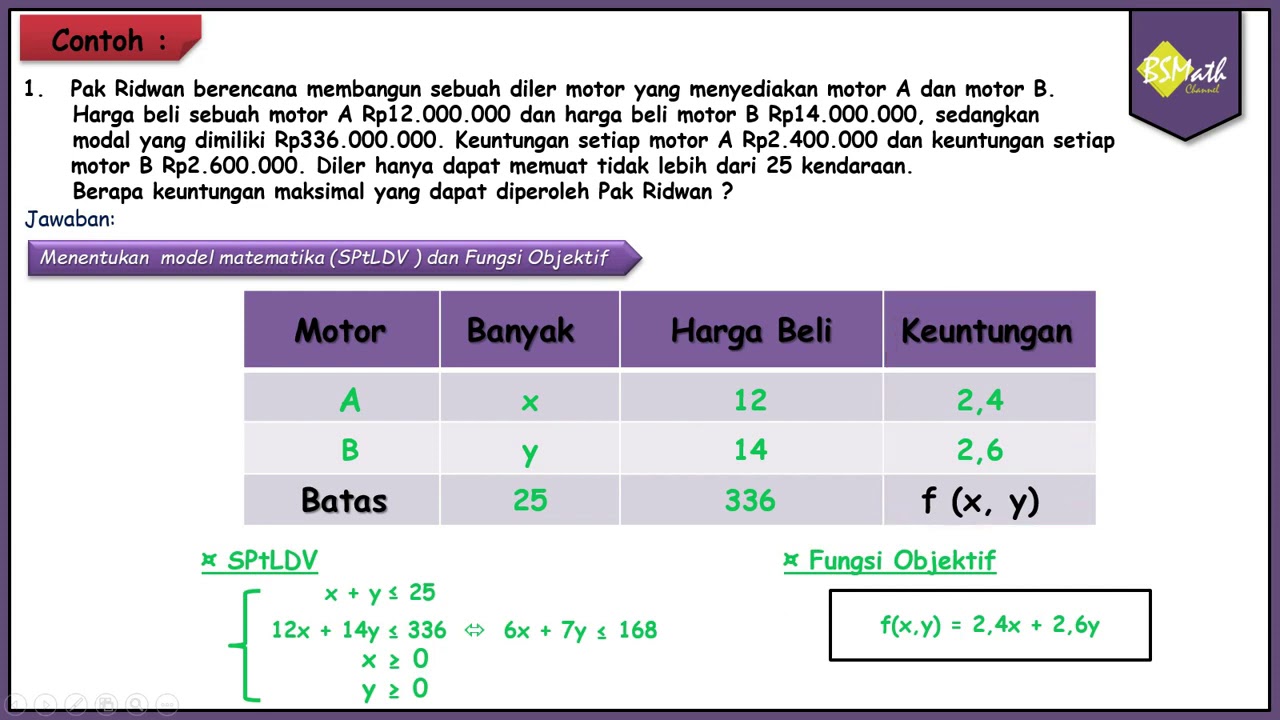
- 😀 An inequality such as 6000x + 4000y ≤ 50000 represents the total cost constraint in the orange-buying problem.
- 😀 The maximum weight constraint in the orange problem is represented by the inequality x + y ≤ 10.
- 😀 Non-negative constraints (x ≥ 0, y ≥ 0) are essential because quantities like weight or amount cannot be negative.
- 😀 The model for the vehicle transport problem involves using variables to represent the number of cars, with constraints on the total number of cars and the passenger capacity.
- 😀 In the vehicle transport example, the inequalities x + y ≤ 100 and 4x + 6y ≤ 500 represent the limitations on car numbers and passengers.
- 😀 After formulating the model, methods like graphing or optimization are used to find solutions and optimal values.
Q & A
What is the main topic of the video script?
-The main topic of the video script is about creating mathematical models for word problems involving systems of linear inequalities with two variables.
What does 'model matematika' (mathematical model) refer to in this context?
-In this context, 'model matematika' refers to a mathematical expression such as an equation, inequality, or function that represents a real-life problem or scenario in a mathematical form.
Why is it important to determine the variables when creating a mathematical model?
-It is important to determine the variables because they represent key quantities in the problem, and defining them properly helps in translating the problem into a solvable mathematical form.
What are the two variables used in the first example problem about purchasing oranges?
-In the first example about purchasing oranges, the two variables are the weight of orange type A (denoted as 'x') and the weight of orange type B (denoted as 'y').
What are the constraints in the first example regarding the budget and the basket capacity?
-The constraints in the first example are that the total expenditure for oranges must not exceed 50,000 IDR (given by the inequality 6000x + 4000y ≤ 50,000), and the total weight of the oranges must not exceed 10 kilograms (given by the inequality x + y ≤ 10).
Why must the variables x and y be non-negative in this problem?
-The variables x and y must be non-negative because they represent the quantity of oranges in kilograms, and negative quantities of oranges do not make sense in this context.
How is the first inequality in the example simplified?
-The first inequality 6000x + 4000y ≤ 50,000 is simplified by dividing through by 2000, resulting in the inequality 3x + 2y ≤ 25.
What does the second example in the video about transportation focus on?
-The second example focuses on a transportation problem where the goal is to determine how many vehicles of two types (sedan and SUV) should be used, subject to constraints on the total number of vehicles and the total number of passengers.
What are the constraints in the second example regarding the number of vehicles and passengers?
-The constraints in the second example are that the total number of vehicles (x + y) must not exceed 100 (x + y ≤ 100), and the total number of passengers (4x + 6y) must not exceed 500 (4x + 6y ≤ 500).
Why is it necessary to include the conditions 'x ≥ 0' and 'y ≥ 0' in both examples?
-It is necessary to include the conditions 'x ≥ 0' and 'y ≥ 0' because the variables represent quantities that cannot be negative, such as the weight of oranges or the number of vehicles.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео

Sistem Persamaan Linear Tiga Variabel (SPLTV) membuat model matematika | by Iga Apriliana Mahardika

Membuat Model Matematika Sistem Persamaan Linear Dua Variabel
Menyelesaikan Permasalahan Program Linear Menentukan Nilai Optimum dengan Metode Uji Titik Pojok

Soal & Pembahasan SPTLDV Sistem Pertidaksamaan Linear Dua Variabel SOAL CERITA

SISTEM PERSAMAAN LINEAR 3 VARIABEL / Mat kls 10

SPtDV • Part 8: Soal Menentukan Sistem Pertidaksamaan Linear Dua Variabel / SPtLDV dari Grafik (2)
5.0 / 5 (0 votes)
