CHORDS, ARCS AND ANGLES || GRADE 10 MATHEMATICS Q2
Summary
TLDRThis video lesson explores the relationship between chords, arcs, and angles in circles. It defines key concepts such as chords, which connect two points on a circle, and arcs, which are segments of the circle defined by those points. The lesson highlights the central angle, which is formed at the circle's center and is equal to its intercepted arc, and the inscribed angle, which is measured as half of its intercepted arc. Through examples and calculations, the video clarifies these geometric principles, making them accessible for learners seeking to understand circular geometry.
Takeaways
- 😀 A circle is defined as a set of all points in a plane that are a given distance (radius) from a fixed point (center).
- 😀 A chord is a line segment joining two points on the circumference of a circle.
- 😀 An arc smaller than a semi-circle is called a minor arc, while the larger arc is known as a major arc.
- 😀 The measure of a major arc is found by subtracting the measure of the corresponding minor arc from 360 degrees.
- 😀 A central angle's measure is equal to the measure of its intercepted arc.
- 😀 An inscribed angle has its vertex on the circle and its sides containing the endpoints of a chord; its measure is half that of the intercepted arc.
- 😀 When given the measure of a central angle, you can find the corresponding arc's measure directly.
- 😀 The measure of a semicircle is always 180 degrees.
- 😀 To find the measure of an inscribed angle, you can use the relationship with the intercepted arc.
- 😀 The relationship between central angles, inscribed angles, and intercepted arcs is fundamental in circle geometry.
Q & A
What is a circle?
-A circle is defined as a set of all points in a plane that are a given distance 'r' from a fixed point called the center.
What is a chord in a circle?
-A chord is a line segment that joins two points on the circumference of the circle.
What distinguishes a minor arc from a major arc?
-A minor arc is smaller than a semicircle, while a major arc is larger. The major arc's measure can be calculated as the difference between 360 degrees and the minor arc's measure.
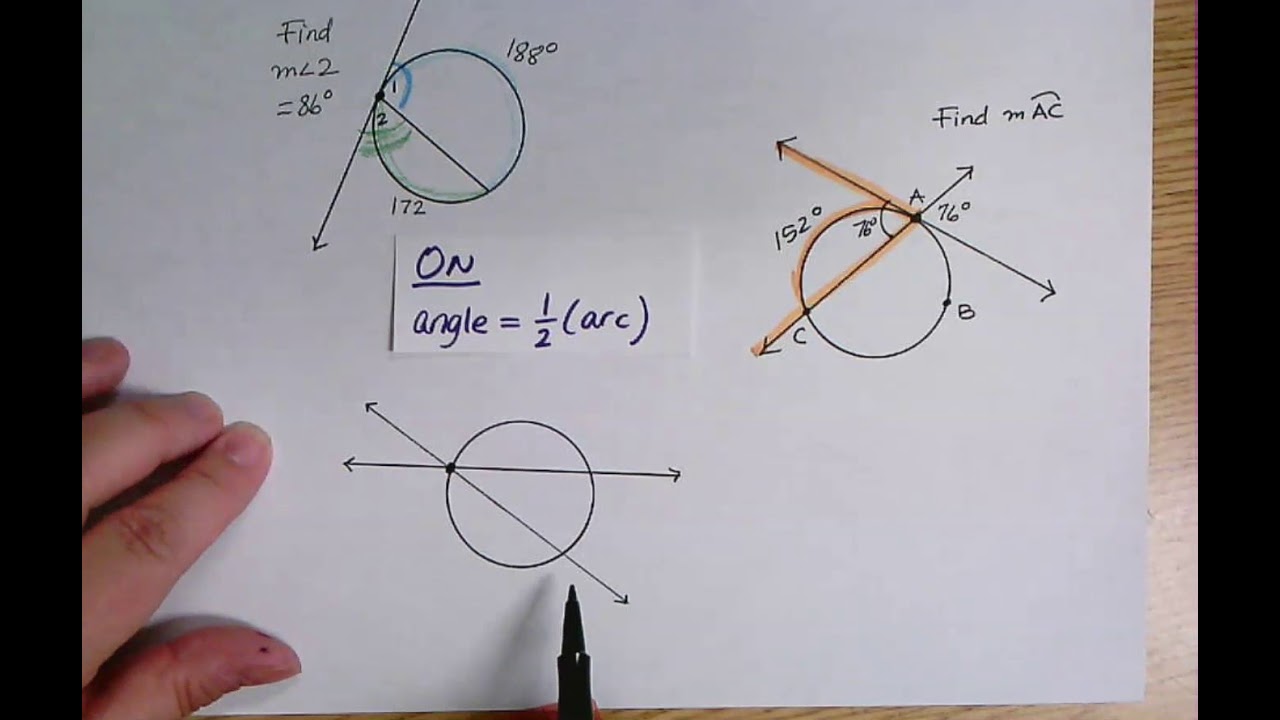
How is the measure of a central angle related to its intercepted arc?
-The measure of a central angle is equal to the measure of its intercepted arc.
What is an inscribed angle?
-An inscribed angle is an angle with its vertex on the circle and its sides containing the endpoints of a chord.
How do you calculate the measure of an inscribed angle?
-The measure of an inscribed angle is half the measure of its intercepted arc.
What is the significance of the vertex of a central angle?
-The vertex of a central angle is located at the center of the circle.
What is the relationship between the measure of a semicircle and the angles formed by it?
-The measure of a semicircle is 180 degrees, and any angle inscribed in a semicircle will also measure 90 degrees.
How can you find the measure of an angle when given the measure of an arc?
-To find the inscribed angle related to an arc, you can take half the measure of the arc. For a central angle, the measure is the same as the intercepted arc.
What is the formula to find the measure of a remaining arc if one arc's measure is known?
-The measure of a remaining arc can be found by subtracting the known arc's measure from 360 degrees.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео
5.0 / 5 (0 votes)