This Downward Pointing Triangle Means Grad Div and Curl in Vector Calculus (Nabla / Del) by Parth G
Summary
TLDRThis video introduces important differential operators—gradient, divergence, and curl—commonly used in physics and vector calculus. It explains how the 'nabla' operator is applied to scalar and vector fields, demonstrating how these operators measure changes in different directions. Through clear examples, such as flour distribution and fluid flow, the video delves into the concepts of gradient (change in a scalar field), divergence (outflow or inflow of a vector field), and curl (rotation in a vector field). The speaker also links these concepts to Maxwell's equations in electromagnetism.
Takeaways
- 🔺 Nabla (or del) is a vector used to represent differential operators like gradient, divergence, and curl.
- 🔄 Gradient measures how quickly a scalar field changes in space and results in a vector field.
- 📏 Partial derivatives (denoted by ∂) help isolate the change in a specific direction while keeping others constant.
- 🌍 Scalar fields represent quantities like altitude or temperature, and the gradient of these fields indicates the direction of the steepest ascent.
- 🌬️ Vector fields can represent physical phenomena like wind or electric fields, assigning a vector to each point in space.
- ⚡ Divergence measures how much a vector field spreads out from a point and can indicate sources or sinks in fields like electric fields.
- 💥 In electromagnetism, the divergence of an electric field relates to the presence of electric charges, and divergence of magnetic fields is always zero (no magnetic monopoles).
- 🔁 Curl measures the rotation or circulation of a vector field, such as the rotation of fluid flow, and results in another vector field.
- 📐 In physics, the curl operator is used in Maxwell’s equations, showing how changing magnetic fields induce electric fields.
- 🎓 Gradient, divergence, and curl are essential tools in vector calculus, with applications in physics like gravity, electromagnetism, and fluid dynamics.
Q & A
What are the gradient, divergence, and curl operators, and where are they commonly used?
-The gradient, divergence, and curl operators (often called grad, div, and curl) are differential operators used in vector calculus. They are widely used in physics, particularly in areas like fluid dynamics, electromagnetism, and gravitational fields. The gradient measures the rate of change of a scalar field, the divergence measures how much a vector field spreads out from a point, and the curl measures the rotation or circulation of a vector field.
What is the del (nabla) operator, and how does it relate to partial derivatives?
-The del (nabla) operator, denoted by a downward-pointing triangle, acts like a vector of partial derivatives in three dimensions. Its components are partial derivatives with respect to x, y, and z. It measures how quickly a quantity changes over small distances in different directions.
How does the gradient operator work, and what does it represent?
-The gradient operator, when applied to a scalar field, gives a vector field that represents the direction and rate of fastest change of the scalar field. For example, in a topographical map, the gradient would indicate the direction of the steepest incline at each point.
What is the physical interpretation of the divergence of a vector field?
-The divergence of a vector field gives a scalar value that represents how much the field is spreading out or converging at a point. For instance, in fluid flow, a positive divergence would indicate that fluid is flowing out from a point (a source), while a negative divergence indicates fluid flowing into a point (a sink).
How does the curl operator work, and what does it measure?
-The curl operator, when applied to a vector field, produces another vector field that represents the rotation or circulation of the original vector field. The direction of the resulting vector indicates the axis of rotation, while the magnitude represents the strength of the rotation.
Can you explain the physical significance of scalar and vector fields?
-A scalar field assigns a single value to each point in space (e.g., temperature distribution), while a vector field assigns a vector to each point (e.g., wind speed and direction). Scalar fields measure quantities like height or temperature, while vector fields measure directional phenomena like electric fields or fluid flow.
How is the gradient of a scalar field applied in physics, particularly with gravitational fields?
-In physics, the gradient of a scalar field, such as the gravitational potential, gives a vector field representing the gravitational force. The direction of the gradient indicates the direction in which the gravitational force acts, while its magnitude shows the strength of the force.
What role does the dot product play in calculating divergence?
-The dot product in the context of divergence applies the del operator to a vector field. It combines the partial derivatives from the del operator with the components of the vector field, resulting in a scalar field that measures how much the field is expanding or contracting at a point.
How are Maxwell's equations related to the divergence and curl operators?
-Maxwell's equations, which describe electric and magnetic fields, make extensive use of divergence and curl. For example, one of the equations states that the divergence of the magnetic field is always zero, implying no magnetic monopoles exist. Another equation involves the curl of the electric field, which is related to the changing magnetic field over time.
What is the difference between applying the gradient, divergence, and curl to fields in vector calculus?
-The gradient is applied to a scalar field and results in a vector field, representing the direction of steepest change. The divergence is applied to a vector field and results in a scalar field, representing how much the field spreads out from a point. The curl is applied to a vector field and results in another vector field, representing the rotation or circulation of the original field.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео

M172 - Operator Diferensial Vektor : Del atau Nabla,memahami secara mudah
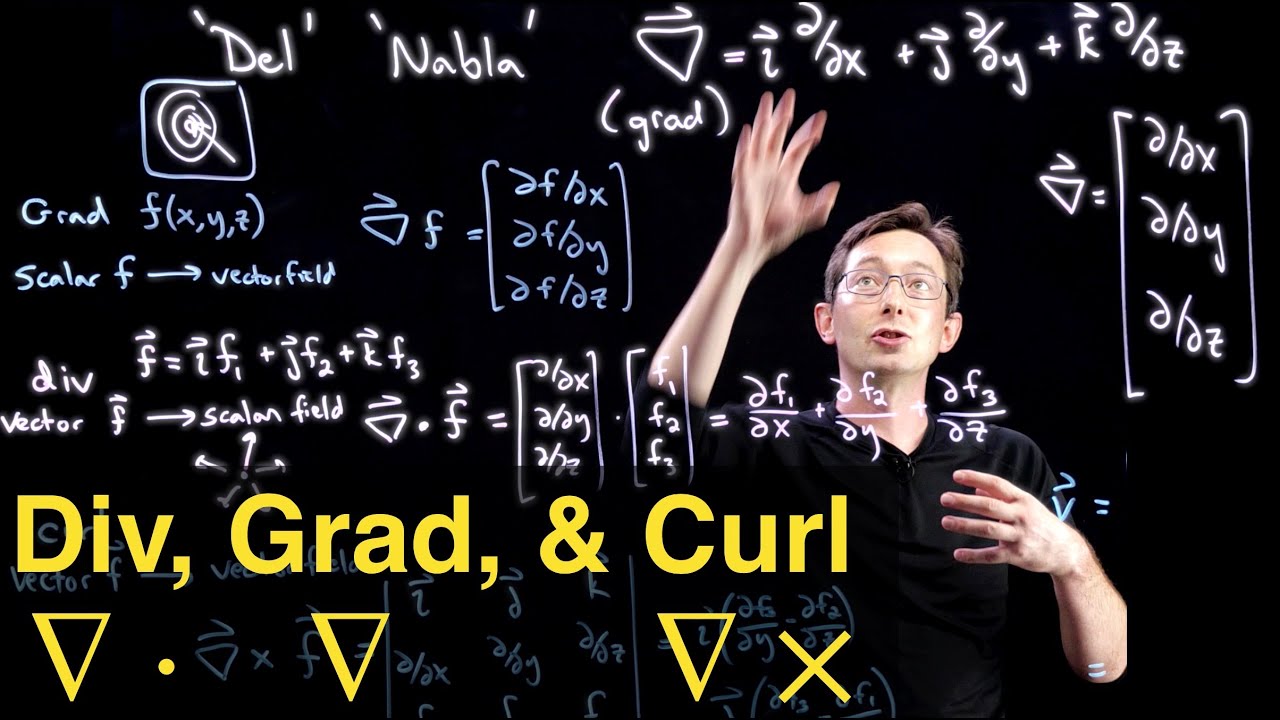
Div, Grad, and Curl: Vector Calculus Building Blocks for PDEs [Divergence, Gradient, and Curl]

The Curl of a Vector Field: Measuring Rotation

Divergence and Curl of vector field | Irrotational & Solenoidal vector
Concept of Vector Point Function & Vector Differentiation By GP Sir

Vector Calculus Module 4 Core Ideas
5.0 / 5 (0 votes)
