How to Divide Polynomials Using LONG DIVISION | Math 10
Summary
TLDRThis educational YouTube video teaches viewers how to divide polynomial expressions using long division. The instructor begins by defining a polynomial expression and explaining how to write it in standard form with terms ordered by decreasing powers of x. The video then outlines the steps for long division of polynomials, including arranging terms, dividing the leading term, multiplying the quotient by the divisor, subtracting the product from the dividend, and repeating until a remainder is reached or zero remainder is achieved. Examples are provided to illustrate the process, demonstrating how to handle remainders and ensuring the quotient is in the correct form.
Takeaways
- 📘 A polynomial expression is defined as an algebraic expression with non-negative integer exponents and real number coefficients.
- 📐 Polynomials are written in standard form with terms ordered by decreasing powers of the variable, like ax^n + ... + a_1x + a_0.
- ✅ The process of dividing polynomials involves arranging terms in decreasing order of indices and rewriting missing terms with zero coefficients.
- 🔢 The division of the first term of the dividend by the first term of the divisor initiates the long division process.
- 📝 Multiplying each term of the quotient by the divisor and subtracting the product from the dividend is a key step in polynomial long division.
- 🔄 The process is repeated with the new dividend until the remainder is obtained, which should have a lower index than the divisor.
- 📉 If the remainder is zero, the division is complete, and the quotient is the final result.
- 📊 Examples are used to demonstrate the division process, such as dividing 2x^2 + 5x - 23 by x + 5 and 6x^2 - 2x - 28 by 2x + 4.
- 🎯 The final quotient is expressed in the form of coefficient plus or minus remainder over the divisor, highlighting the importance of proper notation.
- 👍 The video encourages viewers to like, share, and subscribe for more educational content.
Q & A
What is a polynomial expression?
-A polynomial expression is an expression of the form \( a_nx^n + a_{n-1}x^{n-1} + \ldots + a_1x + a_0 \), where \( n \) is a non-negative integer, and the coefficients \( a_0, a_1, \ldots, a_n \) are real numbers.
How do you write a polynomial expression in standard form?
-To write a polynomial expression in standard form, you need to arrange the terms in decreasing order of the powers of \( x \).
What is the first step in dividing polynomial expressions using long division?
-The first step in dividing polynomial expressions using long division is to arrange the terms in decreasing order of their indices, ensuring the polynomial is in standard form.
What are the roles of the dividend and divisor in polynomial long division?
-In polynomial long division, the dividend is the polynomial being divided, and the divisor is the polynomial by which the dividend is divided.
How do you find the first term of the quotient when dividing polynomials?
-To find the first term of the quotient, divide the first term of the dividend by the first term of the divisor.
What is the purpose of multiplying the quotient by the divisor in the long division process?
-Multiplying the quotient by the divisor helps in obtaining the product, which is then subtracted from the dividend to form a new dividend for further division.
Why is it necessary to bring down the next term after subtraction in polynomial long division?
-Bringing down the next term after subtraction is necessary to continue the division process with the new dividend formed by the difference and the brought-down term.
What should be the condition of the remainder in polynomial long division?
-The remainder in polynomial long division should be of a lower degree than the divisor.
How do you express the final result of a polynomial division with a remainder?
-The final result of a polynomial division with a remainder is expressed as the quotient plus the remainder over the divisor.
What does it mean if the remainder is zero after dividing two polynomials?
-If the remainder is zero after dividing two polynomials, it means the divisor divides the dividend exactly, and the result is fully expressed by the quotient without any remainder.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео
Synthetic Division of Polynomials
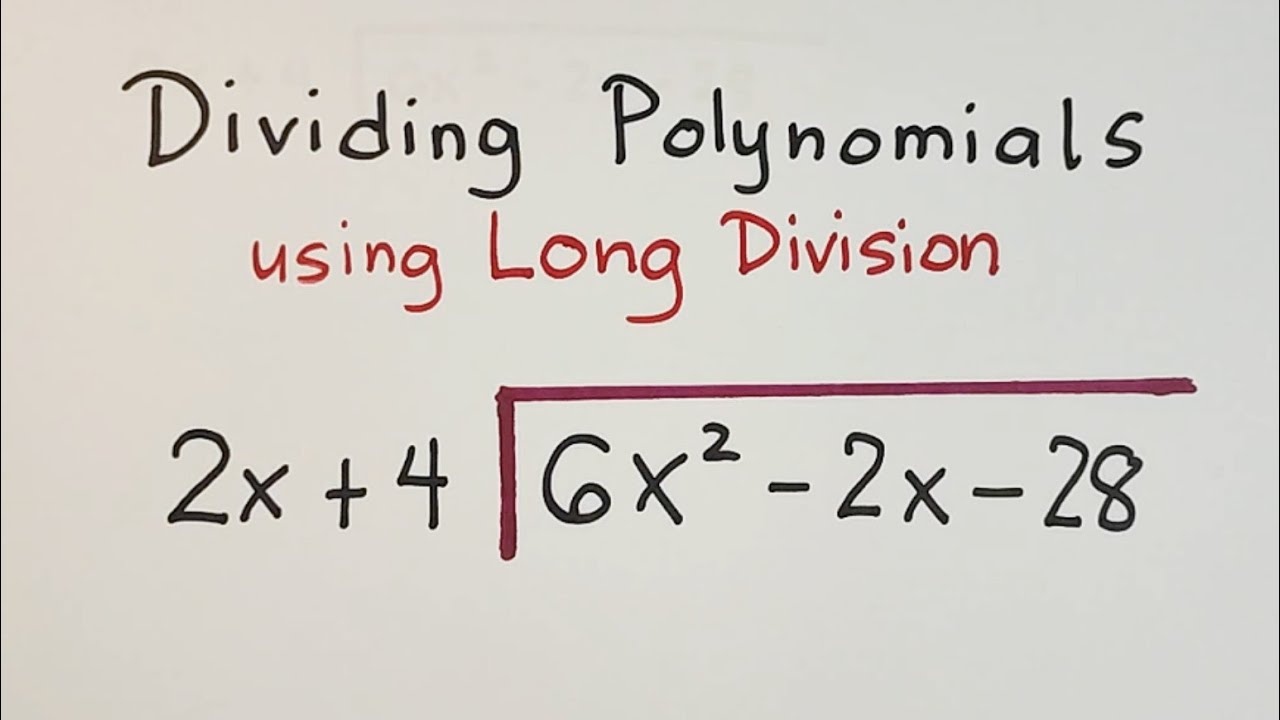
How to Divide Polynomials using Long Division - Polynomials

Lesson 33 Long Division to Find Roots
Polynomials - Adding, Subtracting, Multiplying and Dividing Algebraic Expressions

Fácil e Rápido | APRENDA DIVISÃO EM 6 MINUTOS

POLINÔMIOS #02 | ADIÇÃO E SUBTRAÇÃO COM POLINÔMIOS | \Prof. Gis/
5.0 / 5 (0 votes)
